ELCTROSTATICS QUESTIONS AND ANSWERS
Share via Whatsapp Join our WhatsApp Group Join our Telegram GroupWorked example 1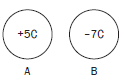
Two spheres A and B carry charges of +5 C and −7 C respectively. They are brought into contact and are then separated.
- What is the nature of the force between the charges before they are allowed to touch? Explain.
- In which direction are electrons transferred during the contact? Explain.
- Calculate the total charge in the system.
- Calculate the charge on each sphere when they are separated.
- Calculate the change in the charge on A and on B.
- Calculate the number of electrons transferred from one sphere to the other.
Solutions
1. Attraction. Opposite charges (+ and −) attract.
2. From sphere B to sphere A. Electrons are transferred from the sphere with the most electrons (B in this case) to the sphere with the least electrons (A).
3. Qtotal = Qi (A) + Qi (B) = +5 + (−7) = −2 C
4. Qnew on each = Qtotal = -2 = −1 C = Qf
2 2
5. ΔQA = Qf – Qi = −1 – (+5) = −6 C ∴ 6 C charge was transferred from B to A
ΔQB = Qf – Qi = −1 – (−7) = +6 C ∴ 6 C charge was transferred from B to A
6. No. of e– transferred ΔQ(A)= No. of e– transferred = ΔQ(B)
Qe- Qe-
= 6 or 6
1.6 × 10-10 1.6 × 10-19
= 3,75 × 1019 electrons
Worked example 2
The original force between two charges is F. If both charges are doubled and the distance is a third of the original distance, what is the magnitude of the new force relative to the original force?
Solution
Foriginal = kQ 1Q 2
r2
Fnew = k(2Q1)(2Q2)
(1/3r)2
Fnew = 4kQ 1Q 2 = 36kQ 1Q 2 = 36Foriginal
1/3r2 r2
Remember to square the distance.
Worked example 3
Two small identical metal spheres carry equal but opposite charges. If their centres are 30mm apart, and the electrostatic force between them is 2.56 × 10–3N. Calculate:
- The magnitude (size) of the charge on each sphere
- The number of electrons that would flow from the negatively charged sphere to the positively charged sphere if they were brought into contact.
Solutions
- F = kQ 1Q 2
r2
2,56 × 10–3 × 0,0009 (9 × 10–4) = 9 × 109 × Q × Q
2,304 × 10–6 / ( 9 × 109) = Q2
Q2 = √2,56 × 10–16 ; Q = 1,6 × 10–8C - number of electrons = total charge
charge on one electron
= (1,6 × 10–8) / (1,6 × 10–19)
= 1 × 1011 electrons
‘Charges are unknown’ this means I need to calculate them.
I must remember just not forget to convert mm to m.
Activity 1
Two small identical metal spheres, A and B carrying charge of –4 × 10–12C and –3 × 10–12C respectively, are mounted on insulated stands as shown.
The distance between the centres of the spheres is 5 cm.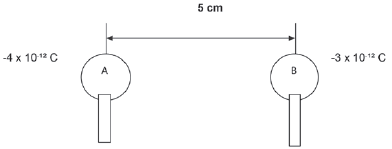
- Calculate the magnitude and direction of the force that A exerts on B. (6)
Sphere A is moved and makes contact with sphere B. It is then moved back to its original position. - Calculate the new charge on each of the spheres. (3)
- How does the magnitude of the force that the sphere A exerts on sphere B change? Answer by writing ONLY increases, decreases or remains the same. (2)
[11]
Solutions
|
Start by converting the distance 5cm to m. Look at the conversion table.
Worked example 4
Two point charges, Q1 and Q2, at a distance of 3 m apart, are shown below. The charge on Q1 is –14 μC and the charge on Q2 is +20 μC.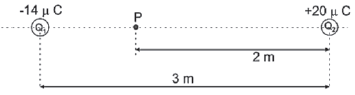
Remember:
First convert μC to C: –14 μC = –14 × 10–6 C and 20 μC = 20 × 10–6 C
- Define the electric field strength at a point.
- Calculate the net (resultant) electric field at point P situated 2 m from Q2.
Solutions
- Electric field strength at a point is the electric force per unit positive charge experienced at the point.
- Electric field at P due to Q1:
E = kQ = (9 × 109)(14 × 10–6)
r2 12
= 1,26 × 105 N·C–1 to the left
Electric field at P due to Q2:
E = kQ = (9 × 109)(20 × 10–6)
r2 12
= 4,5 × 104 N·C–1 to the left
Let ← E+
Enet = EQ1 + EQ2
= (+1,26 × 105) + (+4,5 × 104 N·C–1)
= +1,71 × 105 N·C–1
∴ 1,71 × 105 N·C–1 to the left
The values substituted are positive (+), because left is chosen as positive and both the electric fields are directed to the left.
Step by step
Step 1. ALWAYS calculate the electric field strength at the given point (P in this case) due to each of the point charges first. The negative sign for a negative charge is NOT used in this equation.
Step 2. Then choose an electric field direction as positive and state this clearly.
Step 3. Then find the resultant (or net) electric field strength by adding the two field strength values. Lastly, remember the signs for the directions!
Activity 2
Multiple Choice Questions:
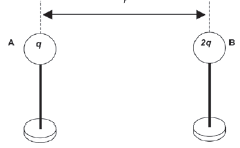
- The sketch below shows two small metal spheres, A and B, on insulated stands carrying charges of magnitude q and 2q respectively.
The distance between the centres of the two spheres is r.
Sphere A exerts a force of magnitude F on sphere B. What is the magnitude of the force that sphere B exerts on sphere A?- ½ F
- F
- 2F
- 4 (2)
- Two identical small metal spheres on insulated stands carry equal charges and are a distance d apart. Each sphere experiences an electrostatic force of magnitude F.
The spheres are now placed a distance ½ d apart.
The magnitude of the electrostatic force each spheres now experience is..- ½ F
- F½
- 2½ F
- 4F (2)
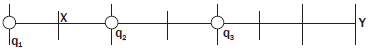
- Three identical point charges, q1, q2 and q3, are placed in a straight line, as shown below. Point charge q2 is placed midway between point charges q1 and q3. X and Y are two points on the straight line as shown.

Which ONE of the following best describes how the electric field E at a point X compares to that at point Y?
| DIRECTION OF E | MAGNITUDE OF E |
| A. Same | EX > EY |
| B. Same | EX < EY |
| C. Opposite | EX > EY |
| D. Opposite | EX < EY |
[6]
| Solutions 1. B (2) 2. D (2) 3. D (2) [6] |
Activity 3
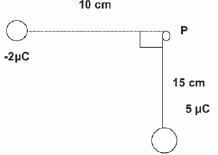
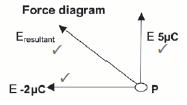
A negative charge of 2 μC is positioned 10 cm from point P, as shown below.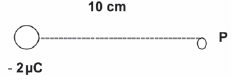
- Define the electric field at point P in words. (2)
- Draw the electric field lines associated with this charge. (2)
- A positive charge of 5 μC is now positioned 15 cm from point P, as showed in the diagram below.
Calculate the magnitude of the electric field at point P due to both charges. (12)
[16]
Solutions
| ||||||
Activity 4
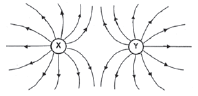
The centres of two small, charged conducting spheres, X and Y, on insulated stands, are separated by a distance of 60 mm. Sphere X initially carries a charge of +12 × 10–9 C.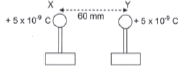
X and Y are brought into contact with each other and are separated again.
After separation, each sphere carries EQUAL charges of +5 × 10–9 C.
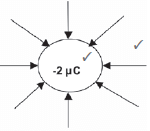
- Draw a neat diagram of the resultant electric field pattern that surrounds X and Y. (4)
- Calculate the number of electrons that must be added to Y to make it neutral. (3)
- Calculate the magnitude of the force which X exerts on Y once they are back in their original positions (after separation). (4)
- Calculate the original charge on sphere Y. (4)
[15]
Solutions
|