CHEMICAL EQUILIBRIUM GRADE 12 NOTES - PHYSICAL SCIENCE PAPER 2: CHEMISTRY STUDY GUIDES
Share via Whatsapp Join our WhatsApp Group Join our Telegram Group- Summary
- Key concepts
- Factors that influence chemical equilibrium position
- Le Châtelier’s Principle
- The Equilibrium Constant (Kc) (The Law of Mass Action)
- Interpretation of graphs for chemical systems in dynamic equilibrium
- Applications of equilibrium principles in the chemical industry
Chemical equilibrium
When a chemical reaction takes place, the atoms or ions in the reactants form new bonds and new products with different chemical formulas and different chemical properties.
3.1 Summary
3.2 Key concepts
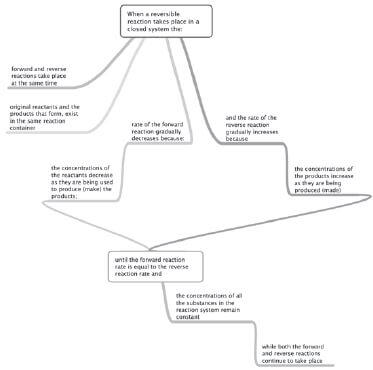
In this section, we will be revising the basic concepts involved in chemical equilibria (plural of equilibrium). In a chemical reaction, chemical equilibrium is the state in which both reactants and products are present in concentrations which have no further tendency to change with time. Usually, this state results when the forward reaction proceeds (continues) at the same rate as the reverse reaction. NB. This does not mean the reaction has stopped; instead it means that it is continuing to react, but the reactants and products are being produced (and re-produced) at a constant rate.
HOMOGENEOUS REACTIONS
are reactions where all the reactants and products are in the same phase,
i.e. all are solids, or all are liquids, or all are gases
SO2(g) + O2(g) ⇌ SO3(g)
HETEROGENEOUS REACTIONS
are reactions where not all species in the reaction vessel are in the same phase.
CaCO3(s) + HCℓ(aq) → CaCℓ2(aq) + CO2(g) + H2O(ℓ)
Most chemical reactions are non-reversible or irreversible – this means that the products cannot change back into the reactants.
These reactions are essentially like baking. The ingredients, acting as the reactants, are mixed and baked together to form a cake, which acts as the product. This cake cannot be converted back to the reactants (the eggs, flour, etc.), just as the products in an irreversible reaction cannot convert back into the reactants (without e.g. human intervention). An example of an irreversible reaction is combustion. When carbon burns in oxygen to form carbon dioxide, the reaction is said to be non-reversible.
C(s) + O2(g) → CO2(g)
Pay special attention
- A non-reversible or irreversible reaction has a single arrow, indicating the products cannot form reactants during the chemical reaction. Combustion, for example, involves burning an organic compound—such as a hydrocarbon, in oxygen, to produce carbon dioxide and water. Because water and carbon dioxide are stable, they do not react with each other to form further reactants unless acted on by a strong external influence such as a lightning strike, an electrolytic cell set up by a human, etc.
Some chemical reactions are reversible. The products react with each other to form the original reactants under suitable conditions. (E.g. change in pressure, temperature, reactant concentration, etc.).
N2(g) + 3 H2(g) ⇋ 2 NH3(g)
hint
- A reversible reaction has a double arrow
Therefore:
Forward reaction | Reverse reaction | |
N2(g) + 3 H2(g) → 2 NH3(g) | Reaction | 2 NH3(g) → N2(g) + 3 H2(g) |
N2(g) + 3 H2(g) | Reactants | 2 NH3(g) |
2 NH3(g) | Products | N2(g) + 3 H2(g) |
NB: DEFINITIONS
- Open chemical system: reactants / products can escape from reaction vessel
- Closed chemical system: reactants / products cannot escape from reaction vessel
- Macroscopic changes: measurable or visible changes e.g. changes in colour, temperature, pressure, volume, concentration
- Yield: the amount of product formed during a chemical reaction
- An open system continuously interacts with its environment (conditions are not kept constant).
- A closed system is isolated from its surroundings (conditions are kept constant)
- A reversible reaction occurs when the products can be converted back to reactants
- Chemical equilibrium is dynamic when the rate of the forward reaction equals the rate of the reverse reaction
- The concentration of a solution or of a gas is the number of moles of substance per unit volume (per dm3).
- A dynamic chemical equilibrium is established in a closed system when the forward and reverse reactions of a reversible chemical reaction take place at the same time (simultaneously) and at the same rate. The conditions in the system must also remain constant.
c = n where n = m or C = m
v M Mv
hint: You need to know the formula, unit and symbols used to calculate the concentration of a solution
- c : concentration (mol·dm–3)
- V : volume (dm3)
- M : Molar mass (g·mol–1)
- n : number of moles (mol)
- m : mass (g)
You need to understand what a chemical equilibrium is and know the conditions needed for a chemical equilibrium.
Chemical equilibrium
- When a chemical system reaches dynamic chemical equilibrium, both the forward and the reverse reactions continue – they do NOT stop.
There are no macroscopic (visible with the eye) changes when the system is in equilibrium, but on a microscopic (atomic) level, the changes (reactions) continue.
Neither the reactants nor the products are ever used up in an equilibrium system (incomplete reaction – i.e. “incomplete” in the sense that there are still species of the original/starting species (reactants.))
Activity 1
Write the name of a reaction in which all reactants and products are in the same phase (1) [1]
Solution
- Homogeneous ✓ [1]
3.3 Factors that influence chemical equilibrium position
- Temperature (T): Increasing or decreasing the temperature of the equilibrium mixture
- Concentration (c): Adding a substance to or removing a substance from the system at constant volume. This only applies to solutions (aq) and to gases (g).
- Pressure (p): Increasing or decreasing the pressure on an equilibrium mixture (this ONLY applies to gases).
3.4 Le Châtelier’s Principle
If a closed system is in a state of dynamic chemical equilibrium, and one of the factors that influences the equilibrium (temperature, concentration or pressure) is changed:
- the equilibrium position will be disturbed;
- either the forward or the reverse reaction will be favoured;
- the applied change will be opposed;
- the reaction rates will change and eventually
- a new equilibrium will be established
- so that the forward and the reverse reactions again take place at the same rate.
NB: Le Châtelier’s Principle states that:
- When the equilibrium in a closed system is disturbed, the system will re-instate a new equilibrium by favouring the reaction that will oppose the disturbance.
3.4.1 Applying Le Châtelier’s Principle to changes in equilibrium conditions
Hint: If any factor changes, we can apply Le Châtelier’s Principle to determine which changes will happen to the system in equilibrium (what upsets the balance).
| Changing the temperature of the reaction mixture (ΔT) | |
Increasing the temperature of the reaction system (i.e. heating it)
| Decreasing the temperature of the reaction system (i.e. cooling it)
|
| Changing the concentration of a solution or gas reactant (∆c) | |
Increasing the concentrationof a (g) or (aq) reactant (or product)
| Decreasing the concentration of a (g) or (aq) reactant (or product)
|
| Changing the pressure of a gaseous system (∆p) | |
Increasing the pressure (or decreasing the volume) of a gaseous system
| Decreasing the pressure (or increasing the volume) of a gaseous system
|
Steps to follow
Follow these steps to predict the change in a closed chemical system in dynamic equilibrium:
Check that the reaction:
- Step 1: Is reversible (⇌) (info given must indicate it is a dynamic equilibrium).
- Step 2: Equation is balanced.
- Step 3: Count the number of gaseous (g) moles on each side of the ⇌ .
Determine:
- Step 4: If the forward reaction is exothermic or endothermic
- Step 5: If the reverse reaction is exothermic or endothermic
- Step 6: The change (which factor?) disturbs the equilibrium
Decide:
- Step 7: According to Le Châtelier’s Principle, which reaction is favoured (forward or reverse)? (Follow the reasoning in the table above.)
- Step 8: How the concentrations of the reactants and products change.
hint
- By closed we do not mean a closed container. Rather we mean a closed system where the conditions are kept constant.
BX2Y2 is a solid. Solids in equilibrium reactions have very little change in mass, hence they are ignored
Activity 2
Consider the following reversible reaction that is in equilibrium in a closed system (the symbols represent chemicals which are unnamed; if you see symbols like X or Y which aren’t on the Periodic Table, it often means you’ve got to work out what kinds of substances they are).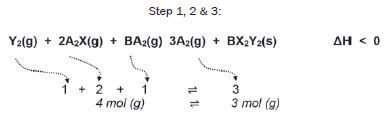
Step 4/5:
∆H < 0
- the forward reaction is exothermic
- the reverse reaction is endothermic
In each of the following cases:
- Explain how the equilibrium will be affected (which reaction will be favoured)
- State how the concentration of the product A2(g) will change.
CASE 1 The reaction system is heated. (3) [3]
Solution
Case 1.
If the reaction system is heated, the temperature of the system increases.
According to Le Châtelier’s Principle, increasing the temperature of the reaction system (i.e. heating it) favours the reaction that will decrease the temperature of the system
- ∴favours the endothermic ✓ reaction but ∆H < 0
- ∴the reverse reaction is endothermic
- ∴the reverse reaction is favoured✓∴the 3A2(g) + BX2Y2(s) reacts faster than it is produced
- ∴the concentration of the A2(g) decreases. ✓
(Remember: The opposite is true if the reaction vessel is cooled.) [3]
hint
You must be able to use Le Châtelier’s Principle to identify the factor that will increase the yield of the products and what influence that factor will have on the reaction AT EQUILIBRIUM
CASE 2 Y2(g) is added to the vessel at constant pressure and temperature (3) [3]
Solution
Case 2.
Adding Y2(g) increases the concentration of the Y2(g). ✓
According to Le Châtelier’s Principle, increasing the concentrationof Y2(g) favours the reaction that decreases the concentration of Y2(g)
- ∴the reaction that uses of Y2(g) as a reactant
- the forward reaction is favoured. ✓
- ∴the rate at which A2(g) and BX2Y2(s) is produced, increases
- the concentration of the A2(g) increases. ✓ [3]
CASE 3 A2(g) is removed from the vessel at constant pressure and temperature. (3) [3]
Solution
Case 3.
Removing A2(g) decreases the concentration of the A2(g). ✓
According to Le Châtelier’s Principle, decreasing theconcentration of the A2(g) favours the reaction that increases the concentration of the A2(g);
- the reaction that makes A2(g) as a product
∴ the forward reaction is favoured. ✓
the rate at which A2(g) and BX2Y2(s) is produced, increases
the concentration of the A2(g) increases. ✓ [3]
CASE 4 Some of the BX2Y2(s) is removed from the system at constant pressure and temperature. (3) [3]
Solution
Case 4.
Removing BX2Y2(s) has no effect on the equilibrium as it is a solid. ✓
Removing a solid from an equilibrium system does not disturb the concentration. ✓ (Solids in equilibrium reactions have very little change in mass, hence they are ignored)
The concentration of A2(g) remain the same. ✓ [3]
CASE 5 The pressure on the system is increased (or the volume is decreased) (3) [3]
Solution
Case 5.
According to Le Châtelier’s Principle, increasing the pressure (or decreasing the volume) of a gaseous system favours the reaction that decreases the pressure of the system by decreasing the total number of gaseous moles in the system. ✓
- the forward reaction is favoured. ✓
- the rate at which A2(g) and BX2Y2(s) is produced, increases
- the concentration of the A2(g) increases. ✓ [3]
hint
There are 4 gaseous moles on the left, and 3 gaseous moles on the right.
Activity 3
Say whether the statement is TRUE or FALSE: If the equilibrium constant for the reaction A2(g) + B2(g) ⇌ 2AB(g) is equal to K, then the equilibrium constant for the reverse reaction
2AB(g) ⇌ A2(g) + B2(g) is also equal to K. (3) [3]
Solution
FALSE✓✓ . ... for the reverse reaction 2AB(g) ⇌ A2(g) + B2(g) is equal to 1/K ✓✓ OR ... less (smaller) than K [3]
Activity 4
The industrial preparation of hydrogen gas is represented by the equation below:
CH4(g) + H2O(g) ⇌ CO(g) + 3H2(g) ∆H > 0
The reaction reaches equilibrium at 1 000 °C in a closed container.
- State Le Châtelier’s principle. (2)
- How will an increase in pressure at 1 000 °C (by decreasing the volume) affect the yield of hydrogen gas? Write down only INCREASES, DECREASES OR NO EFFECT. Explain the answer. (2)
- Give TWO reasons why high temperatures are used for this reaction. (2) [6]
hint
There are various ways to state Le Châtelier’s Principle correctly.
Solution
- When the equilibrium in a closed system is disturbed the system will shift the equilibrium position (OR re-instate a new equilibrium)✓ as to favour the reaction that will oppose OR cancel OR counteract the change OR disturbance. ✓
OR
When a stress / change is placed on a system in equilibrium the system shifts the equilibrium ( position) OR re-instate a new equilibrium ✓ so as to remove OR cancel OR oppose the stress / change. ✓ (2)
OR
When the conditions affecting an equilibrium are changed, the equilibrium (position) shifts in such a way ✓ as to oppose the change OR cancel the change. ✓ (any two) - Decreases ✓ When the pressure is increased, the reverse reaction is favoured.✓
OR
The reaction that produced the smaller volume/amount of gas is favoured. ✓
OR
4 mol or volumes of gas produces ✓ 2 mol or volumes of gas. ✓ (2) (any two) - Products form at faster rate. ✓
Higher yield of products. ✓ (2) [6]
hint
In physical chemistry, saturation is the point at which a solution of a substance can dissolve no more of that substance. Additional amounts of the solute will appear as a separate phase (usually as a precipitate solid). This point of maximum concentration, the saturation point, depends on the temperature and pressure of the solution as well as the chemical nature of the substances involved.
3.4.2 Dynamic chemical equilibrium in solutions
A dynamic chemical equilibrium in a solution:
- is only possible if the solution is saturated
- is only affected by changing the
- concentration of the ions in the solution or
- temperature of the solution.
The common ion effect
Steps to follow
When a solution is added to a reaction system:
- Step 1: Identify the common ion in the solution and the system
- Step 2: Explain the effect of the common ion (addition) on the chemical equilibrium.
| Solution added to the equilibrium mixture: | Effect on equilibrium mixture: H+(aq) + OH−(aq) ⇌ H2O(ℓ) |
| Hydrochloric acid, HCℓ(aq) |
|
Sulphuric acid, H2SO4(aq) |
because: |
| Concentrated sulphuric acid, H2SO4(ℓ) |
|
| A base like NaOH(aq) or KOH(aq) |
|
| A solution containing Cℓ(aq) ions e.g. NaCℓ(aq) |
|
e.g. Worked example 1
Consider the following equilibrium:
CoCℓ42–(aq) + 6H2O(ℓ) ⇋ Co(H2O)62+(aq) + 4Cℓ–(aq) ΔH < 0
blue purple-pink pink
When cobalt chloride crystals (CoCℓ2⋅6H2O) are dissolved in ethanol (the solvent) in a flask, a blue solution is formed with CoCℓ42– ions. By carefully adding water to the blue solution until it just turns purple-pink because of the formation of the pink Co(H2O)62+ ions, the above equilibrium is reached.
Five test tubes each contain a small amount of the above purple-pink equilibrium solution. Describe and explain the colour change observed in each of the following instances:
- The solution is cooled down.
Becomes pink.
According to Le Châtelier’s Principle, a decrease in temperature favours the exothermic, forward reaction therefore increasing the [Co(H2O)62+(aq)] which is pink. - Water is added to the solution.
Becomes pink.
According to Le Châtelier’s Principle, adding H2O will favour the production of more Co(H2O)62+(aq) ions so that the number of H2O molecules will decrease, therefore the forward reaction is favoured. The concentration of the Co(H2O)62+(aq) ions which are pink, increases. - A few NaCℓ crystals are added to the solution. exams
Becomes blue.
Adding NaCℓ to the equilibrium mixture, increases the [Cℓ–] as a result of the common ion (Cℓ–) found in the reaction mixture and in the NaCℓ. The reverse reaction which decreases the [Cℓ–(aq)], is favoured, thereby increasing the concentration of the CoCℓ42–(aq) ions which are blue. - Concentrated sulphuric acid is added to the solution.
Becomes blue.
Concentrated sulphuric acid (H2SO4) is a good dehydrating agent therefore it will extract water molecules (H2O) from the mixture. The reverse reaction that produces H2O molecules is favoured and at the same time increasing the concentration of the CoCℓ42–(aq) ions which are blue. - A few drops of silver nitrate solution are added to the solution.
Becomes pink.
When the silver nitrate solution is added to the mixture, the silver ions, Ag+(aq) react with the Cℓ–(aq) to form a AgCℓ(s) precipitate, thereby decreasing the [Cℓ–]. According to Le Châtelier’s Principle, the forward reaction that increases the [Cℓ–] ions is favoured. More pink Co(H2O)62+(aq) ions form.
Activity 5
1. The following hypothetical reaction reaches equilibrium in a closed container at a certain temperature:
X2(g) + Y2(g) ⇋ 2XY(g) ∆H < 0
Which ONE of the following changes will increase the AMOUNT of XY(g)?
- Decrease in temperature
- Increase in temperature
- Increase in pressure
- D. Decrease in pressure (2)
2. The equation below represents a chemical reaction at equilibrium in a closed container.
H2(g) + I2(g) ⇌ 2HI(g) ∆H < 0
Which ONE of the following changes will increase the yield of HI(g) in the above reaction?
- Increase the temperature
- Decrease the temperature
- Increase the pressure by decreasing the volume
- Decrease the pressure by increasing the volume (2)
3. A chemical reaction reaches equilibrium. Which ONE of the following statements regarding this equilibrium is TRUE?
- The concentrations of the individual reactants and products are constant.
- The concentrations of the individual reactants and products are equal.
- The concentrations of the individual reactants are zero.
- The concentrations of the individual products increase until the reaction stops. (2)
4. The reaction represented by the equation below reaches equilibrium.
2CrO24−(aq) + 2H+(aq) ⇌ Cr2O27−(aq) + H2O(ℓ)
yellow orange
Which ONE of the following changes to the reaction mixture will change its colour from yellow to orange?
- Add a catalyst.
- Add water to the reaction mixture.
- Add a few drops of sodium hydroxide solution to the reaction mixture.
- Add a few drops of concentrated hydrochloric acid to the reaction mixture. (2)
5. Give one word for the following phrase:
The stage reached in a reversible chemical reaction when the rate of the forward reaction is equal to the rate of the reverse reaction. (2) [10]
Solution
1. A ✓✓ (2)
2. B✓✓ (2)
3. A ✓✓(2)
4. D ✓✓ (2)
5. (Dynamic/Chemical) equilibrium ✓✓ (2) [10]
NB
Solids and pure liquids are omitted from the Kc expression as their concentration is [1], as multiplying by 1 has no effect.
3.5 The Equilibrium Constant (Kc) (The Law of Mass Action)
The concentrations of all the compounds (solutions and gases) in a closed system in dynamic chemical equilibrium are related by a mathematical equation. The numerical value of this equation is called the equilibrium constant (Kc).
In the hypothetical equation below the equilibrium expression for this reaction is:
2P+3R⇋2S+4T
Kc = [S]2 × [T]4
[P]2 × [R]3
hint
Kc: equilibrium constant (no unit)
[substance]: concentration of reactant or product (in mol·dm–3) mol: number of moles of each compound in the balanced reaction equation.
NB
- This is the correct way of writing the Kc expression
- The coefficients are the moles of each reactant and product in the balanced equation
- The product of the concentration of reactants (not to be added, but multiplied!!), raised to the power of the number of moles is the numerator
- The product of the concentration of products (not to be added, but multiplied!!), raised to the power of the number of moles is the denominator
- The concentrations used in the Law of Mass Action is the [reactant]equilibrium and [product]equilibrium (NOT initial concentrations!! i.e. the concentrations of reactants and products at equilibrium)
Activity 6
Write Kc expressions for each of the following reactions:
- N2(g) + 3H2(g) ⇋ 2NH3(g) (1)
- CaCO3(s) ⇋ CaO(s) + CO2(g) (1)
- P4(s) + 6Cℓ2(g) ⇋ 4PCℓ3(ℓ) (1) [3]
Solution
- Kc = [NH3]3
[N2]2.[H2]3 - Kc = [CO2]
- Kc = 1
[CL2]6
3.5.1 Factors that influence the equilibrium constant
For a closed system in equilibrium:
- Only the concentrations of aqueous solutions (aq) and gases (g) appear in the Kc expression;
- Solids (s) and pure liquids (ℓ) are NOT included in the Kc expression.
- The value of Kc DOES change if the temperature of the system changes.
The value of Kc DOES NOT change if:
- the pressure in the system changes;
- the concentration of a reactant or product in the system changes;
- a catalyst is added to the system.
hint You need to know the:
- conditions and
- factors
that play a role in HOW/WHEN Kc changes/does NOT change
The effect of a change in temperature on Kc
Kc for a specific reaction is constant at a specific temperature.
| If the change in the temperature of a closed equilibrium system: | |
| favours the forward reaction: | favours the reverse reaction: |
|
|
3.5.2 The meaning of Kc-values
The significance of the Kc value is important in industrial processes. The economic viability of an industrial process in the chemical industry depends on the manufacturing costs, the product yield (amount of product produced), and the retail cost. These factors determine the profit a company would make.
Kc values are used to determine if the possible yield at a specific temperature is low or high.
- If Kc is small (Kc < 1) the:
equilibrium concentrations of the products are relatively low compared to those of the reactants product yield is low industrial process is not economically viable. - If Kc is large (Kc > 1) the:
equilibrium concentrations of the products are relatively high compared to those of the reactants product yield is high industrial process may be economically viable.
NB: You must understand the meaning of Kc. The value of Kc is directly linked to the yield of the reaction.
3.5.3 Calculating the value of Kc for a closed system in dynamic equilibrium|
There are THREE types of calculations that you may be asked to perform. They will be covered in the following pages.
Type 1: The initial amount (mol) of each reactant is given
e.g. Worked example 2
Consider the following balanced reaction for the formation of sulphur trioxide, in the second step of the Contact Process:
2SO2(g) + O2(g) ⇋ 2SO3(g)
Exactly 8 mol SO2 and 10 mol O2 are sealed in a 500 cm3 reaction vessel which is initially empty. The compounds react to produce SO3. At equilibrium, the vessel contains 6 mol SO3.
1.1 Calculate the equilibrium concentrations of each of the compounds in the reaction vessel.
Solution
The Contact
Process is a three step process in the manufacturing of sulphuric acid.
Step 1:
Always start by drawing a table with a column for the descriptions and a column for each of the reactants and products. Fill in the formulas of the reactants and products and the number of moles of each in the balanced reaction equation in the top line. The table should always consist of 5 rows with names as indicated: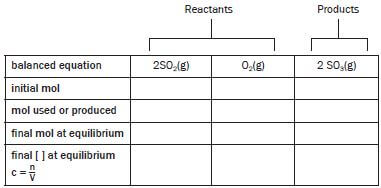
Step 2:
Now fill in all the values given in the question. If only reactants were added at the beginning of the reaction, the initial mol of the products is zero.
balanced equation | 2SO2(g) | O2(g) | 2SO3(g) |
initial mol | 8 | 10 | 0 |
mol used / produced | |||
final mol at equilibrium | 6 | ||
final [ ] at equilibrium | c = n = n | c = n = n | c = n = n |
Step 3:
Find the column with 2 values. Complete the mol used or produced line for this compound.
balanced equation | 2SO2(g) | O2(g) | 2SO3(g) |
initial mol | 8 | 10 | 0 |
mol used / produced | 6 | ||
final mol at equilibrium | 6 | ||
final [ ] at equilibrium | c = n = n | c = n = n | c = n = n |
Step 4:
Compare the ratio of moles according to which the reactants react and to which the products are produced, according to the balanced reaction equation: SO2 : O2 : SO3 = 2 : 1 : 2
Consider the actual mol used or produced for the compound which is known.
We have just calculated that 6 mol SO3 has been produced.
This means that the ratio of SO2 : O2 : SO3 = 2 : 1 : 2 (balanced equation) 6 :3:6
Fill in these values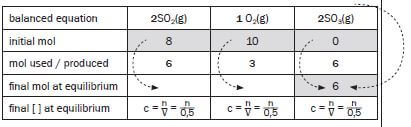
Step 5:
For the reactants: initial mol – mol used = final mol at equilibrium.
For the products: initial mol + mol produced = final mol at equilibrium.
balanced equation | 2SO2(g) | O2(g) | 2SO3(g) |
initial mol | 8 | 10 | 0 |
mol used / produced | 6 | 3 | 6 |
final mol at equilibrium | 8 - 6 = 2 | 10 - 3 = 7 | 6 |
final [ ] at equilibrium | c = n = n | c = n = n | c = n = 6 = 12 |
Step 6:
Substitute the final mol values in the last line, and calculate the final concentrations.
These are the answers to Question 1.1 above.
balanced equation | 2SO2(g) | O2(g) | 2SO3(g) |
initial mol | 8 | 10 | 0 |
mol used / produced | 6 | 3 | 6 |
final mol at equilibrium | 2 | 7 | 6 |
final [ ] at equilibrium | 2 = 4 | 7 = 14 | 12 |
e.g. Worked example 3
1.2 Write an expression for the equilibrium constant for the forward reaction and calculate Kc for the forward reaction at this temperature.
Solution
1. Kc = [SO3]2 = 122 = 0,64
[SO2]2[O2] 42.14
Type 2: The initial amount (mol) of one reactant and the concentration of a reactant or product are given
e.g. Worked example 4
2 mol of NO2(g) and an unknown amount of N2O4(g) are sealed in a 2 dm3 container, that is fitted with a plunger, at a certain temperature. The following reaction takes place:
2NO2(g) ⇋ N2O4(g)
At equilibrium it is found that the NO2 concentration is 0,4 mol·dm–3. The equilibrium constant (Kc) at this temperature is 2.
1. Calculate the initial amount (in mol) of N2O4(g) that was sealed in the container.
Solution
Step 1:
Draw the table as before. Fill in the formulas of the reactants and products and the number of moles of each in the balanced reaction equation in the top line.
Now fill in all the values given in the question. If only reactants were added at the beginning of the reaction, the initial mol of the products is zero. When the initial amount (mol) of a reactant or product is NOT zero and the value is not given (in this case the amount of N2 O4) is not given, let this amount equal x. Write the statement regarding ‘x’ down, “Let …. be x”.
Let the initial amount of N2O4 in the reaction vessel be x.
balanced equation | 2 NO2 | N2O4 |
initial mol | 2 | |
mol used / produced | ||
final mol at equilibrium | ||
final [ ] at equilibrium | 0,4 |
Step 2:
Use the concentration given and the formula for concentration (c = n/V ) to calculate the number of moles of NO2 in the equilibrium mixture. The volume of the container (2 dm3) was given. Show the calculation and substitute the calculated value in the table.
- c = n ∴ 0,4 = n ∴ n = (2)(0,4) = 0,8 mol
V 2
Step 3:
Find the column with Find the column with 2 values. Complete the mol used or produced line for this compound.
- 2 mol NO2 initially – ? mol NO2 used = 0,8 mol
∴ mol NO2 initial = 1,2 mol
Step 4:
From the balanced equation ratio 2 : 1 determine the ratio of the moles used and produced. Therefore 2 : 1 becomes 1,2 (as calculated in Step 3): 0,6
Step 5:
- For the reactants: initial mol – mol used = final mol at equilibrium.
- For the products: initial mol + mol produced = final mol at equilibrium.
Step 6:
Substitute the final mol values into the last line, and calculate the final concentration of the N2O4.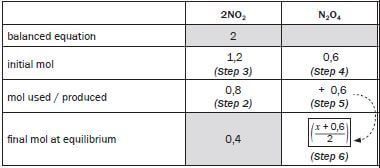
Step 7:
Write the expression for Kc and substitute all the known values – remember that the value of Kc was given. Solve for x.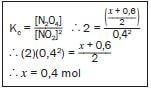
The plunger is now pushed into the container and the volume of the container decreases.
2. How will this change influence the amount of nitrogen dioxide at equilibrium? Only write down increases, decreases or remains the same.
Solution
Decreases
3. Use Le Châtelier’s principle to explain your answer to Question 2.2.
Solution
When the volume of the container decreases, the pressure increases.
According to Le Châtelier’s Principle the reaction that decreases the pressure will be favoured ∴ the (forward) reaction that produces fewer gas moles, 2 mol (g) ⇌ 1 mol (g), is favoured.
Type 3: The initial mass of a reactant and the value of Kc are given and the mass of the reactant that remains unreacted, is asked
Activity 7
The thermal decomposition of calcium carbonate (CaCO 3) is an example of a heterogeneous equilibrium. The decomposition that takes place in a closed container can be represented by the following equation:
CaCO3(s) ⇌ CaO(s) + CO2(g)
Initially 5 g of CaCO3(s) is placed in a closed 500 cm3 container and then heated. Equilibrium is reached at 900°C.
- Why is the above decomposition referred to as a heterogeneous equilibrium? (1)
- Calculate the mass of unreacted CaCO3(s) that remains in the container at equilibrium if Kc for the reaction is 0,0108 at 900°C. (19)
- It is found that the value of Kc increases when the container is heated. Is the forward reaction exothermic or endothermic? Use Le Châtelier’s principle to explain your answer. (5)
- The volume of the container is now decreased to 250 cm3 while the temperature is kept constant. How will each of the following be affected? Give a reason for your answer.
4.1 The value of Kc.
4.2 The number of mol CaCO3(s) present in the equilibrium mixture. (10)
4.3 The concentration of CO2(g) at the new equilibrium. - More CaCO3(s) is now added to the equilibrium mixture in the 500 cm3 container. How will this change influence the number of moles of CO2(g) in the equilibrium mixture? Give a reason for your answer. (2) [37]
- hint; Refer back to the definition of a heterogeneous equilibrium
Solutions
- Reactants and products are in different phases. 3 (1)
- This answer takes six steps. Answers for questions 3.3 to 3.5 appear below these steps.
Step 1:
Convert the mass of the reactant to moles. ONLY moles may be used in the table.
- M(CaCO3) = 40 + 12 + 3(16) = 100 g·mol–1
n = m = 5 = 0,05 mol
M 100
Step 2:
Write the expression for Kc and substitute all the known values – remember that the value of Kc was given.
- Kc = [CO2]
0,0108 = [CO2]
∴ [CO2] = 0,0108 mol·dm–3
Step 3:
Use the calculated CO2(g) concentration and the formula for concentration (c = n/V ) to calculate the number of moles of CO2 in the equilibrium mixture. The volume of the container (500 cm3) was given. Convert the volume to dm3. Show all working.
- c = n ∴ 0,0108 = n ∴ n = (0,5)(0,0108) = 0,0054 mol CO2
V 0,5
Step 4:
Find the column with 2 values. Complete the mol used or produced line for this compound. (0 mol CO2 initially + produced mol CO2 = 0,0054 mol
∴ mol CO2 produced = 0,0054 mol)
Step 5:
From the balanced equation ratio 1 : 1 : 1 determine the ratio of the moles used and produced. Therefore 1 : 1 : 1 becomes 0,0054 (from Step 3): 0,0054 : 0,0054
Step 6
For the reactant CaCO3 ... initial mol – mol used = final mol at equilibrium
∴0,05 mol – 0,0054 = final mol at equilibrium = 0,0046 mol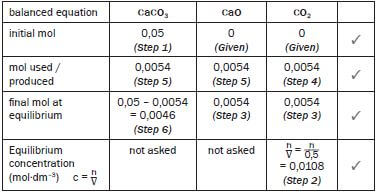
∴0,0046 mol CaCO3 remains unreacted at equilibrium
M(CaCO3) = 40 + 12 + 3(16) = 100 g·mol–1
- n = m ∴ 0,0046 = m ∴ m = (0,0046)(10) = 0,46 g
M 100
∴ 0,46g CaCO3 remains unreacted. (19)
Hint : Mass was asked, so convert the mol calculated to mass n = m
M
3. Endothermic. ✓
- Kc increases ∴ the forward reaction or reaction to the right is favoured. ✓✓
- According to Le Châtelier’s principle, an increase in temperature ✓ the forward reaction or will favour the endothermic reaction reaction to the right must be endothermic. ✓ (5)
4.1 Remains the same. Only temperature affects the value of Kc.
4.2 Increases. ✓✓
- Decreasing the volume increases the pressure. According to Le Châtelier’s Principle, when the pressure is increased, the reaction which decreases the pressure by producing fewer moles of gas products, is favoured. ✓
As 0 mol (g) ∴ 1 mol (g), the reverse reaction is favoured. ✓ - 4.3 Remains the same. ✓✓
Kc = [CO2]. The temperature remains constant ∴ Kc remains constant ∴ the concentration of CO2 will remain constant. ✓ (10)
5. Remains the same. Adding a solid does not affect the equilibrium, it does not change the concentration of a reagent. ✓✓ (2) [37]
Activity 8
NB:
- When∆H > 0 it means the reaction to the right (the forward reaction) is endothermic
- When∆H < 0 the forward reaction is exothermic
hint
- These are the initial concentrations – before the new equilibrium is established. V = 1 dm3 (given)
∴the values of the initial concentrations are equal to the initial number of moles.
c= n and c = n
V 1
∴ n = c(1)
The equation below represents an equilibrium reaction in a sealed 1dm3 container.
NO2(g) + NO(g) ⇋ N2O(g) + O2(g) ΔH > 0
Equilibrium was reached at a certain temperature and the value of Kc was 3,93. The concentration (in mol·dm–3) of each reactant and product in the container at equilibrium was:
- [NO2] = 0,06 mol·dm–3
- [NO] = 0,29 mol·dm–3
- [N2O] = 0,18 mol·dm–3
- [O2] = 0,38 mol·dm–3
One of the conditions affecting the equilibrium is changed and a new equilibrium is established. At the new equilibrium, the concentration of the NO2(g) is 0,12mol·dm–3.
1. Calculate the Kc value at the new equilibrium. (11)
2. Which condition, concentration or temperature, was changed? (1)
3. Give an explanation for the answer to Question 4.2. (1) [13]
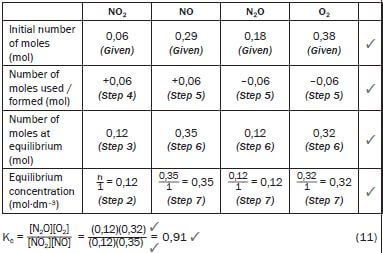
Solutions
- NO2(g) + NO(g) + Energy ⇌ N2O(g) + O2(g)
∆ [NO2] = [NO2]final − [NO2]initial = (0,12 – 0,06) = 0,06 mol NO2 is formed. but NO2 is the product of the reverse reaction
∴ the reverse reaction was favoured.From the balanced reaction equation, 1 NO2 : 1 NO : 1 N2O : 1 O2
∴0,06 mol N2O + 0,06 mol O2 → 0,06 mol NO2 + 0,06 mol NO
So at equilibrium:
Temperature.
Kc was initially 3,93 (given) now it is 0,91. If the Kc value changed, the temperature must have changed – only a change in temperature can change the value of Kc. (1)
Activity 9
Consider the following reaction equation for the production of ammonia in the Haber process.
NB
- The Haber Process is the name of the chemical process to manufacture NH3
N2(g) + 3H2(g) ⇌ 2NH3(g) ∆H < 0
In each of the following cases:
- State how Kc changes and
- Briefly explain your answer.
1. The concentration of the nitrogen gas is increased. (1)
2. The pressure in the reaction system is decreased. (1)
3. FeO is added to the reaction system. (1)
4. The reaction system is heated. (5) [8]
Solutions
1. Remains constant because... ✓ (1)
2. ... Kc is only affected by a change in temperature. ✓ (1)
3. Kc Decreases ✓ (1)
4. Heating the reaction system favours the endothermic, reverse reaction ✓ which decreases the temperature ✓ of the system ∴ the concentration of the forward product, [NH3] ✓ decreases while the concentrations of the forward reactants, [N2] and [H2] increase ✓ ∴ Kc decreases. ✓ (5) [8]
Activity 10
The reaction represented by the equation below reaches equilibrium.
Co(H2O)62+(aq) + 4Cℓ–(aq) ⇋ CoCℓ42–(aq) + 6H2O(ℓ) ΔH>0
pink blue
Which ONE of the following changes to the reaction mixture will changes its colour from blue to pink?
- Add a catalyst
- Place the reaction mixture in a container with hot water.
- Add a few drops of concentrated hydrochloric acid to the reaction mixture.
- D. Add water to the reaction mixture. (2) [2]
Solution
1. D [2]
3.6 Interpretation of graphs for chemical systems in dynamic equilibrium
Dynamic equilibrium in a chemical system can be represented graphically by a graph of:
- reaction rate versus time
- amount of each reagent (number of moles / mass / volume)
- concentration of each reagent (in mol·dm–3) versus time.
For each of these graphs:
- time is the independent variable and is therefore placed on the horizontal axis and
- either the concentration, amount or the reaction rate is measured and is therefore the dependent variable which is placed on the vertical axis.
e.g. Worked example 5
Equal amounts of SO2(g) and O2(g) react in a sealed container and reach equilibrium:
2SO2(g) + O2(g) ⇌ 2SO3(g) ∆H < 0

Pay special attention:
- We use square brackets [ ] to indicate the concentration of a substance
Steps to follow
Step 1: We need to interpret each part of the graph for each reactant and product, step by step as the reaction proceeds and
Step 2: identify what happens if there is a change in the rate/ concentration of each substance, which is visible by a change in the shape of the graph.
(i) When the reaction initially starts:
Initially
- The forward reaction rate is high because the [reactants] is initially high and
- The reverse reaction rate is zero as there are no products to react in the reverse reaction.
As the reaction takes place the
- concentrations of the reactants, [SO2] and [O2] decrease
- concentration of the forward product, [SO3] increases as forward reaction rate > the reverse reaction rate.
The forward reaction rate gradually decreases and the reverse reaction rate gradually increases.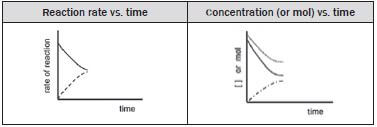
(ii) The reaction reaches a state of dynamic chemical equilibrium
The forward and reverse reactions take place at the:
- same time and
- same rate.
The concentrations of the reactants and of the products remain constant.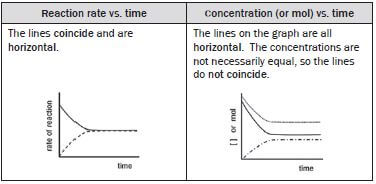
(iii) O2(g) is added to the system.
Adding O2 (g) to the system increases the concentration of the O2(g). O2(g) is a reactant in the forward reaction, therefore the rate of the forward reaction immediately shows a sharp increase.
According to Le Châtelier’s Principle:
- the reaction that opposes the change and decreases the concentration of the O2(g) is favoured so the
- forward reaction is favoured
- concentrations of the SO2(g) and O2(g) (forward reactants) decrease
- concentration of the SO3(g) (forward product) increases.
The rate of the
- forward reaction decreases as the [O2] and [SO2] decrease and
- reverse reaction increases as the [SO3] increases
- until a new dynamic equilibrium is established when the
- forward and reverse reaction rates are again equal and
- [O2] and [SO2] as well as the [SO3] again remain constant.
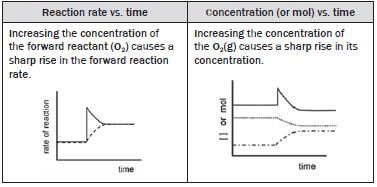
(iv) SO3(g) is removed from the system.
Removing SO 3(g) to the system decreases the concentration of the SO3 (g). SO3(g) is a reactant in the reverse reaction, therefore the rate of the reverse reaction immediately shows a sharp decrease.
According to Le Châtelier’s Principle:
- the reaction that opposes the change and increases the concentration of the SO3(g) is favoured so the
- forward reaction is favoured
- concentrations of the SO2(g) and O2(g) (forward reactants) decrease
- concentration of the SO3(g) (forward product) increases.
The rate of the
- forward reaction decreases as the [O2] and [SO2] decrease
- reverse reaction increases as the [SO3] increases
- until a new dynamic equilibrium is established when the
- forward and reverse reaction rates are again equal
- [O2] and [SO2] as well as the [SO3] again remain constant.
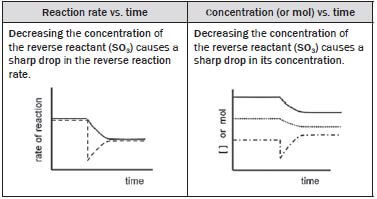
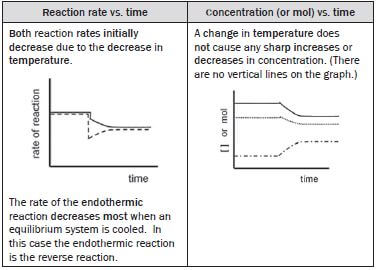
(v) The temperature of the equilibrium mixture is increased.
The heat of reaction is negative (∆H < 0) so the
- forward reaction is exothermic
- reverse reaction is endothermic.
Increasing the temperature of the system increases both the forward and the reverse reaction rates. The rate of the endothermic reaction always increases the most if the system’s temperature increases, so for this reaction, the reverse reaction rate increases the most.
According to Le Châtelier’s Principle:
- The reaction that opposes the change and decreases the temperature of the equilibrium system, is favoured so
- the endothermic reaction is favoured and therefore the
- reverse reaction is favoured and the
- concentration of the SO3(g) (reverse reactant) decreases and the
- concentrations of the SO2(g) and O2(g) (reverse products) increase and
The rate of the
- reverse reaction that was initially increased, decreases as the [SO3] decreases and
- forward reaction increases as the [O2] and [SO2] increase
- until a new dynamic equilibrium is established when the
- forward and reverse reaction rates are again equal and
- [O2] and [SO2] as well as the [SO3] again remain constant.
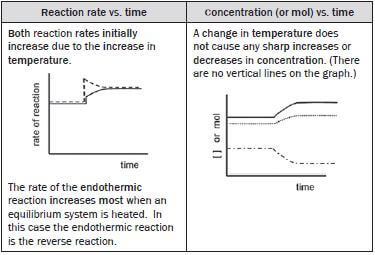
(vi) The temperature of the equilibrium mixture is decreased.
The heat of reaction is negative (∆H < 0) so the
- forward reaction is exothermic
- reverse reaction is endothermic.
Decreasing the temperature of the system decreases both the forward and the reverse reaction rates. The rate of the endothermic reaction always decreases the most if the system’s temperature decreases, so for this reaction, the reverse reaction rate decreases the most.
According to Le Châtelier’s Principle:
- the reaction that opposes the change and increases the temperature of the equilibrium system, is favoured so the
- exothermic reaction is favoured and therefore
- forward reaction is favoured
- concentrations of the SO2(g) and O2(g) (forward reactants) decrease and
- concentration of the SO3(g) (forward product) increases and
The rate of the
- forward reaction decreases as the [O2] and [SO2] decrease
- reverse reaction increases as the [SO3] increases
- until a new dynamic equilibrium is established when
- the forward and reverse reaction rates are again equal and
- the [O2] and [SO2] as well as the [SO3] again remain constant.
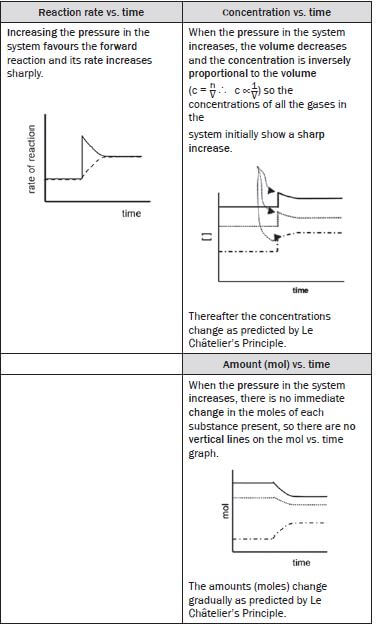
(vii) The pressure of the equilibrium mixture is increased
From the balanced reaction equation, the number of gaseous moles is:
- 2SO2(g) + 1O2(g) ⇌ 2SO3(g)
- 2 + 1 = 3 mol(g) ⇌ 2 mol (g)
According to Le Châtelier’s Principle:
- the reaction that opposes the change and decreases the pressure in the equilibrium system, is favoured so
- the reaction that produces the least moles of gas is favoured
- therefore the forward reaction is favoured and
- the concentrations of the SO2(g) and O2(g) (forward reactants) decrease and
- the concentration of the SO3(g) (forward product) increases and
The rate of the
- forward reaction decreases as the [O2] and [SO2] decrease
- reverse reaction increases as the [SO3] increases
- until a new dynamic equilibrium is established when
- the forward and reverse reaction rates are again equal and
- the [O2] and [SO2] as well as the [SO3] again remain constant.
viii). The pressure of the equilibrium mixture is decreased (the volume is increased). The same reasoning is applied as in the previous example and the graphs are as follows: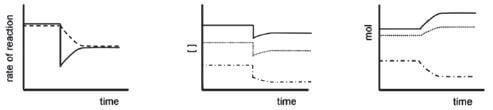
(ix) The addition of a suitable catalyst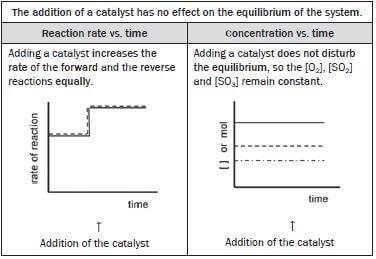
Activity 11
0,25 mol of A and 0,15 mol of B are introduced into a 1 dm3 vessel. A and B react and reach an equilibrium which can be represented by the following equation: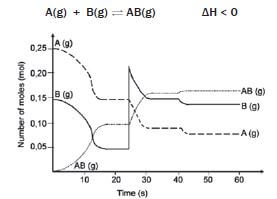
hint: Make use of the graph to read off the values
The graph shows the number of moles of A(g), B(g) and AB(g) vs time, under varying conditions.
- What are the concentrations of A(g), B(g) and AB(g) at t = 20 s? (3)
- How can we be sure that the system has reached equilibrium at t = 20 s? (1)
- 3. Give an explanation for the change that occurs at t = 25 s. (1)
- Explain the change in concentrations of A(g) and B(g) between t = 25 s and t = 35 s. (3)
- How does the equilibrium constant at t = 35 s compare with the equilibrium constant at t = 25 s? (No calculation is required.) (1)
- Assuming that the volume was kept constant, what was done at t = 40 s that produced the change as shown? Explain your answer. (5)
- Do any reactions occur during the interval t = 30 s and t = 40 s? Briefly explain your answer. (4) [18]
Solutions
- [A] = 0,15 mol·dm–3 [B] = 0,05 mol·dm–3 [AB] = 0,1 mol·dm–3 (3)
- The number of mol of A, B and AB remains constant from 16 s to 25 s. ✓(1)
- The amount of B is increased by adding B to the system, thus increasing the [B]. ✓(1)
- With the addition of B, [B] increases and the forward reaction is favoured ✓ according to Le Châtelier’s principle, so that some of B reacts with A to form more AB. Hence the [A] and [B] 3 decrease and [AB] increases. ✓ (3)
- Kc remains constant provided the temperature remains constant. ✓ (1)
- The temperature was lowered. ✓ It was not a concentration change, because there are no peaks at 25 s ✓. Therefore it must be a temperature change. From the graph, it can be seen that the forward reaction was favoured ✓ because there is an increase in the number of moles of AB, and a decrease in the number of moles of A and B when establishing the new equilibrium. ✓ According to the information given, the forward reaction is exothermic. ✓ According to Le Châtelier’s Principle, a decrease in temperature favours the exothermic reaction. (5)
- Yes, but since equilibrium has been reached, ✓ the forward and reverse reactions take place at the same time ✓ and at the same ✓ rate, so that no changes occur in the concentrations of A, B or AB. ✓ (4) [18]
Activity 12
Nitrogen and oxygen gases react in a sealed container according to the following equation:
O2(g) + N2(g) ⇋ 2NO(g)
After the reaction reaches equilibrium, certain changes are made. The following graph of rate of reaction versus time represents the situation.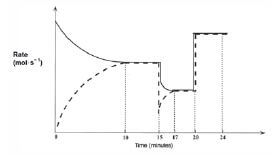
- Write the equation for the reaction represented by the dashed line on this graph. (2)
- What is represented by the section of the graph between the 10th and 15th minute? Explain your answer. (2)
- A temperature change takes place at t = 15 minutes.
3.1 Was the temperature increased or decreased at t = 15 minutes? (1)
3.2 Explain whether the forward reaction is exothermic or endothermic. (3)
3.3 What effect does this temperature change have on the equilibrium constant (Kc)? Explain your answer. (3) - A pressure change is introduced at t = 20 minutes.
4.1 Was the pressure increased or decreased? (3)
4.2 Explain how this change in pressure affects the amounts of each gas at equilibrium. (2) [16]
Solutions
- 2NO(g) → N2(g) + O2(g) ✓✓ (2)
- The system is in equilibrium ✓ because the rates of the forward and reverse reactions are equal. ✓ (2)
- 3.1 Decreased. ✓ (1)
3.2 The rates of both the forward and the reverse reactions decrease ✓, but the rate of the reverse reaction decreases more. ✓ The rate of the endothermic reaction will always decrease the most when a reaction mixture is cooled. Thus the reverse reaction is endothermic. That means that the forward reaction must be exothermic and was favoured by the decrease in temperature. ✓
O2(g) + N2(g) ⇌ 2NO(g) + Energy (3)
3.3 Increases. ✓
Since Kc = [NO]2
[N2][O2] (3)
and the forward reaction is favoured ✓ which causes an increase in [NO], the value of Kc increases. ✓ (3) - 4.1 Increased. ✓ The pressure is probably caused by a volume decrease. This results in the concentrations (c = n/V ) of the reactants, as well as the products, to increase ✓ , which in turn causes an equal increase in the forward and reverse reaction rates. ✓ (3)
4.2 The amount (n) of reactants and products does not change, ✓ since 2 moles of gaseous reactant molecules ⇌ 2 moles of gaseous product molecules. ✓ (2) [16]
Activity 13
A fertiliser company produces ammonia on a large scale at a temperature of 450°C. The balanced equation below represents the reaction that takes place in a sealed container.
N2(g) + 3H2(g) ⇌ 2NH3(g) ∆H < 0
To meet an increased demand for fertiliser, the management of the company instructs their engineer to make the necessary adjustments to increase the yield of ammonia. In a trial run on a small scale in the laboratory, the engineer makes adjustments to the TEMPERATURE, PRESSURE and CONCENTRATION of the equilibrium mixture. The graphs below represent the results obtained.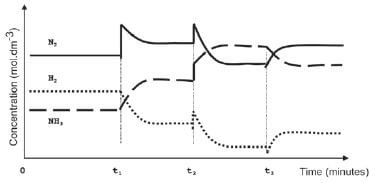
- Identify the changes made to the equilibrium mixture at each of the following times:
1.1 t1 (2)
1.2 t2 (2)
1.3 t3 (2) - At which of the above time(s) did the change made to the reaction mixture lead to a higher yield of ammonia? Write down only t1 and/or t2 and/or t3 (2)
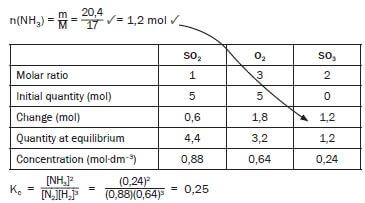
- The engineer now injects 5 mol N2 and 5 mol H2 into a 5 dm3 sealed empty container. Equilibrium is reached at 450 °C. Upon analysis of the equilibrium mixture, he finds that the mass of NH3 is 20,4 g. Calculate the value of the equilibrium constant (Kc) at 450 °C (9) [17]
Solutions
- 1.1 The concentration of nitrogen ✓ is increased ✓ / More Nitrogen ✓ added ✓ (any two) (2)
1.2 The pressure ✓ on the system is increased ✓ (2)
1.3 The temperature ✓ is increased ✓ (2) - t1 3and t2 ✓ (2)
- Pay special attention:
This is how marks are allocated to the answer of this Kc calculation:- Use of n = m/M ✓
- n(NH3) at equilibrium = 1,2 mol ✓
- Using ratio n(N2) : n(H2) : n(NH3) = 1 : 3 : 2 ✓
- n(N2) at equilibrium (initial - change) ✓
- n(H2) at equilibrium (initial - change) ✓
- divide by volume (calculation of concentration) ✓
- Kc expression ✓
- Substitution into Kc expression ✓
- Final answer ✓ (9)
 [17]
[17]
Activity 14
2 mol of NO2(g) and an unknown amount of N2O4(g) are sealed in a 2 dm3 container, that is fitted with a plunger, at a certain temperature. The following reaction takes place:
2NO2(g) ⇌ N2O4(g)
At equilibrium it is found that the NO2 concentration is 0,4 mol·dm–3. The equilibrium constant at this temperature is 2.
- Calculate the initial amount (in mol) of N2O4(g) that was sealed in the container. (8)
The plunger is now pushed into the container causing the pressure of the enclosed gas to increase by decreasing the volume - How will this change influence the amount of nitrogen dioxide at equilibrium? Only write down INCREASES, DECREASES or REMAINS THE SAME. (1)
- Use Le Châtelier’s principle to explain your answer to QUESTION 2.1.2. (2) [11]
Solutions
1.
| 2NO2 | N2O4 | |
| Initial number of mole (mol) | 2 | x |
| Number of moles used/formed (mol) | -1,2 | +0,6 |
| Number of moles at equilibrium(mol) | 0,8 | x + 0,6 |
| Equilibrium concentration (mol·dm -3) | 0,4 | (x + 0,6) 2 |
- Kc = [N2O4]
[NO2]2
2 = (x + 0,6)2
(0,4)2
x = 0,004 mol
2. Decreases (1)
3. When the pressure is increased the system will try to decrease the pressure. The forward reaction (2 mol to 1 mol) is favoured. (2) [11]
3.7 Applications of equilibrium principles in the chemical industry
Many industrial processes involve reversible chemical reactions that reach equilibrium.
Two factors that are important from an economic point of view are the yield (amount of products produced relative to the amount of reactants) and the reaction rate (rate at which the products are formed).
hint: The success of chemical processes in both the laboratory and the industry depends on: the amount of product that is produced and how cost effective this production is.
NB: Remember Standard atmospheric pressure (1 atm) is the atmospheric pressure at sea level.
- 1 atm = 101,3 kPa = 101 325 Pa (pascal)
| Haber Process | Step 2 of the Contact Process | |
| Nitrogen, N2(g) Hydrogen, H2(g) | Reactants | Sulphur dioxide, SO2(g) Oxygen, O2(g) |
| Source of reactants |
|
| Ammonia, NH3(g) | Products | Sulphur trioxide, SO3(g) |
| N2(g) + 3H2(g) ⇋ 2NH3(g) | Reaction equation | 2SO2(g) + O2(g) ⇋ 2SO3(g) |
| 4 mol (g) : 2 mol (g) | Gas mol ratio | 3 mol (g) : 2 mol (g) |
| ∆H<0 ∴ forward reaction is exothermic | Heat of reaction | ∆H<0 |
| Increase the reaction rate by |
|
| Increase the yield by |
|
Temperature: approx. 450 °C | Optimal Reaction conditions | Temperature: approx. 450 °C |
Fe (iron) OR FeO (iron(II)oxide) | Catalyst | V2O5 (vanadium pentoxide) |
NB
- When the reaction system is in a state of dynamic equilibrium, high temperatures will increase the reaction rates BUT will decrease the yield of the products. Both the forward reactions are exothermic, therefore high temperature will, according to Le Châtelier’s Principle, favour the reverse endothermic reactions. This makes the reactions economically less viable!
- Temperatures of ± 450 °C are maintained in both the Haber and Contact processes.
- Industrial processes at high pressures require powerful compressors and strong reaction vessels which are very expensive. This makes the reactions economically less viable! Industrial plants that operate at high pressures also pose safety risks for the workers.
- It is therefore necessary to find a compromise between the reaction rates, the yield, and the higher costs.
- A pressure of 200 times that of atmospheric pressure is maintained in the Haber process to ensure that the yield is high enough.
- The yield of SO3 in the Contact Process is however more than 90% at a pressure of 1 atm and therefore it is economically viable to keep the reaction at atmospheric pressure. It is unnecessary to maintain higher pressures and this saves unnecessary costs.
Activity 15
Name the industrial process for the production of ammonia (1) [1]
Solution
- Haber (Bosch) process [1]
Activity 16
Sulphuric acid is an important substance used in the manufacture of fertilisers. The equation below represents one of the steps in the industrial preparation of sulphuric acid.
2SO2(g) + O2(g) ⇋ 2SO3(g) ∆H < 0
- Write down the name of the process used to prepare sulphuric acid in industry. (1)
- Write down the NAME or FORMULA of the catalyst used in the process in question (1)
- Is the forward reaction exothermic or endothermic? Give a reason for the answer. The reaction reaches equilibrium at a certain temperature in a 2 dm3 closed container. On analysis of the equilibrium mixture, it is found that 0,6 mol of SO2(g), 0,5 mol of O2(g) and 0,4 mol of SO3(g) are present in the container. (1)
- List THREE changes that can be made to this equilibrium to increase the yield of SO3(g) (3)
- The temperature is NOW increased and the reaction is allowed to reach equilibrium for the second time at the new temperature. On analysis of this new equilibrium mixture, it is found that 0,2 mol of for this reaction at the new temperature. (8) [14]
Solutions
- Contact process ✓ (1)
- V2O5 (vanadium pentoxide) ✓ (1)
- Exothermic∆H < 0 ✓ (1)
- Any three: ✓✓ ✓
Decrease temperature
Increase pressure
Increase concentration of both/any one of the reactants
Remove SO3 continuously (3) -
Kc = [SO3]2 = (0,1)2 = 0,21SO2 O2 SO3 Molar ratio 2 1 2 Initial quantity (mol) 0,6 0,5 0,4 Change (mol) 0,2 0,1 0,2 Quantity at equilibrium 0,8 0,6 0,2 Concentration (mol·dm–3) 0,4 0,3 0,1
[SO2]2[O2] (0,4)2(0,3)
NB
This how marks are allocated to the answer of this Kc calculation:
- Change in n(SO3) = 0,2 mol ✓
- Ratio n(SO2) : n(O2) : n(SO3) = 2 : 1 : 2 ✓
- n(SO2) at equilibrium = initial + change ✓
- n(O2) at equilibrium = initial + change ✓
- divide three equilibrium amounts by 2 (calculation of concentration) ✓
- Kc expression ✓
- Substitution into Kc expression ✓
- Final answer ✓ (8) [14]
Activity 17
The reaction below represents the catalysed step in the contact process:
2SO2(g) + O2(g) ⇋ 2SO3(g) ∆H <0
The reaction takes place in a closed container and reaches equilibrium at 427°C. How will a HIGHER temperature affect each of the following? Write down only INCREASES, DECREASES or REMAINS THE SAME.
- The rate of production of SO3(g) (2)
- The yield of SO3(g) (2)
- The reaction is investigated on a small scale in the laboratory. Initially 4 mol of SO2(g) and an unknown mass, x, of O2(g) are sealed in a 2 dm–3 flask and allowed to reach equilibrium at a certain temperature. At equilibrium it is found that the concentration of SO3(g) present in the flask is 1,5 mol·dm–3. Calculate the mass of O2(g) initially present in the flask if the equilibrium constant (Kc) at this temperature is 4,5. (8) [12]
Solutions
1. Increases ✓✓ (2)
2. Decreases ✓✓ (2)
3.
| SO2 | O2 | SO3 | |
| Molar ratio | 2 | 1 | 2 |
| Initial quantity (mol) | 4 | x 32 | 0 |
| Change (mol) | 3 | 1,5 | 3 ratio |
| Quantity at equilibrium | 1 | x - 1,5 32 | 3 |
| Concentration (mol ·dm –3 | c = n = 1 = 0,5 V 2 | (x - 48) 64 | 3/2 = 1,5 (divide by 2) |
 (8) [12]
(8) [12]