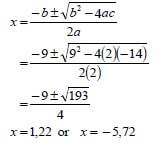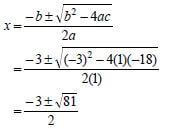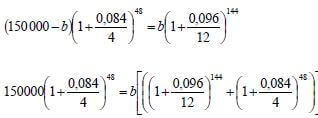MATHEMATICS PAPER 1 GRADE 12 MEMORANDUM - AMENDED SENIOR CERTIFICATE EXAMS PAST PAPERS AND MEMOS MAY/JUNE 2018
Share via Whatsapp Join our WhatsApp Group Join our Telegram GroupMATHEMATICS
PAPER 1
GRADE 12
AMENDED SENIOR CERTIFICATE EXAMS
PAST PAPERS AND MEMOS
MAY/JUNE 2018
MEMORANDUM
NOTE:
- If a candidate answers a question TWICE, only mark the FIRST attempt.
- Consistent accuracy applies in ALL aspects of the marking guidelines.
QUESTION 1
1.1.1 | (3x −1 )(x + 4)= 0 | ✓ x = 1 |
1.1.2 | 2x2 +9x−14 = 0 OR | ✓ substitution into correct formula OR ✓ for adding 81 on both sides |
1.1.3 | √3 − 26x = 3x | ✓ 3 − 26x = 9x2 |
1.1.4 | (x −1 )(x − 4)> x +11 | ✓ x2 −5x + 4 |
1.2 | 4√x7 - 5√x7 OR √x7(4−5) = −x3 OR
| ✓ 4√x7 - 5√x7 OR ✓ √x7(4−5) OR ✓ 4x7/2 - 5x7/2 |
1.3 | x−2y−3=0 OR y = x - 3 ................ (1) OR x−2y−3=0 x = 2[9] +3 | ✓ x=2y+3 OR ✓ y = x -3 OR ✓ y = 9 |
1.4 | x2 + 2xy + 2y2 | ✓ x2 + 2xy + y2 +y2 |
QUESTION 2
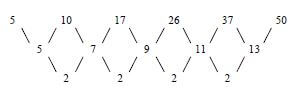
2.1.1 |
| ✓ 37 |
2.1.2 | a = second difference = 2 =1 | ✓ second difference of 2 |
2.1.3 | n2 + 2n + 2 =1765 OR n2 +2n+2 =1765 | ✓ equating Tn to 1765 OR ✓ equating Tn to 1765 |
2.2 | Sum of multiples of 7 from 35 to 196: Sum of all natural numbers from 35 to 196: | ✓ correct a, d and n substitution into correct formula |
QUESTION 3
3.1 | r = 0,94; a =100 | ✓ r = 0,94 |
3.2 | Sn = a(rn −1 ) He will pass the halfway point on the 10th day | ✓ substitution into correct formula |
3.3 | S∞ = a | ✓ use of S∞ formula |
QUESTION 4
4.1 | 0< x ≤1 or (0 ;1] | ✓✓ answer (2) |
4.2 | p = log4/3 16/9 [4]2 = [4] | ✓ substitution |
4.3 | f : y = log4/3 x | ✓ x = log 4/3 y ✓ y = [4]x (2)
|
4.4 | y > 0 or y∈(0;∞) | ✓✓ answer (2) |
4.5 | [−2 ; 16/9] | ✓ -2 |
QUESTION 5
5.1 | x∈R; x ≠ −1 | ✓ x∈R |
5.2 | x-intercept of f: | ✓ equating to 0 |
5.3 | y = 2 + 4 | ✓ substitution |
5.4 | C(2; 4) | ✓ 2 |
5.5 | y = a(x + p)2 + q | ✓ a(x−2)2 +4 |
5.6 | x≤− 3 or −1<x < 0 or x > 4 | ✓ x ≤ − 3/2 |
5.7 | 2 − 5: f shifted 1 unit to the right and 9 units down. | ✓ both shifted 1 unit to the right |
QUESTION 6
6.1 | A = P(1−i)n | ✓ A = 0,5P |
6.2 | In account one month before his 55th birthday: OR
| ✓ value of i OR ✓ value of i |
6.3 | Invest Rx in account A paying 8,4% p.a. compounded quarterly. OR a = amount invested at 8,4% p.a. compounded quarterly | ✓ x [1 + 0,084/4]48 OR ✓[1 + 0,096/12]144 |
QUESTION 7
Penalize 1 mark for incorrect notation in the whole question.
7.1 |  OR 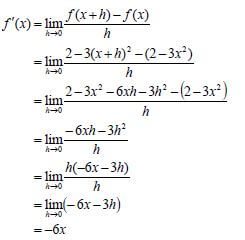 | ✓ 2−3x2 −6xh −3h2 OR ✓ subst. into formula |
7.2.1 | Dx[4x +5)2] | ✓ 16x2 + 40x + 25 |
7.2.2 |
| ✓ x¼ |
QUESTION 8
8.1 | C(0;12) | ✓ C(0;12) (1) |
8.2 | 3 − x +13x +12 = 0 3 x −13x −12 = 0 2 (x +1)(x − x −12)= 0 (x +1 )(x − 4)(x +3)= 0 A( − ; ) B(4;0) | ✓ f (x) = 0 |
8.3 | fI (x)= −3x2 +13 OR g(x) = x3 - 13x -12 OR f (x)= −3x2 +13 | ✓ fI (x)= −3x2 +13 OF OR |
8.4 | FIx)= −3x2 +13 | ✓ equating derivative to - 14 |
QUESTION 9
9.1.1 | AC = t - 30 | ✓ answer (1) |
9.1.2 | 302 =(t−30)2 + p2 [Pythagoras] | ✓ p2 = 900 −(t −30 )2 |
9.2 | V (t)= 1/3 π r2t | ✓ substitution (1) |
9.3 | V (t)= 20π t2 − 1/3πt3 | ✓ 40π t |
9.4 | Volume of cone | ✓ volume of cone |
QUESTION 10
10.1 | 10! | ✓ 10! |
10.2 | 4!× 7! OR 4!×6!×7 | ✓ 4! OR ✓ 4! |
10.3 | 6! | ✓ 6!
|
QUESTION 11
11.1 | P(tennis)× P(≤35years)= P(tennis and ≤ 35years ) | ✓ statement |
11.2 | P(gym or ≤35 years ) | ✓ statement
|
TOTAL: 150