PHYSICAL SCIENCES PAPER 1 GRADE 12 QUESTIONS - NSC EXAMS PAST PAPERS AND MEMOS NOVEMBER 2020
Share via Whatsapp Join our WhatsApp Group Join our Telegram GroupPHYSICAL SCIENCES: PHYSICS (PAPER 1)
GRADE 12
NOVEMBER 2020
NATIONAL SENIOR CERTIFICATE
INSTRUCTIONS AND INFORMATION
- Write your examination number and centre number in the appropriate spaces on the ANSWER BOOK.
- This question paper consists of TEN questions. Answer ALL the questions in the ANSWER BOOK.
- Start EACH question on a NEW page in the ANSWER BOOK.
- Number the answers correctly according to the numbering system used in this question paper.
- Leave ONE line between two subquestions, e.g. between QUESTION 2.1 and QUESTION 2.2.
- You may use a non-programmable calculator.
- You may use appropriate mathematical instruments.
- Show ALL formulae and substitutions in ALL calculations.
- Round off your FINAL numerical answers to a minimum of TWO decimal places.
- Give brief motivations, discussions, etc. where required.
- You are advised to use the attached DATA SHEETS.
- Write neatly and legibly.
QUESTION 1: MULTIPLE-CHOICE QUESTIONS
Various options are provided as possible answers to the following questions. Choose the answer and write only the letter (A–D) next to the question numbers (1.1 to 1.10) in the ANSWER BOOK, e.g. 1.11 E.
1.1 The rate of change of momentum of an object is equal to the …
- impulse on the object.
- net force acting on the object.
- product of the object's mass and its change in velocity.
- product of the net force acting on the object and its acceleration. (2)
1.2 The gravitational acceleration on the surface of planet X with mass M and radius r is g.
The gravitational acceleration on the surface of planet Y with mass 2M and radius ½r is …
- ½g
- g
- 4g
- 8g (2)
1.3 The graph below shows how one of the physical quantities associated with an object in free fall changes with time t. The label on the y-axis is omitted. Ignore air friction.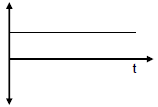
Which ONE of the following physical quantities can be the label on the y-axis?
- Velocity
- Position
- Weight
- Momentum (2)
1.4 A ball of mass m, falling vertically downwards, hits the floor at a speed v and bounces vertically upwards at a speed 0,75v.
Which ONE of the following combinations regarding the change in momentum of the ball during the collision is CORRECT?
| MAGNITUDE | DIRECTION | |
| A | 0,25mv | Upwards |
| B | 0,25mv | Downwards |
| C | 1,75mv | Upwards |
| D | 1,75mv | Downwards |
(2)
1.5 The base SI unit of the physical quantity 'work' is …
- kg∙m∙s-1
- kg∙m2∙s2
- kg∙m2∙s-2
- kg∙m∙s-2 (2)
1.6 The siren of a police car, moving in front of a truck, emits sound waves of frequency f. Both vehicles are travelling at the same constant velocity.
The frequency of the sound heard by the driver of the truck is …
- f.
- zero.
- greater than f.
- smaller than f. (2)
1.7 Two identical metal spheres, P and R, on insulated stands, carry different charges. The spheres are brought into contact and then separated again.
If the charge on sphere R AFTER the separation is q, the charge on sphere P after the separation is …
- q.
- zero.
- less than q.
- greater than q. (2)
1.8 An AC generator generates a current with a frequency of 50 Hz.
The number of times that the maximum (peak) current is produced in one second is …
- 25.
- 50.
- 75.
- 100. (2)
1.9 In the circuit below, the battery has an internal resistance r and an emf .
A variable resistor R is connected in the circuit and the ammeter and voltmeter register readings.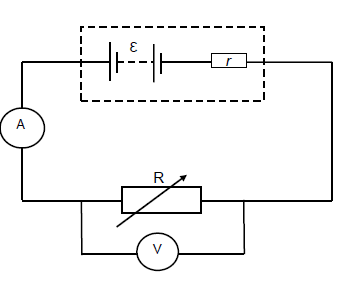
The resistance of the variable resistor R is INCREASED now.
Which ONE of the following combinations is the CORRECT representation of the change in the readings on the ammeter and voltmeter as the resistance of R is increased?
| AMMETER READING | VOLTMETER READING | |
| A | Decreases | Increases |
| B | Increases | Increases |
| C | Increases | Decreases |
| D | Decreases | Decreases |
(2)
1.10 The sodium cathode of a photocell is irradiated with ultraviolet light as shown in the diagram below. The ammeter registers a current.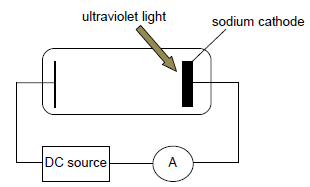
Which ONE of the following changes will INCREASE the ammeter reading?
- Use a thinner sodium cathode.
- Increase the intensity of the ultraviolet light.
- Increase the frequency of the ultraviolet light.
- Replace the sodium cathode with a cathode of lower work function(2)
[20]
QUESTION 2 (Start on a new page.)
A 20 kg block, resting on a rough horizontal surface, is connected to blocks P and Q by a light inextensible string moving over a frictionless pulley. Blocks P and Q are glued together and have a combined mass of m.
A force of 35 N is now applied to the 20 kg block at an angle of 40° with the horizontal, as shown below.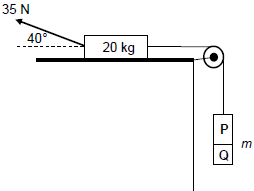
The 20 kg block experiences a frictional force of magnitude 5 N as it moves to the RIGHT at a CONSTANT SPEED.
2.1 Define the term normal force. (2)
2.2 Draw a labelled free-body diagram of the 20 kg block. (5)
2.3 Calculate the combined mass m of the two blocks. (5)
2.4 At a certain stage of the motion, block Q breaks off and falls down.
How will EACH of the following be affected when this happens?
2.4.1 The tension in the string
Choose from INCREASES, DECREASES or REMAINS THE SAME. (1)
2.4.2 The velocity of the 20 kg block
Explain the answer. (3)
[16]
QUESTION 3 (Start on a new page.)
A small ball is dropped from a height of 2 m and bounces a few times after landing on a cement floor. Ignore air friction.
The position-time graph below, not drawn to scale, represents the motion of the ball.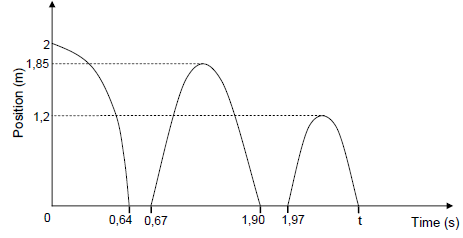
3.1 Define the term free fall. (2)
3.2 Use the graph and determine:
3.2.1 The time that the ball is in contact with the floor before the first bounce (2)
3.2.2 The time it takes the ball to reach its maximum height after the first bounce (2)
3.2.3 The speed at which the ball leaves the floor at the first bounce (3)
3.2.4 Time t indicated on the graph (6)
[15]
QUESTION 4 (Start on a new page.)
Ball P of mass 0,16 kg, moving east at a speed of 10 m∙s-1, collides head-on with another ball Q of mass 0,2 kg, moving west at a speed of 15 m∙s-1. After the collision, ball P moves west at a speed of 5 m∙s-1, as shown in the diagram below.
Ignore the effects of friction and the rotational effects of the balls.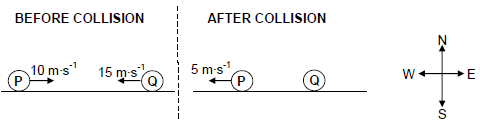
4.1 Define the term momentum in words. (2)
4.2 Calculate the:
4.2.1 Velocity of ball Q after the collision (5)
4.2.2 Magnitude of the impulse on ball P during the collision (3)
[10]
QUESTION 5 (Start on a new page.)
A roller-coaster car of mass 200 kg, with the engine switched off, travels along track ABC which has a rough surface, as shown in the diagram below. At point A, which is 10 m above the ground, the speed of the car is 4 m·s-1.
At point B, which is at a height h above the ground, the speed of the car is 2 m·s-1. During the motion from point A to point B, 3,40 × 103 J of energy is used to overcome friction.
Ignore rotational effects due to the wheels of the car.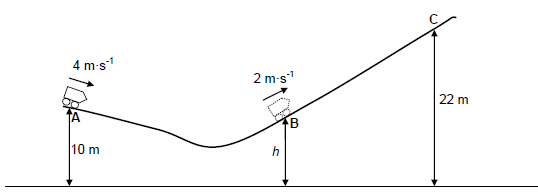
5.1 Define the term non-conservative force. (2)
5.2 Calculate the change in the kinetic energy of the car after it has travelled from point A to point B. (3)
5.3 Use energy principles to calculate the height h. (4)
On reaching point B, the car's engine is switched on in order to move up the incline to point C, which is 22 m above the ground. While moving from point B to point C, the car travels for 15 s at a constant speed of 2 m·s-1, while an average frictional force of 50 N acts on it.
5.4 Calculate the power delivered by the engine to move the car from point B to point C. (5)
[14]
QUESTION 6 (Start on a new page.)
The siren of a train, moving at a constant speed along a straight horizontal track, emits sound with a constant frequency. A detector, placed next to the track, records the frequency of the sound waves. The results obtained are as shown in the graph below.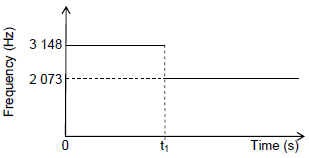
6.1 State the Doppler effect in words. (2)
6.2 Does the detector record the frequency of 3 148 Hz when the train moves TOWARDS the detector or AWAY from the detector? (1)
6.3 Calculate the speed of the train. Take the speed of sound in air as 340 m∙s-1. (6)
6.4 The detector started recording the frequency of the moving train's siren when the train was 350 m away.
Calculate time t1 indicated on the graph above. (2)
[11]
QUESTION 7 (Start on a new page.)
Two small charged spheres, A and B, are placed on insulated stands, 0,2 m apart, as shown in the diagram below. They carry charges of −4 x 10-6 C and +3 x 10-6 C respectively.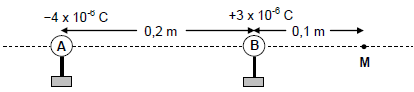
M is a point that is a distance of 0,1 m to the right of sphere B.
7.1 Calculate the number of electrons in excess on sphere A. (3)
7.2 Calculate the magnitude of the electrostatic force exerted by sphere A on sphere B. (3)
7.3 Describe the term electric field. (2)
7.4 Calculate the magnitude of the net electric field at point M. (5)
Charged spheres A and B and another charged sphere D are now arranged along a rectangular system of axes, as shown in the diagram below.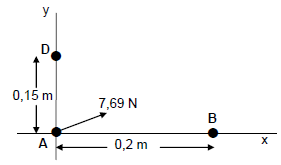
The net electrostatic force experienced by sphere A is 7,69 N in the direction as shown in the diagram above.
7.5 Is the charge on sphere D POSITIVE or NEGATIVE? (1)
7.6 Calculate the magnitude of the charge on sphere D. (3)
[17]
QUESTION 8 (Start on a new page.)
A battery with an internal resistance of 0,5 Ω and an unknown emf (ε) is connected to three resistors, a high resistance voltmeter and an ammeter of negligible resistance, as shown in the circuit diagram below.
The resistance of the connecting wires must be ignored.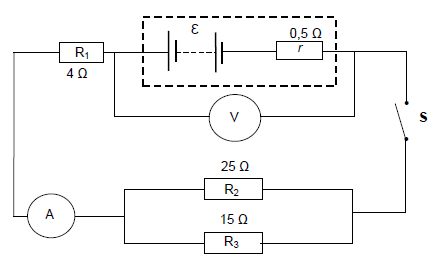
8.1 Define the term emf of a battery. (2)
The reading on the voltmeter DECREASES by 1,5 V when switch S is closed.
8.2 Give a reason why the voltmeter reading decreases. (2)
8.3 Calculate the following when switch S is closed:
8.3.1 Reading on the ammeter (3)
8.3.2 Total external resistance of the circuit (4)
8.3.3 Emf of the battery (3)
8.4 A learner makes the following statement:
The current through resistor R3 is larger than the current through resistor R2.
Is this statement CORRECT? Choose from YES or NO. Explain the answer. (3)
8.5 The 4 Ω resistor is now removed from the circuit.
How will this affect the emf of the battery? Choose from INCREASES, DECREASES or REMAINS THE SAME. (1)
[18]
QUESTION 9 (Start on a new page.)
9.1 A simplified diagram of an electrical machine is shown below.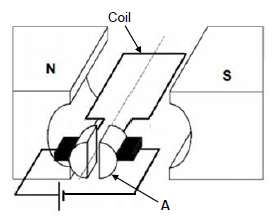
9.1.1 Is this machine a DC motor or a DC generator? (1)
9.1.2 Write down the energy conversion that takes place while this machine is in operation. (2)
9.1.3 Write down the name of component A in the diagram. (1)
9.1.4 In which direction will the coil, shown in the diagram above, rotate? Choose from CLOCKWISE or ANTICLOCKWISE. (2)
9.2 An electrical device is marked 200 W ; 220 V.
9.2.1 Define the term rms voltage. (2)
9.2.2 Calculate the resistance of the device. (3)
This device is now connected to a 150 V AC source.
9.2.3 Calculate the energy dissipated by the device in 10 minutes. (5)
[16]
QUESTION 10 (Start on a new page.)
An experiment is conducted to investigate the relationship between the frequency of light incident on a metal and the maximum kinetic energy of the emitted electrons from the surface of the metal. This experiment is conducted for three different metals.
The graph below represents the results obtained.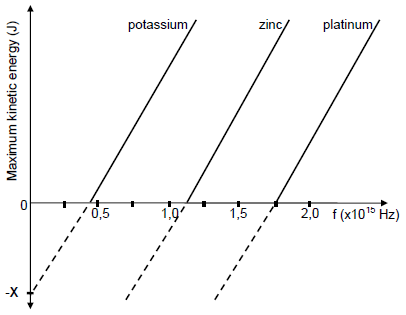
10.1 Name the phenomenon on which this experiment is based. (1)
10.2 Name the physical quantity represented by X on the graph. (1)
10.3 Which ONE of the three metals needs incident light with the largest wavelength for the emission of electrons?
Give a reason for the answer. (2)
10.4 Define the term work function in words.(2)
10.5 Calculate the:
10.5.1 Work function of platinum (3)
10.5.2 Frequency of the incident light that will emit electrons from the surface of platinum with a maximum velocity of 5,60 x 105 m∙s-1 (4)
[13]
TOTAL: 150
DATA FOR PHYSICAL SCIENCES GRADE 12
PAPER 1 (PHYSICS)
NAME | SYMBOL | VALUE |
Acceleration due to gravity | g | 9,8 m•s-2 |
Universal gravitational constant | G | 6,67 × 10-11 N•m2•kg-2 |
Speed of light in a vacuum | c | 3,0 × 108 m•s-1 |
Planck's constant | h | 6,63 × 10-34 J•s |
Coulomb's constant | k | 9,0 × 109 N•m2•C-2 |
Charge on electron | e | -1,6 × 10-19 C |
Electron mass | me | 9,11 × 10-31 kg |
Mass of earth | M | 5,98 × 1024 kg |
Radius of earth | RE | 6,38 × 103 km |
TABLE 2: FORMULAE
MOTION
| vf = vi + aΔt | Δx = ViΔt + ½aΔt2 or Δy = ViΔt2 + ½aΔt2 |
Vf2 = Vi2 + 2aΔx or Vf2 = vi2 + 2aΔy | Δx = [Vi + Vf]Δt or Δy = [Vi + Vf]Δt |
FORCE
Fnet = ma | p= mv |
fsmax = µsN | fk = µkN |
FnetΔt = Δp | w =mg |
F = Gm1m2 | g = G M |
WORK, ENERGY AND POWER
W =FΔxcosθ | U= mgh or EP = mgh |
K = ½mv2 or Ek = ½mv2 | Wnet = ΔK or Wnet = ΔEk ΔK = Kf −Ki or ΔEk =Ekf − Eki |
Wnc= ΔK + ΔU or Wnc= ΔEk + ΔEp | P = W Δt |
Pav = Fv |
WAVES, SOUND AND LIGHT
v = f λ | T =1/f |
fl = v ± vl fs fl = v ± vl fb | E = hf or E = h c |
E = W0 + Ek where | |
ELECTROSTATICS
| F = kQ1Q2 r2 | E = KQ |
E = V | E = F |
V = W | n = Q |
ELECTRIC CIRCUITS
R = V | emf (ε) = I(R + r) |
RS = R1 + R2 + ....... | q = I Δt |
W = Vq | P= W |
ALTERNATING CURRENT
I rms = Imax | Paverage = VrmsIrms |