GRADE 12 MATHEMATICAL LITERACY
PAPER ONE (P1) 
NSC EXAM PAPERS AND MEMOS
NOVEMBER 2016

INSTRUCTIONS AND INFORMATION 

1. This question paper consists of FIVE questions. Answer ALL the questions. 
2.    
   1.  Use the ANNEXURES in the ADDENDUM to answer the following questions:
      ANNEXURE A for QUESTION 1.1
      ANNEXURE B for QUESTION 3.1
      ANNEXURE C for QUESTION 3.2
      ANNEXURE D for QUESTION 5 
   2. Answer QUESTION 1.2.4(a) on the attached ANSWER SHEET 1.
      Answer QUESTION 4.2.6 on the attached ANSWER SHEET 2.
   3. Write your centre number and examination number in the spaces on the ANSWER SHEETS. Hand in the ANSWER SHEETS with your ANSWER BOOK.
3. Number the answers correctly according to the numbering system used in this question paper.
4. Start EACH question on a NEW page.
5. You may use an approved calculator (non-programmable and non-graphical), unless stated otherwise,
6. Show ALL calculations clearly.
7. Round off ALL final answers appropriately according to the given context, unless stated otherwise.
8. Indicate units of measurement, where applicable.
9. Maps and diagrams are NOT necessarily drawn to scale, unless stated otherwise.
10. Write neatly and legibly. 

QUESTIONS

QUESTION 1 
1.1 

Use ANNEXURE A to answer the questions that follow. 

1.1.1 Give the name of the borrower. 
1.1.2 State the end date (month and year) of the loan.  (2)
1.1.3 Calculate the difference between the insured value of the property and the registered bond amount.  (2)
1.1.4 Determine the total administration fee payable for the whole loan period.  (3)
1.1.5 On 30 January 2014 the interest rate was decreased by 0,5%.  
Find the interest rate used before 30 January 2014.  (2)
1.1.6 Calculate the VAT amount that is included in the monthly administration fee.  (3)
1.1.7 Explain the term home loan.  (2)
1.1.8 Choose ONE of the following statements that correctly explains why the interest amounts charged for February and March are different:  (2)

1. 1. The interest rate changed.
   2. Interest is charged on the daily outstanding balance.
   3. The amount of interest decreases monthly.    (2)

1.1.9 Due to a bank error the debit order was unpaid on 1 October 2013. The debit order was paid on 2 October 2013. The bank rectified the error by making an adjustment, as shown in the statement. 

1. 1. Calculate the adjustment amount. (2) 
   2. Hence, state whether this adjustment amount should be reflected as a debit or a credit.  (2)

1.1.10 Calculate the amount of interest due on 1 April 2014 to be shown on the next statement.  (3)
You may use the formula: 

Interest =  B × n × r       where
                     365 
B = balance on 1% of the previous month
n= the number of days in the month
r= the interest rate 

1.2 

Use the information in the table above and the graphs on ANSWER SHEET 1 to answer the questions that follow. 

1.2.1 Explain the term variable cost in this context.  (2)

1.2.2 Calculate the exact total cost of renting the Beach Hotel venue for 230 people. 
You may use the following formula: 
Total cost (in rand) = fixed cost + 230 x variable cost  (3)

1.2.3 Determine: 

1. 1. The cheapest venue if only 90 persons attend the event (2)
   2. The maximum number of people that can attend the event if the total cost of the venue is R15 000  (2)

1.2.4 Khumu sells the tickets for R150 each. 

1. 1. Draw the income graph from the sale of up to 200 tickets on the same grid as the total cost graphs on ANSWER SHEET 1. (4)
   2. Calculate the total profit to be made if she rents the Castle venue and pays for 250 people, but sells only 194 tickets.  (5)

[43]

QUESTION 2 
2.1 

Kataryna is planning a fashion show and intends using the school hall for the event. The hall has a stage and she plans to have a raised T-shaped platform, called a runway, erected in front of the stage, as shown in the diagrams below. 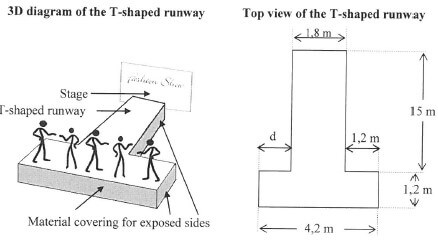   The SEVEN exposed rectangular sides of the T-shaped runway will be covered with material. The top of the runway will be carpeted. The total length of the runway is equal to į of the length of the hall.  [Adapted from www.jerichostage.com]

2.1.1 Calculate: 

1. 1. The missing value d (in mm) (3)
   2. The total length (in mm) of the exposed sides of the runway (3)
      The area (in m2) of the runway that needs to be covered with carpet (4)
      You may use the following formula:
      Area of a rectangle = length x width 
   3. The length (in m) of the hall  (3)

2.1.2 Harry, a British model, wants to know the measurement (in feet) of the front end of the runway.  (3)
Convert 4,2 m to feet, rounded off to one decimal place. 
NOTE: 1 foot = 0,3048 m 

2.2 

The organisers of the fashion show decide to hand out chocolates to the audience at the entrance. The pictures and diagrams below show the two different containers in which the chocolates will be packed.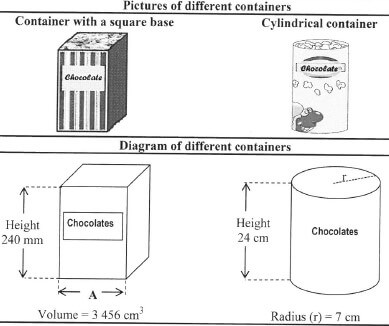

2.2.1 Determine A, the length (in cm) of ONE side of the square base. You may use the following formula:
Volume of box with square base = (side)² x height  (4)
2.2.2 The organisers of the fashion show want to use their own label around the outer curved side of the cylindrical container. The label will be 1 cm longer than the circumference of the circular base to allow for an overlap. 
Determine the total area (to the nearest cm²) of all the labels that will be required for 76 cylindrical containers.  (4)
You may use the following formula:
Area of one label (in cm²) = (1 + 2π × r ] × height
using π = 3,142 
2.2.3 Show, with calculations, that the volume of the cylindrical container is 238,99 cm³ more than the volume of the container with the square base.  (3)
You may use the following formula:
Volume of cylinder = π × r² x height
using π = 3,142 
2.2.4 State the most appropriate metric unit of measure for the mass of a container of chocolates.  (2)

[29]

QUESTION 3 
3.1 

 Use ANNEXURE B to answer the questions that follow. 

Related Items

3.1.1 Determine the total number of seats available in the middle block. (3)
3.1.2 Give the compass direction from seat E12 towards the stage. (2)
3.1.3 Rahim is seated exactly in the middle of a row in the middle block. The row he is seated in has an odd number of seats and is furthest from the stage. Name the row and seat number where he is seated. (3) 
3.1.4 Mali is seated at D14. She decides to go to the refreshment stand which is directly east of the lighting box. Give the directions for the route from her seat to the refreshment stand.  (4)
3.1.5 Determine the probability of randomly choosing a spectator to join the band on the stage if 871% of all the seats in the arena are occupied. (3) 
3.1.6 It is predicted that it is most unlikely that it will rain on the night of the performance. Choose ONE of the values below that best describes this probability: 
1,0           ½               0,0             40%               ³/₅           0,8                 20%     (2)

3.2 ANNEXURE C shows the assembly diagrams for a floor lamp. 
Use ANNEXURE C to answer the questions that follow. 

3.2.1 Refer to DIAGRAM 4. 

1. 1. Must the nut be screwed or unscrewed? (2)
   2. Give the direction in which the nut should be turned.  (2)

3.2.2 How many screws are needed to assemble the lamp shade?  (2)
3.2.3 Which diagram is associated with the instruction: 'Join the stand to the base."?  (2)
3.2.4 The total height of the floor lamp in the picture is 62 mm. 
Determine the actual height (in m) of the floor lamp if the scale of the diagram is 1 : 30. (3) 

[28] 

QUESTION 4 
4.1 

 Use TABLE 2 to answer the questions that follow. 

4.1.1 Determine the difference between the highest and lowest land-speed records that were set between 1950 and 2000.  (3)
4.1.2 Determine the number of riders that set new land-speed records from 1930 to 2010.  (2)
4.1.3 Identify the TWO years during which the land-speed record remained unbroken for the longest time AND also state the number of years the record remained unbroken.  (3)
4.1.4 Name the rider that held the land-speed record the most number of times AND also state how many times this rider held the record.  (3)
4.1.5 Determine the probability (as a percentage) of randomly selecting a land speed record in TABLE 2 that was set during the 219 century.  (3)

4.2 

Use TABLE 3 to answer the questions that follow. 

4.2.1 State why the data for the number of children is regarded as discrete data.  (2) 
4.2.2 Identify the age group where the majority of children did not attend any educational institution.  (2)
4.2.3 Give the year during which the age group 16 to 18 showed the best attendance.  (2)
4.2.4 Determine the missing value A.  (2)
4.2.5 Determine the missing value B, if the total number of children in that age group was 9 281 000 in 2008.  (3)
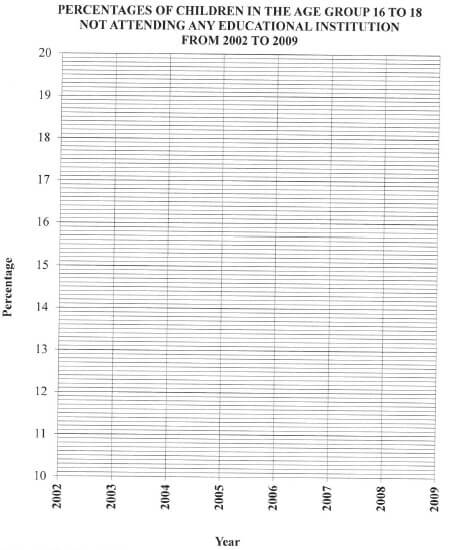
4.2.6 Draw a broken line graph on ANSWER SHEET 2 to represent the percentage of children in the age group 16 to 18 not attending any educational institution from 2002 to 2009  (5)

[30]

QUESTION 5 

Use ANNEXURE D to answer the questions that follow. 

5.1 Identify the country that has the strongest currency in comparison to the rand.   (2)
5.2 Calculate the price in rand that you will pay for a 2 { cola in the United States of America.   (2)
5.3 Determine the missing values: 

5.3.1  A   (2)
5.3.2 B, the value of ONE Indian rupee in rand   (2)

5.4 Determine the simplified ratio of the Singapore price of a Big Mac Burger to a 2 { cola.   (3)
5.5 Identify the TWO countries that have almost similar purchasing power.  (2)
5.6 Define the term median.   (2)
5.7 Use the prices in rand for a Big Mac Burger to do the following: 

5.7.1 Arrange the data in descending order   (2)
5.7.2 Calculate the mean price   (3)

[20]
TOTAL: 150  

ANSWER SHEET I
CENTRE NUMBER:  

EXAMINATION NUMBER:

QUESTION 1.2.4(a)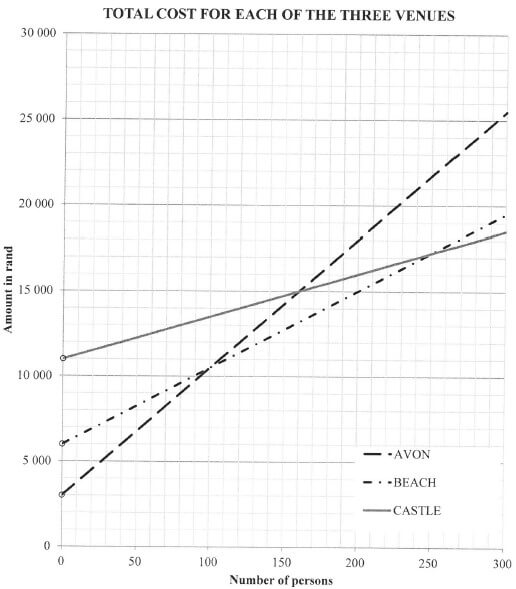 

ANSWER SHEET 2 
CENTRE NUMBER:  

EXAMINATION NUMBER:

QUESTION 4.2.6