MATHEMATICAL LITERACY PAPER 2 GRADE 12 QUESTIONS - NSC PAST PAPERS AND MEMOS SEPTEMBER 2020 PREPARATORY EXAMINATIONS
Share via Whatsapp Join our WhatsApp Group Join our Telegram GroupNATIONAL SENIOR CERTIFICATE
GRADE 12
SEPTEMBER 2020
MATHEMATICAL LITERACY P2
INSTRUCTIONS AND INFORMATION
Read the following instructions carefully before answering the questions.
- This question paper consists of FOUR questions. Answer ALL the questions.
- Use the ADDENDUM with ANNEXURES to answer the following questions:
ANNEXURE A for QUESTION 1.2,
ANNEXURE B for QUESTION 2.4 and
ANNEXURE C for QUESTION 3.1
Use ANSWER SHEET 1 for QUESTION 4.3.4 and hand it in with your ANSWER BOOK - Number the answers correctly according to the numbering system used in this question paper.
- An approved calculator (non-programmable and non-graphical) may be used, unless stated otherwise.
- Maps and diagrams are NOT necessarily drawn to scale, unless stated otherwise.
- Round off ALL final answers according to the context used, unless stated otherwise.
- Indicate units of measurement, where applicable.
- Start EACH question on a NEW page.
- Show ALL calculations clearly.
- Write neatly and legibly.
QUESTION 1
1.1
| Zulegha has a catering service and has an order to make eclairs. Below is the recipe that she is going to use to make the eclairs. Recipe for eclairs (1 Batch makes 25) Ingredients Preparation Method (25 minutes) 250 mℓ water 1. Boil water and oil in a saucepan. 125 mℓ oil 2. Remove saucepan from heat and add cake flour and mix 250 mℓ cake flour 3. Carry on mixing over low heat until paste forms a ball and sides of the saucepan are clean. 3 eggs 4. Place ball into a mixing bowl and allow cool. 5. Once cooled, beat in the eggs one at a time (blending well after each addition). 6. Spoon mixture onto a greased baking tray. Bake in a preheated oven at 180 °C for 45 minutes. You may use the following: 250 mℓ = 1 cup Flour: 5 mℓ → 3 g |
[Source: Boeka treats: A Compilation of Breads, Biscuits, Cakes and Pastries]
1.1.1 Determine how many cups of oil will be used if Zulegha wants to make 3 batches of eclairs. (3)
1.1.2 Determine the total cost for the eggs for one batch if Zulegha bought a dozen of eggs for R14,99. (4)
1.1.3 Zulegha’s friend, Najmie, in Qatar told her that she sets her oven temperature to 330°Fahrenheit to bake the eclairs. Use the following formula to show that her oven temperature is 26 °F lower than required.
°Fahrenheit = 1,8 × °Celsius + 32° (2)
1.1.4 Zulegha has a large order for 9 batches of eclairs that should be ready at 07:30 pm. She claims that if she starts at 09:15, the order will be ready. Verify, with the necessary calculations, whether her statement is valid or not. (6)
1.1.5 TABLE 1: QUANTITY OF INGREDIENTS AND AVAILABILITY IN SHOPS
| Ingredient | Amount needed | Availability in shops | Price inb shops | Cost per ingredient that is needed for the recipe |
| Cake flour | 250mℓ | 2.5 kg | R21,99 | A |
| Oil | 125mℓ | 2 litres | R35,99 | B |
| Eggs | 3 | Dozen | R14,99 | C |
Use TABLE 1 above to calculate missing values A, B and C and verify, with the necessary calculations, that the actual cost for the ingredients to make 9 batches, is more than R76,00. (9)
1.2
| Study the floorplan of Zulegha’s kitchen, ANNEXURE A, and answer the questions that follow. You may use the following: 1 foot = 0,3048 m 1 inch = 2,54 cm |
1.2.1 Calculate the length of the kitchen floor in metres. (6)
1.2.2 Identify the type of view that is represented by the image next to the floorplan and give a reason for your answer. (3)
1.2.3 Except for the living room in a house, the kitchen is seen as the most accessible room. Give TWO reasons why the kitchen is seen as the most accessible. (4)
[37]
QUESTION 2
2.1
| Mrs Lingham has a factory where coffee mugs are made and due to the high demand, she decided to open a second factory. Mrs Lingham did not have enough money to start the business and opted for a loan of R280 000 from a financial institution. |
2.1.1 Calculate the interest that she will pay on the Simple Interest Option. (3)
2.1.2 Advise Mrs Lingham as to which option to opt for if she wants to pay less interest. Show all calculations. (7)
2.2
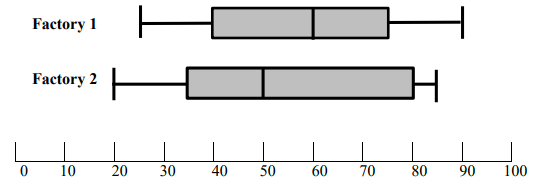
After the second factory was up and running, she compared the production of the two factories in one year. She used a box-and-whisker diagram. Production in thousands |
2.2.1 Explain which factory performed the worst in terms of production if the median and interquartiles ranges are compared. (8)
2.2.2 With reference to your answer in QUESTION 2.2.1, do you think it was a good idea of Mrs. Lingham to compare the production of the two factories? (2)
2.3
Mrs Lingham instructed her workers to pack the coffee mugs in boxes. The dimensions of coffee mugs and boxes are given below.
|
2.3.1 Show that the height of the coffee mug is 62,7% of the height of the box. (2)
2.3.2 Mrs Lingham claims that 66 mugs will fit in 10 boxes. Verify, showing all calculations, whether her statement is valid or not. (8)
2.4
| ANNEXURE B shows one of the routes that Mrs Lingham uses for her deliveries. Use ANNEXURE B to answer the following questions. |
2.4.1 Give the TWO general directions that the truck driver will follow from Port Elizabeth via Kokstad to Sani Lodge. (4)
2.4.2 Show that the probability of using a national road for the route from Port Elizabeth via Kokstad to Sani Pass for transporting a load is 25%. (2)
2.4.3 On the way from Port Elizabeth to Sani Lodge close to East London, the driver received a call that there was a protest action on the N2 in Mthatha. Advise the driver on which route (s)he should use to travel to avoid Mthatha. (3)
[39]
QUESTION 3
3.1
Yamkela, an employee, 64-years-old, receives a gross salary of R37 537,75 per month.
|
ANNEXURE C shows the 2019/2020 Tax Table. Use ANNEXURE C to answer the following questions.
3.1.1 Show how the initial tax of R35 253 in Tax bracket No. 2 was calculated. (2)
3.1.2 Calculate the total amount that Yamkela pays towards pension and donations for the year. (5)
3.1.3 Hence, calculate Yamkela’s annual taxable income. (3)
3.1.4 Verify, with the necessary calculations, that Yamkela’s tax that he pays per month is more than R6 850. (7)
3.1.5 Explain why people 75 years and older pay less tax than people younger than 75 years. (2)
3.1.6 The monthly gross salary of Yamkela increased by 6,4% in 2019. Calculate what his gross salary was in 2018. (3)
3.2
| Yamkela is also a rugby coach in his community. He is looking for an under-19 rugby team. He uses the following data that he collected from the schools’ database in his area for rugby players according to their ages. TABLE 2: AGES AND NUMBER OF BOYS
|
3.2.1 Determine the value of A (number of boys), if the data that Yamkela collected was for 90 boys. (3)
3.2.2 Determine the modal age for the data collected. (2)
3.2.3 Yamkela’s assistant coach stated that if you look at the data, the mean age is exactly 16 years. Verify, with the necessary calculations, whether his statement is valid or not. (4)
3.2.4 To qualify for the under-19 rugby team, the boys should be older than 14 years and younger than 19 years. Determine the probability that a boy who is randomly selected will make the team. Write your final answer as a decimal fraction to 3 decimal places. (3)
3.2.5 Yamkela’s assistant coach stated that age could not be the only qualifying factor to be selected for the under-19 rugby team. Support this statement with a reason. (2)
[36]
QUESTION 4
4.1
Study the following graph showing the exchange rate between the American Dollar($) and the South African Rand (R) from 11 January 2020 to 10 February 2020.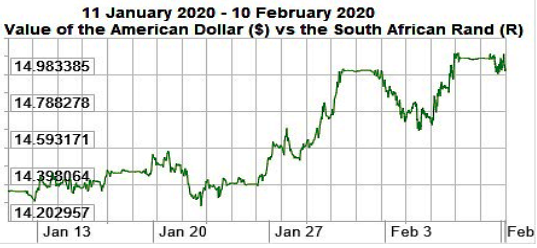 NOTE: The vertical axis represents the rand value. [Source: https://www.x-rates.com/] |
4.1.1 Identify, with a reason, the value of the South African Rand that was the strongest against the American Dollar. (2)
4.1.2 Zama states that he would have received exactly $100 more for his exchange when it was 14,983385 than when it was 14,398064 against the dollar.
NOTE: Zama’s exchange value is R40 830 and he must pay an exchange fee of 4,5% on the value.
Verify, with the necessary calculations, whether his statement is valid or not. (7)
4.1.3 Give TWO possible reasons why exchange rates fluctuate from time to time. (4)
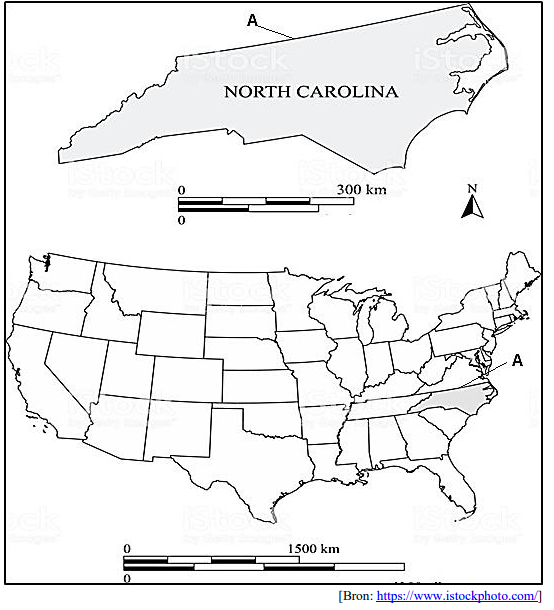
4.2 The following map shows the map of the United States of America as well as an extract of North Carolina. The shaded area on the map as well as the enlarged part refers to North Carolina.
4.2.1 Verify, with the necessary calculations, that the northern line of North Carolina, marked A, on both the map and the enlargement have different distances in kilometres. (7)
4.2.2 A family of four is going on a 15-day boat cruise from North Carolina to Los Angeles.
A package deal was given as follow:
- Traveller 1 and 2 pays $670,36 per person.
- Traveller 3 and 4 each pays 23,9% of the amount of travellers 1 and 2.
- An additional charge for tax and port duty is $188,64 per person.
Calculate the total amount that the family will spend on the boat cruise. (6)
4.3
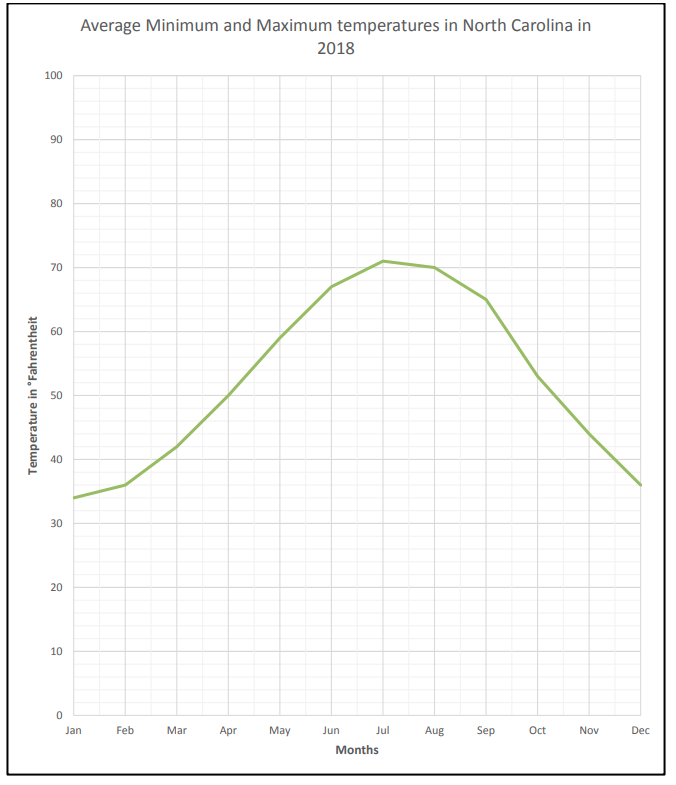
| The following average minimum and maximum temperatures in °Fahrenheit were recorded in North Carolina during 2018. TABLE 3: AVERAGE MINIMUM AND MAXIMUM TEMPERATURES OF NORTH CAROLINA IN 2018
|
4.3.1 Determine the value of A, the highest maximum temperature, if the range for the maximum data is 34 °F. (2)
4.3.2 Determine the probability of randomly selecting a month where the minimum temperature is between 36 °F and 60 °F. Write your final answer to the nearest percentage. (3)
4.3.3 A South African stated that the minimum temperature in North Carolina for January is lower than 0 °Celsius. Verify, with the necessary calculations, whether his statement is valid or not. (4)
4.3.4 A line graph with the minimum temperatures has already been drawn on ANSWER SHEET 1. Use the same set of axes to draw a line graph for the maximum temperatures. (3)
[38]
TOTAL: 150
ANSWER SHEET 1
QUESTION 4.3.4