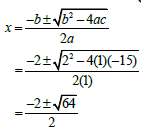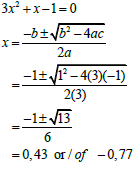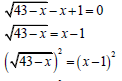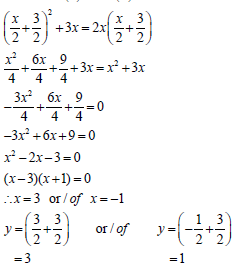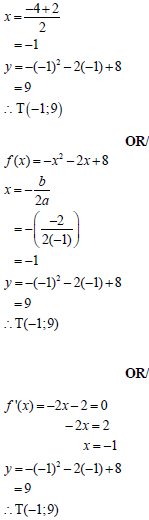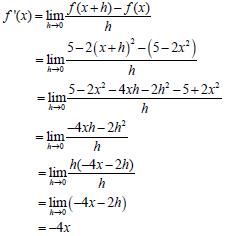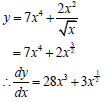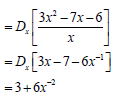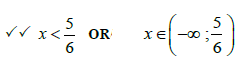Mathematics Paper 1 Memorandum - Grade 12 September 2021 Preparatory Exams

Thursday, 03 March 2022 06:32

Mathematics Paper 1 Memorandum - Grade 12 September 2021 Preparatory Exams

More in this category: « Mathematics Paper 1 Questions - Grade 12 September 2021 Preparatory Exams Mathematics Paper 2 Questions - Grade 12 September 2021 Preparatory Exams »

Share via Whatsapp Join our WhatsApp Group Join our Telegram Group

NOTE:

If a candidate answers a question TWICE, mark the FIRST attempt
Consistent accuracy applies in ALL aspects of the marking
If a candidate crossed out an attempt of a question and did not redo the question, mark the crossed-out
The mark for substitution is awarded for substitution into the correct

MEMORNDUM

QUESTION 1

1.1.1

x² + 2x -15 = 0
(x - 3)(x + 5) = 0
∴ x = 3 or / of x = -5

🗸 factors
🗸 x = 3 🗸 x = -5 (3)

🗸 substitution
🗸 x = 3 🗸 x = -5 (3)

1.1.2

Penalise 1 mark for incorrect rounding

🗸 substitution

🗸 x = 0, 43 🗸 x = -0, 77

(3)

1.1.3

x(x - 3) ≥ -2
x² - 3x + 2 ≥ 0
(x -1)(x - 2) ≥ 0
∴ x ≤ 1 or x ≥ 2

🗸 standard form

🗸 factorisation

🗸 x ≤ 1 or/of 🗸 x ≥ 2 (4)

1.1.4

43 - x = x² - 2x +1
x² - x - 42 = 0
(x - 7)(x + 6) = 0
∴ x = 7 or x # - 6

🗸 isolating the surd

🗸 squaring both sides

🗸 standard form

🗸 factorisation

🗸 selection (5)

1.2

2y - x = 3 (1)
y² + 3x = 2xy (2)

x = 2y - 3 (3)
Substitute(3)into(2)
y² + 3(2 y - 3) = 2 y(2 y - 3)
y² + 6 y - 9 - 4y² + 6 y = 0
-3y² +12 y - 9 = 0
y² - 4 y + 3 = 0
( y - 3)( y -1) = 0

∴ y = 3 or y = 1
x = 2(3) - 3 or x = 2(1) - 3
= 3 = -1

OR
2y - x = 3 (1)
y² + 3x = 2xy (2)
y = x + 3 (3)
2 2
Substitute (3) into (2)

🗸 x = 2y - 3

🗸 substitution

🗸 standard form

🗸 factorisation

🗸 y-values

🗸 y = x + 3
2 2

🗸 substitution

🗸 standard form

🗸 factorisation

🗸 x-values

🗸 y-values (5)

1.3

For non-real roots:
Δ < 0
p(6 - p) - 9 < 0
- p² + 6p - 9 < 0
p² - 6p + 9 > 0
(p - 3)² > 0
∴ p∈ but p # 3

For non-real roots:
Δ< 0
p(6 - p) - 9 < 0
- p² + 6p - 9 < 0
(3 - p)( p - 3) < 0
∴ p∈ but p # 3

🗸 Δ< 0

🗸 standard form

🗸 factorisation

🗸 answer

🗸 Δ< 0

🗸 standard form

🗸 factorisation

🗸 answer (4)

[24]

QUESTION 2

2.1.1	8	🗸 8 (1)
2.1.2	2a = 4 ∴ a = 2 3a + b = 4 3(2) + b = 0 ∴ b = - 6 a + b + c = -16 2 - 6 + c = -16 ∴ c = -12 T_n = 2n² - 6n -12	🗸 a = 2 🗸 b = -6 🗸 c = -12 🗸 T_n = 2n² - 6n -12 (4)
2.1.3	T₃₈= 2(38)² - 6(38) -12 = 2648	🗸 substitution 🗸 answer (2)

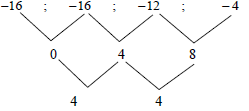
2.1.4	General term for first differences: T_n = 4n - 4 400 = 4n - 4 ∴ n = 101 T_n(linear) = (T_n+1 - T_n )_(quadratic)∴ n = 101 and n +1 = 102 The termsare :101 and 102 OR 2(n +1)² - 6(n +1) -12 - (2n² - 6n -12) = 400 2n² + 4n + 2 - 6n - 6 -12 - 2n² + 6n +12 = 400 4n - 4 = 400 4n = 404 ∴ n = 101 ∴ Between T₁₀₁ and T₁₀₂ OR Trialand error T₁₀₂ = 2(102)² - 6(102) -12 = 20184 T₁₀₁ = 2(101)2 - 6(101) -12 = 19784 Difference: 400 ∴ BetweenT₁₀₁ and T₁₀₂	🗸 T_n = 4n - 4 🗸 T_n = 400 🗸 answer OR 🗸 4n - 4 = 400 🗸 🗸 answer OR 🗸subst. for T₁₀₁ and T₁₀₂ 🗸 400 🗸 answer (3)
2.2.1	T_n = a + (n -1)d 89 = 2 + (n -1)(3) 3n -1 = 89 3n = 90 n = 30	🗸 substitution 🗸 answer (2)
2.2.2		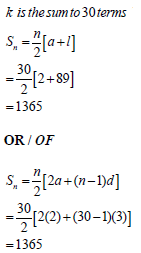🗸 Sum formula 🗸 substitution 🗸 answer OR 🗸 Sum formula 🗸 substitution 🗸 answer (3)
		[15]

QUESTION 3

3.1	T₉ = ar⁸ = 768 T₁₃ = ar¹² = 12 288 ar¹² = 12 288 ar⁸ 768 ∴ r⁴ = 16 r =± 2 a = 768 (±2)⁸= 3	🗸ar¹² = 12 288 ar⁸ 768 🗸 r =± 2 🗸 value of a	(3)
3.2.1	S₂ = 54 - 24 19 19 = 30 19	🗸 answer	(1)
3.2.2	T₁ + T₂ = 30 19 a + ar = 30 19 a(1+ r) = 30 19 a = 30 19(1+ r)	🗸 a + ar = 30 19	(1)
3.2.3	S_∞ = a = 54 1- r 19 ∴ a = 54(1- r) 19 a = 30 ................. from (3.2.2) 19(1+ r) ∴ 30 = 54(1- r) 19(1+ r) 19 (1- r)(1+ r) = 30 54 1- r² = ⁵/₉ r² = ⁴/₉∴ r = ²/₃	🗸 a = 54(1- r) 19 🗸 equating 🗸r² = ⁴/₉ 🗸 answer	(4)
		[9]

QUESTION 4


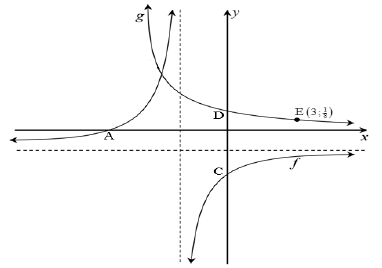4.1	D(0 ; 1)	🗸 (0 ; 1)	(1)
4.2	x = -2 ; y = -1	🗸 x = -2 🗸 y = -1	(2)
4.3	𝑥 ∈ R but 𝑥 # −2	🗸 𝑥 ∈ R 🗸𝑥 # −2	(2)
4.4	g(x) = b^x 8 = b^-38 = 1 b³ b³ = 1 8 ∴ b = 1 2	🗸 substitution 🗸 answer	(2)
4.5	y = -3 -1 x + 2 0 = -3 -1 x + 2 1 = -3 x + 2 x + 2 = -3 x = -5 ∴ A(-5; 0) y = -3 -1 0 + 2 =- 5 2 ∴ C (0; - ⁵/₂ )	🗸 substitution y = 0 🗸 x = -5 🗸 y =- 5 2	(3)
4.6	∴ y = log _½ x OR y = 2^-x∴ x = 2- y y = -log₂ x	🗸 🗸 y = log _½ x OR 🗸 x = 2- y 🗸 y = -log2 x	(2) (2)
4.7
4.7.1	-5 < x < -2 OR x ∈(-5 ; - 2)	🗸🗸 answer (A)	(2)
4.7.2	0 < x ≤ ¹/₈ OR x ∈(0 ; ¹/₈]	🗸🗸 answer (A)	(2)
			[16]

QUESTION 5


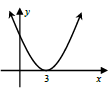
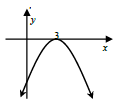
5.1	-x² - 2x + 8 = 0 x² + 2x - 8 = 0 (x + 4)(x - 2) = 0 ∴ x = -4 or x = 2 ∴ R(-4; 0) and S(2 ; 0) ∴ RS = 6 units	🗸 f (x) = 0 🗸 factorisation 🗸 values of x 🗸 answer	(4)
5.2		🗸 method 🗸 x = -1 🗸 y = 9 OR 🗸 - b 2a 🗸 x = -1 🗸 y = 9 OR 🗸 -2x - 2 = 0 🗸 x = -1 🗸 y = 9	(3)
5.3.1	f (x) = -x² - 2x + 8 f '(x) = -2x - 2 ∴ -2x - 2 = 2 ∴ x = -2 ∴ y = - (-2)² - 2(-2) + 8 = 8 ∴ W (-2;8)	🗸 f / (x) 🗸 f / (x) = 2 🗸 x = -2 🗸 y = 8	(4)
5.3.2	g(x) = mx + c m = - ½ (⊥ lines ) c = 8 ∴ y = - ½ x + 8	🗸 gradient 🗸 equation	(2)
5.4	f (x) = -x² - 2x + 8 h(x) = - f (x -1) = - [-(x -1)² - 2(x -1) + 8] = - [-(x² - 2x +1) - 2x + 2 + 8] = - [-x² + 2x -1- 2x + 2 + 8] = x² - 9 OR h(x) = (x + 3)(x - 3) = x² - 9 OR New turning point = (0 ; –9) y = x² - 9	🗸 - f (x -1) 🗸 substitution 🗸 simplifying 🗸 equation OR 🗸🗸 roots 3 and –3 🗸 +( x + 3)(x – 3) 🗸 equation OR 🗸 (0 ; 🗸🗸–9) 🗸 equation	(4)
			17]

QUESTION 6

6.1		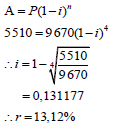🗸 subst. into correct formula 🗸 simplification 🗸 answer (3)
6.2		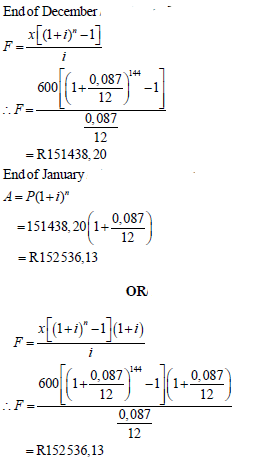🗸 n = 144 🗸 subst. into correct formula 🗸 adding final month’s interest 🗸 answer OR 🗸 n = 144 🗸 subst. into correct formula 🗸 adding final month’s interest 🗸 answer (4)
6.3.1		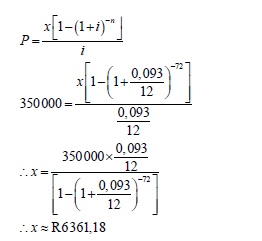🗸 i = 0, 093 and n = 72 12 🗸 substitution into correct formula 🗸 answer (3)
6.3.2		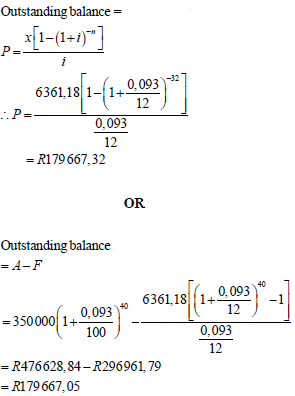🗸 i = 0,093 and/en n = 32 12 🗸 subst. into correct formula 🗸 P = 179 667, 32 OR 🗸 i = 0,093 and/en n = 40 12 🗸 subst. Into correct formula 🗸 P = 179 667, 32 (3)
6.3.3		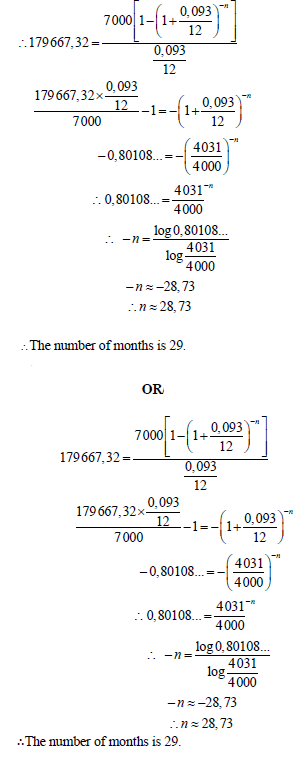🗸 subst. into correct formula 🗸 correct use of logs 🗸 = 28,73 🗸 n = 29 months OR 🗸 subst. into correct formula 🗸 correct use of logs 🗸 = 28,73 🗸 n = 29 months (4)
		[17]

QUESTION 7

7.1		🗸 substitution 🗸 expansion 🗸 simplification 🗸 notation and lim (-4x - 2h) h -> 0 🗸 answer (5)
7.2.1		🗸 2x^½ 🗸 28x³ 🗸 3x^½ (3)
7.2.2		🗸 3x - 7 🗸 -6x^-1🗸 3 and differentiating constant 🗸 +6x^-2(4)
		[12]

QUESTION 8

8.1.1	f (x) = 2(x - x₁ )(x - x₂ )(x - x₃) = 2 ( x +1)( x - ½)( x - 3) = ( x +1)(2x -1)( x - 3) = ( x +1)(2x² - 7x + 3) = 2x³ - 7x² + 3x + 2x² - 7x + 3 = 2x³ - 5x² - 4x + 3 f (x) = 2x³ + bx² + cx + d ∴ b = -5, c = - 4 , d = 3	🗸🗸 f (x) = 2( x +1)( x - ½ )( x - 3) OR 🗸🗸 f (x) = ( x +1)(2x -1)( x - 3) 🗸 exapnsion 🗸 simplifying (4)
8.1.2	f '(x) = 6x² -10x - 4 0 = 6x² -10x - 4 ∴ 3x² - 5x - 2 = 0 (3x +1)( x - 2) = 0 ∴ x = - ¹/₃ or x = 2 ∴ N is at f (2) f (2) = 2 (2)³ - 5(2)² - 4 (2) + 3 = -9 ∴ N (2 ; - 9)	🗸 f '( x) = 6x² -10x - 4 = 0 🗸 factorisation 🗸 chosing : x = 2 🗸 y = -9	(4)
8.1.3 (a)	- ¹/₃ < x < 2	🗸🗸 answer	(2)
8.1.3 (b)		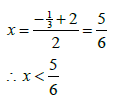🗸 f ''(x) =12x -10 🗸 f ''(x) < 0 🗸 answer OR 🗸 x = ⁵/₆	(3)
8.2		🗸 f (0) = 0 🗸 (m ; 0) 🗸 shape	(3)
		[16]

QUESTION 9

9.1	A = (½ ×15x × 8x × 2) + (15xy) + (8xy) + (17xy) 5760 = 120x² + 40xy ∴y = 5760 -120x² 40x	🗸 total surface area 🗸 5760 = 120x² + 40xy	(2)
9.2	V = (½b.h) × H V = ½ × 15x × 8x × y = ½ × 15x × 8x × 5760 -120x² 40x = 60x (144 - 3x²) = 8640x -180x³	🗸 substitution into V 🗸 substituing for y	(2)
9.3	V '(x) = 8640 - 540x²V '(x) = 0 ∴ 8640 - 540x² = 0 8640 = 540x²x² = 16 ∴ x = 4	🗸 V '(x) = 8640 - 540x²🗸 V '(x) = 0 🗸 simplification 🗸 answer	(4)
		[8]

QUESTION 10

10.1.1	P(B) = 1- P(not) = 1- 0, 45 = 0, 55	🗸 0,55 (1)
10.1.2	P(A and/en B) = P(A)×P(B) = 0, 2 × 0, 55 = 0,11 P(A or B) = P(A) + P(B) - P(A and B) = 0, 2 + 0, 55 - 0,11 = 0, 64 or ¹⁶/₂₅	🗸 P(A)×P(B) 🗸 substitution 🗸 answer (3)
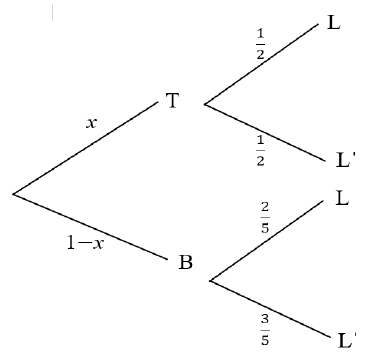
10.2	P(late) = ¹/₂ x + ³/₅(1- x) ¹/₂ x + ³/₅ (1- x) = ⁸/₁₅ 15x +18(1- x) = 16 15x +18 -18x = 16 - 3x = -2 x = ²/₃	🗸 ¹/₂ x + ³/₅(1- x) 🗸 equating 🗸 substitution 🗸 answer (4)
		[8]

QUESTION 11

11.1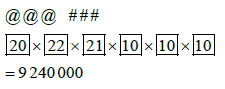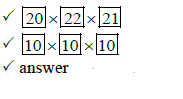
11.2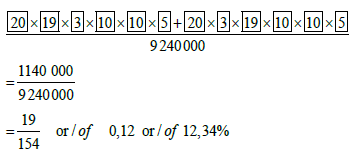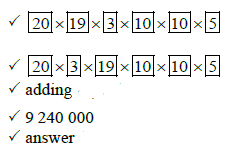
		[8]

TOTAL: 150

Last modified on Thursday, 17 March 2022 06:47

Published in Grade 12 Preparatory Exam Papers and Memos September 2021

Mathematics Paper 1 Memorandum - Grade 12 September 2021 Preparatory Exams

MEMORNDUM

Related items