PHYSICAL SCIENCES: PHYSICS (PAPER 1)
GRADE 12
NATIONAL SENIOR CERTIFICATE
MEMORANDUM
NOVEMBER 2020

QUESTION 1
1.1 B ✓✓(2)
1.2 D ✓✓ (2)
1.3 C✓✓ (2)
1.4 C ✓✓ (2)
1.5 C ✓✓ (2)
1.6 A ✓✓ (2)
1.7 A ✓✓ (2)
1.8 D ✓✓ (2)
1.9 A ✓✓ (2)
1.10 B ✓✓ (2)
[20]

QUESTION 2
2.1 Marking criteria
If any of the underlined key words/phrases in the correct context are omitted: - 1 mark per word/phrase.
The perpendicular force exerted by a surface on an object in contact with the surface. ✓✓ (2)

2.2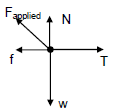

Accepted symbols
N - FN/Normal/Normal force/173,5N /Normaal
f -  Ff /fk /frictional force/kinetic frictional force/5 N
w - Fg /mg/Weight/FEarth on block/Fw/Gravitational force/196 N
T - Tension/FT
Fapplied  - F/Applied force/35 N/ FA

Notes

• Mark is awarded for label and arrow.
• Do not penalise for length of arrows.
• Deduct 1 mark for any additional force.
• If all forces are correctly drawn and labelled, but no arrows, deduct 1 mark. (5)

2.3 For the 20 kg:
Fnet = ma
T – f – FAx = ma
T – 5 – 35 cos40° = 0 ✓
T = 31,81 N
For m:
Fnet = ma
mg – T = ma
m(9,8) – 31,81 ✓ = 0
m = 3,25 kg ✓
Marking criteria

• Formula for 20 kg or m kg 20 kg of m kg / Fnet = ma ✓
• Substitution of zero into either formula 
• All substitutions into Fnet for 20 kg as shown 
• Substitution of value of T in eqn for m
• Final answer/finale antwoord: 3,25 kg ✓ (5)

2.4.1 Decreases ✓ (1)
2.4.2 POSITIVE MARKING FROM QUESTION 2.3
Moving to the right
Velocity decreases
Accelerates/Net force to left✓✓
OR
As the tension force decreases, the net force/acceleration acts in the opposite direction of motion /to the left.✓✓
Moving to the left
Velocity increases ✓
Accelerates/Net force to left ✓✓(3)
[16]

QUESTION 3
3.1 (Motion of an object) under the influence of gravity (weight) only. (2 or 0)
OR
(Motion in which) the only force acting on the object is gravity (weight). (2)

3.2.1 Δt = 0,67 – 0,64 = 0,03 s (2)

3.2.2 OPTION 1
Δt =(1,90 - 0,67)
              2
= 0,62 s (0,615 s)

OPTION 2
Δx = viΔt + ½ aΔt²
(-1,85) = 0 + ½ (-9,8)Δt²
Δt = 0,61 s  (0,6145 s) (2)

OPTION 3
Δt =(1,90 + 0,67)
               2
= 1,285 s
Δt = 1,285 – 0,67 ✓
= 0,62 s (0,615 s)

OPTION 4
vf2 = vi2 + 2aΔx
0 = vi2 + 2(-9,8)(1,85)
vi = 6,02 m·s-1
vf = vi + aΔt
0 = 6,02 + (-9,8)Δt ✓
Δt = 0,61 s✓

3.2.3 POSITIVE MARKING FROM QUESTION 3.2.2
Marking Criteria

• Any appropriate formula
• Correct substitution
• Final answer: 5,94 to 6,08 m·s-1 ✓

OPTION 1
Upwards positive
vf = vi + aΔt ✓
0 = vi + (-9,8)(0,62) ✓
vi = 6,08 m·s-1 (6,076 m·s-1)
Downwards positive
Δy = viΔt + ½ aΔt2 ✓
1,85 = vi (0,62) + ½ (9,8) (0,62)2 ✓
vi = -6,02
vi = 6,02 m·s-1 (6,022 m·s-1) ✓

OPTION 2
Upwards positive
Δy = viΔt + ½ aΔt²✓
1,85 = vi (0,62) + ½ (-9,8) (0,62⁾²
vi = 6,02 m·s-1 (6,022 m·s-1 ) ✓
Downwards positive/Afwaarts positief
vf = vi + aΔt ✓
0 = vi + (9,8)(0,62) ✓
vi = -6,08
6,08 m·s-1 (6,076 m·s-1 )✓

OPTION 3
Motion from top to bottom
Downwards positive
vf2 = vi² + 2aΔy
vf2 = 0 + 2(9,8)(1,85)✓
vf = 6,02 m·s-1 ✓
initial velocity =6,02 m·s-1
Upwards positive
vf2 = vi² + 2aΔy
2 = 0 + 2(-9,8)(-1,85) ✓
vf = 6,02 m·s-1✓
initial velocity = 6,02 m·s-1
Motion from bottom to top
Downwards positive
vf2 = vi² + 2aΔy
0² = vi2 + 2(9,8)(-1,85)✓
vi = 6,02 m·s-1✓
Upwards positive
vf2 = vi² + 2aΔy
0 = vi2 + 2(-9,8)(1,85)✓
vi = 6,02 m·s-1 ✓

OPTION 4
Upwards positive
Δy = viΔt + ½ aΔt² ✓
0 = vi(1,23) + ½ (-9,8)(1,23)2✓
vi = 6,03 m·s-1 ✓
Downwards positive
Δy = viΔt + ½ aΔt2 ✓
0 = vi(1,23) + ½ (9,8)(1,23)2 ✓
vi = - 6,03 m·s-1
speed = 6,03 m·s-1✓

OPTION 5
Δy = (vf + vi)  Δt
             2
1,85 = (0 + vi) (0,62)
                2
vi = 5,97 m·s-1✓✓

OPTION 6
FnetΔt = mΔv
FnetΔt = m(vf – vi)
m(9,8)(0,62) = m(0 – vi) ✓
vi = 6,08 m·s-1 ✓

OPTION 7
(Ep + Ek)floor = (Ep + Ek)top✓
(mgh + ½ mv2)floor = (mgh + ½ mv2)top
0 + ½ v2 = (9,8)(1,85) + 0✓
v = 6,02 m·s-1✓(3)

3.2.4 OPTION 1, 2, 3, 4: Marking criteria
Calculate initial velocity:

• Appropriate formula
• Substitution

Calculate Δt:

• Appropriate formula
• Substitution
• 1,97 s + Δt ✓
• Fin answer: 2,95 – 2,97 s ✓

Calculate initial velocity:
OPTION 1
Downwards positive
vf² = vi² + 2aΔy
0 = vi2 + 2(9,8)(-1,2)✓
vi = - 4,85 m·s-1
Upwards positive
vf² = vi² + 2aΔy
0 = vi2 + 2(-9,8)(1,2)✓
vi = 4,85 m·s-1

OPTION 2
(Emech)top = (Emech)bot
(Ep +Ek)top = (Ep +Ek)Bot
(mgh + ½mv2)top = (mgh + ½mv2)Bot
(9,8)(1,2) + 0 = 0 + (½)v2 ✓
vi = 4,85 m·s^-1

OPTION 3
Wnc = ΔEp + ΔEk
0 = (0 – mgh) + ½m(v²_f - v²_i)
0 = -(9,8)(1,2) + ½vi²
vi = 4,85 m·s-1 upwards

OPTION 4
Wnet = ΔEk
wΔxcos180° = ½m(v²_f - v²_i)
(9,8)(1,2)cos180° = ½vi²✓
vi = - 4,85 m·s^-1

OPTION 5
Downwards positive
Δy = viΔt + ½ aΔt2 ✓
1,2 ✓= 0 + ½(9,8) Δt2✓
Δt = 0,49 s
t = 1,97 + ✓ 2(0,49) ✓
= 2,96 s ✓
Upwards positive
Δy = viΔt + ½ aΔt2 ✓
-1,2 ✓= 0 + ½(-9,8)Δt2✓
Δt = 0,49 s
t = 1,97 + ✓ 2(0,49)✓
= 2,96 

Calculate time Δt
Upwards positive
Δy = viΔt + ½ aΔt2✓
1,2 = (4,85)Δt + ½(-9,8)Δt2 ✓
Δt = 0,4898 s / 0,5 s
t = 1,97 + 2(0,4898)✓
= 2,95 s / 2,97 s✓
OR
Δy = viΔt + ½ aΔt2 ✓
0 = (4,85)Δt + ½(-9,8)Δt2 ✓
Δt = 0,9898 s (or Δt = 0)
t = 1,97 + 0,9898 ✓ = 2,96 s ✓
Downwards positive
Δy = viΔt + ½aΔt2✓
1,2 = (-4,85)Δt + ½(9,8)Δt2 ✓
Δt = 0,4898 s / 0,5 s
t = 1,97 + 2(0,4898)✓
= 2,95 s / 2,97 s ✓
OR
Δy = viΔt + ½aΔt2✓
0 = (4,85)Δt + ½(9,8)Δt2✓
Δt = 0,9898 s (or Δt = 0)
t = 1,97 + 0,9898✓ = 2,96 s✓
OR
vf = vi + aΔt ✓
-4,85 = 4,85 + (-9,8)Δt ✓
Δt = 0,9898 s
Δt = 1,97 + 0,9898 ✓= 2,96 s✓
OR
Upwards positive
vf = vi + aΔt✓
0 = 4,85 + (-9,8)Δt ✓
Δt = 0,4949 s
Δt = 1,97 + (2)(0,4949)✓
= 2,96 s✓✓
OR
Δy = (vi + vf) Δt
              2
1,2 = (0 + 4.85) Δt✓Δt = 0,4948s
               2
Δt total = 2(0,4948) = 0,99 s
Δt = 1,97 + 0,99✓= 2,96 s ✓

OPTION 5: Marking criteria

• Formula ✓
• Substitution Δy = 1,2✓
• Substitution 0 + ½(9,8) Δt2
• 1,97 s + ✓
• 2 Δt ✓
• Final answer: 2,95 - 2,97 s ✓(6)

[15]

QUESTION 4
4.1 (Linear) momentum (of an object) is the product of mass and velocity.✓✓
(2 or 0) (2)

4.2.1 OPTION 1
East as positive
Σpi = Σpf
mpvpi + mQvQi = mpvpf + mQvQf
(0,16)(10) + (0,2)(-15) ✓= (0,16)(-5) +(0,2)vQf ✓
vQf = -3 m∙s-1
vQf = 3 m∙s-1 ✓ west ✓

OPTION 2
West as positive
Σpi = Σpf
mpvpi + mQvQi = mpvpf + mQvQf
(0,16)(-10) + (0,2)(15) ✓= (0,16)(5) +(0,2)QNf ✓
vQf = 3 m∙s-1 ✓ west ✓

OPTION 3
Δpp = -ΔpQ✓
(0,16)(-5 – 10) ✓= -(0,2)(v – (-15))✓
v = -3 m·s-1
= 3 m·s-1 ✓west ✓✓✓ (5)
Any one

4.2.2 For ball:
West as negative
Impulse = Δp
FnetΔt = Δp
Δp = m(vPf – vPi)
= 0,16(-5 – 10)✓
= - 2,4
2,4 N∙s ✓ (2,4 kg∙m∙s-1)
OR
West as positive /Wes as positief
Impulse = Δp
FnetΔt = Δp
= m(vPf – vPi)
= 0,16(5 – (-10)) ✓
= 2,4 N·s 

POSITIVE MARKING FROM QUESTION 4.2.1 (3)
For ball:
West as negative
Impulse = Δp
FnetΔt = Δp
= m(vQf – vQi)
= 0,2[-3 – (-15)]✓
= 2,4 N∙s ✓ (2,4 kg∙m∙s-1)
OR
West as positive
Impulse = Δp
FnetΔt = Δp
= m(vQf – vQi)
= 0,16(3 – (15)) ✓
= - 2,4 N·s
2,4 N∙s✓ (2,4 kg∙m∙s-1)
[10]

QUESTION 5
5.1 Marking criteria
If any of the underlined key words/phrases in the correct context are omitted:
1 mark per word/phrase. However, IF: The word “work” is omitted 0 marks

A force is non-conservative if the work it does on an object (which is moving between two points) depends on the path taken.✓✓
OR
A force is non-conservative if the work it does on an object depends on the path taken. ✓✓
OR
A force is non-conservative if the work it does in moving an object around a closed path is non-zero. ✓✓ (2)

5.2 K = ½ mv2 /Ek = ½ mv2
ΔK = Kf - Ki
K = ½mvf² - ½mvi² 
= ½m(vf²- vi²)
=½(200)(2² - 4²)✓
K = - 1 200 J✓ (3)
Any one

5.3 POSITIVE MARKING FROM QUESTION 5.2.
Marking criteria

• Appropriate formula
• Substitution into appropriate formula together with -3,40 × 10³ ✓✓
• Final answer: 8,88 m✓

OPTION 1
Wnc = ✓K +✓U
Wnc = ½ mvf² - ½ mvi² + mghf - mghi
= ½ m (vf²- vi²) + mg(hf - hi)
-3,40 × 10³ ✓= -1 200 + 200(9,8)(hf - 10) ✓
h = 8,88 m ✓ (8,87765 m)

OPTION 2
E(mech/meg)A + Wf = E(mech)B
(Ep +Ek)A + Wf = (Ep +Ek)_B
(mgh + ½mv²) A + Wf = (mgh + ½mv²)_B
200(9,8)(10) + ½(200)(4²) - 3,40 × 10³ = 200(9,8)(h) + ½(200)(2)² 
h = 8,88 m ✓ (8,87755)

OPTION 3
Wnet = ✓K
Wf + Ww = ½mvf² - ½mvi²
Wf – ΔEp = ½mvf² - ½mvi²
Wf - mg(hf - hi) = ½m(vf²- vi²)
-3,40 × 10³ - 200(9,8)(h-10) = -1 200 
h = 8,88 m ✓ (8,87755 m) (4)

5.4 OPTION 1 AND 2: Marking criteria

• Appropriate formula
• Work done by friction
• Substitution of (200)(9,8)(13,12)
• Appropriate formula
• Substitution into power formula
• Final answer: 1 814,35 W

OPTION 1
Wnc = ✓K + ✓U
Wengine + Wf = ½mvf² - ½ mvi² + mghf - mghi
= ½m(vf²- vi²) + mg(hf - hi)
W_engine+ (50)(15)(2)cos180° = 0 + 200(9,8)(22 – 8,88)
W_engine = 27 215,20 J
P_engine =W_engine
                   t
=27 215,20
       15
= 1 814,35 W
Any one

OPTION 2
Wnet = K
WN + W_engine + Ww + Wf = 0
WN + W_engine - ΔEp + Wf = 0
0 + W_engine - (200)(9,8)✓(13,12) + (50)(2)(15)cos180° ✓✓= 0
W_engine= 27 215,20 J
OR
Wnet = ✓K✓✓
WN + W_engine + Ww|| + Wf = 0
WN + W_engine +mgsinθΔxcos180o + Wf = 0
0 + W_engine - (200)(9,8)(13,12)Δx(-1)+ (50)(2)(15)cos180° = 0
                                         Δx
W_engine= 27 215,20 J
P_engine = W_engine
                  Δt
=25 215,20
       15
= 1 814,35 W

OPTION 3: Marking criteria

• Appropriate formula
• Substitution of - 50 ✓✓
• Substitution of (-200)(9,8)(0,4373) or (-200)(9,8)(0,44)✓
• Appropriate formula
• Substitution into P_ave = Fv_ave
• Final answer: 1 814,35 W - 1 824,8 W

OPTION 3
Fnet = ma
F_engine + F_friction + Fg// = 0
F_engine + (-50) + (-200)(9,8)(0,4373) = 0
F_engine = 906,52 N (906,52 – 912,4)
P_ave = Fv_ave
P_ave = (908,52)(2)
= 1 813,04 W (1 824,8 W)

sin = ^h/_Δt
=13.12
  2(15)
= 0.4373

OR
W = FengineΔxcosθ
= (906,52)(30)cos0o
= 27 195,6 J (27 372 W)
P = W = 27195,6 = 1 813,04 W ✓✓(1 824,8 W)
      Δt        15
(5)
[14]

QUESTION 6
6.1 Marking criteria
If any of the underlined key words/phrases in the correct context are omitted:
- 1 mark per word/phrase.
The change in frequency✓ (or pitch) (of the sound) detected by a listener because the source and the listener have different velocities relative to the medium of propagation.✓
OR
An (apparent) change in (observed/detected) frequency (pitch), as a result of the relative motion between a source and an observer (listener). (2)

6.2 Towards (1)

6.3 f_L = v ± v_Lfs  OR fL =     v       OR fL =    v     fs
             v ± v_S                      v - vs                 v + v_s
3148 = 340 + 0 fs                           2073 = 340 - 0 fs
            340 - v_s                                          340 + v_s
3148(340 - v_s) = 2073(340 + v_s) 
    340 + 0                   340 - 0
vs = 70 m·s-1✓ (69,95 - 70,16 m·s-1) (6)

6.4 POSITIVE MARKING FROM QUESTION 6.3
OPTION 1
Δt = Δx 
         v
Δt = 350
         70
Δt =5 s ✓

OPTION 2
Δx = viΔt + ½ aΔt²
350 = 70Δt + 0 ✓
Δt = 5 s ✓

OPTION 3
Δx =(v_i + v_f) Δt 
            2
350 =(70 + 70) Δt 
               2
Δt = 5 s ✓ (2)
[11]

QUESTION 7
7.1
n = Q 
      e
=  (-)4 x 10 ^-6
  (-)1,6 x 10^-19
= 2,5 x 10¹³ (3)

7.2 Electrostatic force on B due to A:
F_AB = kQ₁Q₂
              r²
= [9 x 10⁹(4 x 10^-6)(3 x 10^-6)]
                    0.2²
= 2,7 N (3)
Ignore negative signs

7.3 Marking criteria
If any of the underlined key words/phrases in the correct context are omitted:
- 1 mark per word/phrase.
Electric field is a region (in space) where (in which) an (electric) charge experiences a (electric) force. ✓✓ (2)

7.4 Marking criteria

• Appropriate formula
• Correct substitution for A and B
• Subtraction of electric fields
• Final answer: 2,3 x10⁶ N∙C-1

OPTION 1
Electric field at M due to : -4 x10^-6 C
EAM = k Q 
               r²
=9 x 10⁹(4 x 10^-6)
                 (0.3)²
= 4,0 x 10⁵ N∙C^-1 (to left )
Electric field at M due to : +3 x 10^-6 C,
EBM = k Q 
               r²
9 x 10⁹(3 x10^-6)
              (0,1)²
= 2,7 x10⁶ N∙C^-1 (to right)

Net electric field at M
Enet = EBM + EAM
= 4,0 x10⁵ – 2,7 x 10⁶ ✓
= 2,3 x10⁶ N∙C^-1 (right)
OR
Net electric field at M
Enet = EBM + EAM
= -4,0 x 10⁵ + 2,7 x 10⁶ 
= - 2,3 x 10⁶ N∙C-1
= 2,3 x 10⁶ N∙C-1 (right) (5)

OPTION 2
FAM = kQ₁Q₂  = (9 x 10⁹)(4 x 10^-6)Q = 4 x 10⁵Q N
               r²                  (0,3)²
FBM = kQ₁Q₂  = (9 x 10⁹)(4 x 10^-6)Q = = 2,7 x 10⁶Q N
               r²                  (0,1)²
F_net = 2,7 x 10⁶Q + (-4 x 10⁵Q) = 2,3 x 10⁶Q
E = F  = 2,3 x 10⁶Q = 2,3 x 10⁶ N·C-1 (right)
      q            Q

7.5 Positive (1)

7.6 POSITIVE MARKING FROM 7.2
Marking criteria

• Correct substitution into Pythagoras’s equation
• Correct substitution into Coulomb’s Law
• Correct answer (3)

(Fnet)² = (FAD)2 + (FAB)²
(7,69)² = (FAD)2 + (2,7)² ✓
FAD = 7,2 N
FAD = kQ₁Q₂
              r²
7,2 = (9 x10⁹)(4 x10^-6)Q
                 (0,15)²
Q_D = 4,5 x 10^-6 C

OR
FAD = kQ₁Q₂
              r²
= 9 x10⁹(4 x10^-6)Q
           0,15²
= 1,6 x 10⁶Q
Fnet = √F_AB² + F_AD² OR Fnet² = F_AB² + F_AD²
7,69 =√2,7² + (1,6x10⁶Q)²
Q = 4,50 x10^-6 C 
[17]

QUESTION 8
8.1 Marking criteria
If any of the underlined key words/phrases in the correct context are omitted:
- 1 mark per word/phrase.
(Maximum) energy provided (work done) by a battery per coulomb/unit charge passing through it. ✓✓
Work done by the battery to move a unit coulomb of charge across the circuit. (2)

8.2 Energy (per coulomb of charge) is converted to heat in the battery due to the internal resistance. ✓✓ (2)

8.3.1
I = ^V/_R
I = ^1.5/_0.5
= 3 A (3)

8.3.2 OPTION 1
1  = 1  +  1  
R_P  R₁    R₂
1  = 1  +  1  
R_P  25    15
Rp = 9,375 Ω
Rext = 9,375 + 4 = 13,38 Ω
(13,375Ω)

OPTION 2
R_P = R₁ + R₂ 
        R₁ + R₂
R_P =(25)(15)
        25 + 15
Rp = 9,375 Ω
Rext = 9,375 + 4 = 13,38 Ω
(13,375Ω) (4)

8.3.3 POSITIVE MARKING FROM QUESTIONS 8.3.1 AND 8.3.2. (3)
OPTION 1
Ɛ = I(R + r) 
= 3(13,38 + 0,5)✓
= 41,64 V ✓ (Range: 41,625 – 41,64)

OPTION 2
Ɛ = Vext + Vint
= (3)(13,38) + 1,5 ✓
= 41,64 V (Range: 41,625 – 41,64)

8.4 Yes.
For the same voltage/potential difference, a larger current will flow through a smaller resistor (I = ^V/_R)
OR
I α^I/_R , V = constant 
I is inversely proportional to R and V is constant.
OR
V_|| = IR
= (3)(9,38)
= 28,14 V
I_R2 = V  = 28,14 = 1,13 A✓
         R       25
I_R3 = V  = 28,14 = 1,88 A✓
         R       15
OR
V is the same
I_15Ω = ²⁵/₄₀ I
I_25Ω = ¹⁵/₄₀ I (3)

8.5 Remains the same✓ (1)
[18]

QUESTION 9
9.1.1 (DC) motor(1)
9.1.2 POSITIVE MARKING FROM QUESTION 9.1.1
Electrical to mechanical /kinetic (energy) ✓✓ (2 or 0) (2)

9.1.3 Split ring/commutator (1)

9.1.4 Anticlockwise (2)

9.2.1 (The rms voltage/value of AC is) the AC voltage/potential difference which dissipates the same amount of energy/heat/power as an equivalent DC voltage/potential difference. ✓✓ (2 or 0)
ACCEPT
The rms voltage/value of AC is the DC potential difference which dissipates the same amount of energy/heat/power as AC.(2)

9.2.2 Marking criteria

• Appropriate formula for Pave
• Substitution to calculate R ✓
• Final answer: 242 Ω

OPTION 1
Pave = V²_rms 
              R
200= 220²
           R
R = 242 Ω 

OPTION 2
Pave = Vrms Irms
200 = Irms (220)
Irms = 0,909 A(0,91)
R = Vrms or R = V 
       Irms             I
200 =   220  
          0.909
R = 242 Ω (241,76 Ω)

OPTION 3
Pave = Vrms Irms
200 = Irms =  0,909 A (0,91)
Pave = Irms²R
200 = (0,909)²R ✓
R = 242 Ω
(241,52 Ω) (3)

9.2.3 Marking criteria for options 1,2 and 3

• Appropriate formula to calculate P or Irms
• Substitution
• Formula for P or W containing ✓t
• Substitution
• Final answer: 55 785,12 J ✓

POSITIVE MARKING FROM QUESTION 9.2.2.
OPTION 1
Marking criteria 

• Appropriate formula for W containing V
• Substitution
• Final answer: 55 785,12 J ✓ (5)

W = V² Δt
          R
=150² 
   242
= 55 785.12J

OPTION 2
Pave = V²_rms
               R
P_av =  92,975 W
P = ^W/_Δt
92,975 =    W     
              (10)(60)
W = 55 785,12 J ✓
(55785,12 – 55896 J)

OPTION 3
R = ^V/_I
242 = 150 
          Irms
Irms = 0,620 A
Pave = Irms Vrms
= (0,62)(150)
= 92,97 W (93 W)
P = W 
      Δt
92,975 =    W    
             (10)(60)
W = 55 785,12 J ✓
(55785,12 – 55896 J)

OPTION 4
R = ^V/_I
242 = 150 
          Irms
Irms = 0,620 A
W = I²RΔt
= (0,62)2(242)(10)(60) ✓
= 55 814,88 J ✓
(55785,12 – 55896 J)
OR
W = VIΔt
= (150)(0,62)(600)
= 55 800 J

OPTION 5
Pave = V²_rms = 150² = 92.975W
               R        242    
Pave = Irms²R
92,975 = Irms²(242)
Irms = 0,6198 A
W = I²RΔt 
= (0,6198)²(242)(10)(60) 
= 55 778,88 J 
[16]

QUESTION 10
10.1 Photoelectric effect(1)

10.2 Work function (of potassium) (1)

10.3 Potassium
It has the lowest work function / threshold frequency / highest threshold wavelength.(2)

10.4 Marking criteria/Nasienriglyne
If any of the underlined key words/phrases in the correct context are omitted:
- 1 mark per word/phrase.
The work function of a metal is the minimum energy that an electron (in the metal) needs✓ to be emitted/ejected from the metal / surface.(2)

10.5.1 Wo= hfo
= (6,63 x 10^-34)(1,75 x 10¹⁵) = W_o + 0
= 1,160 x 10-18 J ✓
OR
E = Wo + Ek(max)
hf = Wo + Ek(max)
(6,63 x 10^-34)(1,75 x 10¹⁵) = W_o + 0
W_o = 1,160 x 10^-18 J  (3)

10.5.2 POSITIVE MARKING FROM QUESTION 10.5.1.
E = Wo + Ek(max)
hf = hf_o + ½mv²_max
(6,63 x 10-34)f  = 1,160 x 10^-18 + ½(9,11 x 10^-31) (5,60 x 10⁵)²
f = 1,97 x 10¹⁵ Hz (4)
[13]
TOTAL: 150