MATHEMATICAL LITERACY P1 with Memorandum - 2024 Grade 12 June Common Exams
Share via Whatsapp Join our WhatsApp Group Join our Telegram GroupMARKS: 100
TIME: 2 hours
INSTRUCTIONS AND INFORMATION
The question paper consists of FOUR QUESTIONS. Answer ALL the questions.
Start EACH question on a new page.
Number the answers correctly according to the numbering system used in this question paper.
Leave ONE line between two sub-questions, for example between QUESTION 2.1 and QUESTION 2.2.
Use the ANSWER SHEET attached to answer QUESTION 3.6.
You may use an approved calculator (non-programmable and non-graphical), unless stated otherwise.
You may use appropriate mathematical instruments.
Show ALL formulae and substitutions in ALL calculations.
Round off your final numerical answers to a minimum of TWO decimal places.
Write neatly and legibly.
Question 1
The parking tariffs at BT Ngebs Mall in Mthatha are given below. The rate is charged per hour or part thereof. Study the table below and answer the questions that follow.
TABLE 1: PARKING TARIFF AT BT NGEBS
1.1
DISCLAIMER OF LIABILITY Saipark, BT Ngebs Mall and/or their employees, agent, or sub-contractors shall not be liable for any loss or damage of whatever nature caused, which is suffered by the customer in respect of any vehicle or any goods left or deposited with Saipark, BT Ngebs Mall/or their employees, agent, or sub-contractors, while such vehicle or goods are on the premises of BT Ngebs Mall, even where the loss is caused by the negligence or gross negligence of Saipark, BT Ngebs Mall and/or their employees or subcontractors. | ||||||||||||||||||||
| The total number of qualified teachers in South Africa in 2022 was 490 993. 68% of the teachers are female. |
1.2.1 Is the data discrete or continuous? (2)
1.2.2 Write the number of qualified teachers in words. (2)
1.2.3 Determine how many teachers are males. (3)
1.2.4 Express as a ratio the number of female teachers to the total number of qualified teachers. Write your ratio in the form 1 : … (3)
1.3 Choose the appropriate option for the following given statements. Write the question number and the letter only, for example, 1.3.3 E.
| A – Certain B – Impossible C – Even chance D – Less likely |
1.3.1 The probability of selecting a learner doing Mathematical Literacy and Mathematics.(2)
1.3.2 The probability of selecting a taxpayer receiving all the three rebates at a local school. (2) [20]
Question 2
Daniel is a 53-year-old man earning a monthly gross salary of R32 500. He contributes 7,5% of his salary towards pension each month. He contributes towards medical aid for himself, his wife and three children. Use the tax table below to answer the questions that follow. TABLE 2: 2023 TAX YEAR (1 MARCH 2022 – 28 FEBRUARY 2023)
REBATES
MEDICAL TAX CREDIT RATES
[Adapted from www.sars.gov.za. Accessed 10 January 2024] |
2.1 Determine the annual contribution towards pension. (3)
2.2 Daniel claims that his tax is more than 15% of his monthly gross salary. Show with calculations that his statement is VALID or not. (9)
2.3 Show how R239 452 in tax bracket 6 is calculated. (3)
2.4 Daniel received a lumpsum for having spent 20 years working for the same company. The company rule is to award employees with 80% of their monthly gross salary. Daniel invested the amount he received into an account offering 11,5% p.a., compounded annually. Calculate how much will he receive after three years. (6) [21]
QUESTION 3
The provincial number of voters that was recorded after the first registration in November 2023 are shown in the table below. Use the table to answer the questions that follow. TABLE 3: PROVINCIAL NUMBER OF REGISTERED VOTERS
[Adapted from www.elections.org.za. Accessed on 7 January 2024] |
3.1 Determine which province recorded the least number of voters. (2)
3.2 There are two ways to calculate the value of A. Use both methods to calculate the number of voters registered in KwaZulu-Natal and explain why the answers are different. (6)
3.3 Show that the percentage of voters in the Western Cape is 11,91%. (3)
3.4 Calculate the mean number of voters in South Africa per province. (4)
3.5 Determine the interquartile range for the number of registered voters. (6)
3.6 Use the provided ANSWER SHEET to draw the line graph for the percentage of voters per province. (4)
3.7 Give a possible reason why the number of registered voters is important to political parties. (2)
3.8 Determine the probability, as a decimal, rounded off to 3 decimal places of selecting a registered voter residing in the Cape province(s). (3) [30]
Question 4
| In the Africa Cup of Nations, the winning soccer team was promised $7 million. The team, including the technical staff, has a total number of 30 members. They are going to share the $7 million equally. |
4.1
4.1.1 Write the winning money in digits. (2)
4.1.2 Determine how much each member will receive if they win the tournament. Round off the answer to the nearest 1 000. (3)
4.2
The South African cricket team participated up to the semi-final in the 2023 Cricket World Cup. There were two main sponsors from different countries. They used their own currency to pay players for different awards such as the most sixes scored, player of the match and player of the tournament. Use the exchange rates given below to answer the questions that follow. TABLE 4: EXCHANGE RATES OF BUYING AND SELLING FOREIGN CURRENCY
|
4.2.1 There is 2,5% commission charged when converting into local currency. Calculate how much will be deposited into the player’s account, in Rands, if he was given $5 000 and £2 000 from the two main sponsors. (6)
4.2.2 Give a possible reason why there is a difference between buying and selling foreign currency. (2)
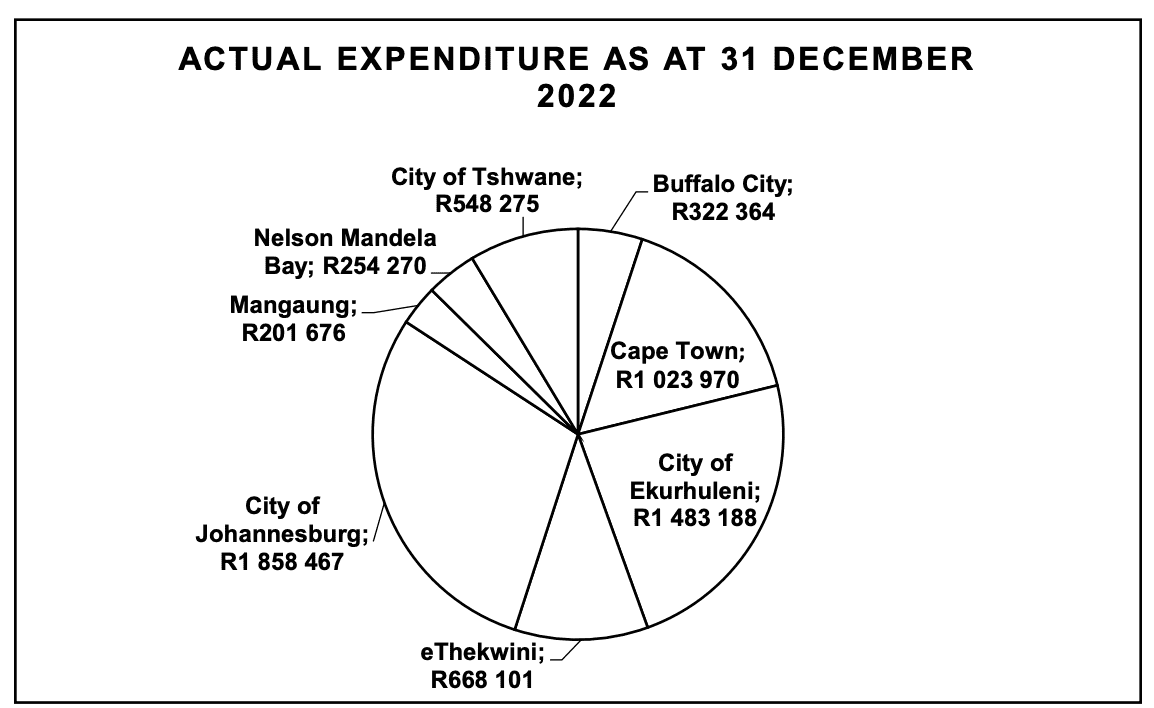
4.3 The diagram below shows the expenditure of the metropolitan municipalities of South Africa in the year ending 2022.
[Adapted from www.gov.local-government.gov.za. Accessed on 6 January 2024] |
4.3.1 How many provinces have metropolitan municipalities in the country? (2)
4.3.2 Name the type of graph that was used to represent the actual expenditure in the metropolitan municipalities of South Africa. (2)
4.3.3 Show that the probability of choosing a municipality that has an expenditure of at most R0,7 million is 62,5%. (4)
4.4 Thandi buys and sell stationery packs at schools in her community. The table below shows the cost and income per stationery pack.
|
4.4.1 Define the term break-even in the given context. (2)
4.4.2 Name the type of cost that R6 000 represents. (2)
4.4.3 Determine how many packs Thandi must sell to break-even. (4) [29]
TOTAL: 100
MARKING GUIDELINES
MARKS: 100
Symbol | Explanation |
M | Method |
MA | Method with accuracy |
CA | Consistent accuracy |
A | Accuracy |
C | Conversion |
S | Simplification |
RT | Reading from a table/graph/document/diagram |
SF | Correct substitution in a formula |
O | Opinion/Explanation |
P | Penalty, e.g. for no units, incorrect rounding off, etc. |
R | Rounding off |
NPR | No penalty for correct rounding minimum two decimal places |
AO | Answer only |
MCA | Method with constant accuracy |
NOTE:
- If a candidate answers a question TWICE, only mark the FIRST attempt.
- If a candidate has crossed out (cancelled) an attempt to a question and NOT redone the question, mark the crossed out (cancelled) version.
- Consistent accuracy (CA) applies in ALL aspects of the marking guidelines. Stop marking at the second calculation error.
- NOTE: Consistent accuracy (CA) does NOT apply in cases of a breakdown.
- If the candidate presents any extra solution when reading from a graph, and table then penalise for every extra item presented.
- As a general marking principle, if a candidate has incurred one mistake and there is evidence of sound Mathematics thereafter, then that candidate should lose ONE mark only.
Topics: F – Finance, DH – Data Handling, P – Probability
QUESTION 1 [20 MARKS] | |||
Ques. | Solution | Explanation | T&L |
1.1.1 | R10,00 ✓✓RT | 2RT reading from table (2) | F L1 |
1.1.2 | The rate will be paid per full one hour even if you spend less than one hour ✓✓ O | 2 Opinion (2) | F L1 |
1.1.3 | Amount paid: 75/100 x 20 ✓ M = R15 ✓ A | 1M multiplication 1A answer (2) | F L1 |
1.2.1 | Discrete ✓✓A | 2 A correct classification (2) | DH L1 |
1.2.2 | Four hundred and ninety thousand, nine hundred and ninety-three ✓✓A | 2 A correct wording (2) | DH L1 |
1.2.3 | 100% − 68% = 32% ✓A ∴32/100 × 490 993 = 157 117,76 ✓CA ≈ 157 118 ✓A OR Females = 68/100 x 490 993 ✓ MA = 333 875,24 MA = 490 933 – 333 875,24 ✓M = 157 117,76 = 157 118 ✓ CA | 1A calculating male percentage 1CA simplification 1A answer R
1MA calculating female number M subtracting correct values CA simplification (3) | DH L1 |
1.2.4 | 68/100× 490 993 = 333 875,24 ≈ 333 875 ✓M 333 875 :490 993 ✓M 1 : 1,47 ✓A Accept also [using percentages] 68 : 100 1 : 1,47 | 1M multiplication 1MAconcept of ratio in correct order 1CA simplification (3) | DH L1 |
1.3.1 | B ✓✓ | 2A correct option (2) | P L1 |
1.3.2 | D or B ✓✓A | 2A correct option (2) | P L1 |
[20] |
QUESTION 2 [21 MARKS] | |||
Ques. | Solution | Explanation | T&L |
2.1 | 7,5 / 100 × 12 ✓M × R32 500 ✓S = R29 250 ✓A | 1M multiply by 12 1 simplification 1A answer (3) | F L2 |
2.2 | Annual salary R32 500 × 12 = R390 000 ✓M Taxable income = R390 000 − R29 250 = R360 750✓A Annual tax = R73 726 + 31% × (R360 750 − R353 100) ✓SF = R73 726 + 0,31 × R7 650 = R73 726 + R2 371,50 = R76 097,50 Less rebate: R76 097,50 − R16 425 ✓M = R59 672,50 Less MTC R59 672,50 − [(R347 + R347 + R234 + R234 + R234) × 12] = R59 672,50 − R16 752 ✓M Annual tax = R42 920,50 Monthly tax =R42 920,50 ÷ 12✓M = R3 576,71 ✓CA 15% of salary:15 / 100× R32 500 = R4 875 ✓A Not valid. ✓O | 1MA annual salary 1A taxable income 1SF correct substitution
1MA subtracting correct rebate
1MA subtracting medical tax credit
1MCA division by 12 1CA monthly tax 1A 15% of salary 1O opinion (9) | F L3 |
2.3 | R170 734 + 39% × (R817 600 − R641 400) ✓SF ✓S = R170 734 + R68 718✓M = R239 452 | 1SF correct substitution 1simplification 1M addition (3) | F L2 |
2.4 | Lump sum = 80% × R32 500 ✓M = R26 000 ✓A Balance at the end of First Year = R26 000 + 11,5% × R26 000 ✓M = R28 990 ✓A Balance at the end of Second Year = R28 990 + 11,5% × R28 990 = R32 323,85 ✓A Balance at the end of Third Year = R32 323,85 + 11,5% × R32 323,85 = R36 041,09 ✓CA OR Balance = R26 000 × 1,115 ✓M × 1,115 ✓M × 1,115 ✓M = R36 041,09 ✓A | 1MA calculating 80% 1simplification
1M multiplication 1A answer 1A answer 1CA answer (6) | F L3 |
[21] |
QUESTION 3 [30 MARKS] | |||
Ques. | Solution | Explanation | T&L |
3.1 | Northern Cape ✓✓A | 2A answer (2) | DH L1 |
3.2 | Method 1: A = 26 850 972 − (3 348 392 + 1 422 384 + 6 274 046 + 1 965 259 + 634 792 + 2 714 474 + 1 718 340 + 3 198 146) ✓M A = 5 575 139 ✓A Method 2: 20,76/100× 26 850 972 ✓M = 5 574 261,78 ≈ 5574 262 ✓A The difference is caused by rounding off to two decimal places of the percentage. ✓✓ | 1M addition 1A answer
1M multiplication 1A rounded off answer 2O explanation (6) | DH L4 |
3.3 | 3 198 146 ÷ 26 850 972✓RT × 100% ✓M = 11,91% ✓A | 1RT correct values 1M multiplication 1 A answer (3) | DH L2 |
3.4 | Mean =26 850 972 ÷ 9✓RT ✓M = 2 983 441,333 ✓A ≈ 2 983 441 ✓R | 1RT correct values 1M division 1A answer 1R rounding (4) | DH L2 |
3.5 | Ascending order: 634 792; 1 422 384; 1 718 340; 1 965 259; 2 714 474; 3 198 146; 3 348 392; 5 575 139; 6 274 046 ✓M Lower Quartile =(1 422 384+1 718 340) ÷ 2✓MA = 1 570 362 ✓A Upper Quartile =(3 348 392 + 5 575 139) ÷ 2 = 4 461 765,5 ✓A IQR = 4 461 765,5 − 1 570 362 ✓M = 2 891 403,5 ≈ 2 891 404 ✓A | 1M arranging in ascending/ descending order 1MA calculating lower quartile 1A simplification 1A upper quartile 1CA calculating IQR 1A answer (6) | DH L2 |
3.6 | 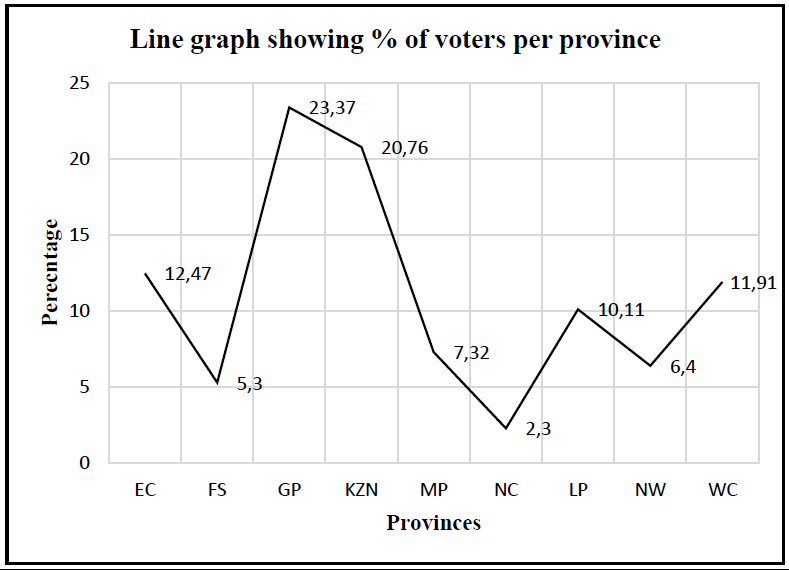 | ✓titles ✓✓plotting all 9 ✓ joining the points
(4) | DH L3 |
3.7 | To arrange campaigns ✓✓O To provide enough polling observers during elections ✓✓O [Any other valid reason] | 2 O opinion (2) | DH L4 |
3.8 | P(Cape province) = 11,91% + 2,3% + 12,47% ✓M = 26,68% ✓A = 0,267 ✓R OR P(Cape province) = (3 348 392 + 634 792 + 3 198 146) ÷ 26 850 972✓RT =7 181 330 ÷ 26 850 972✓M = 0,267 ✓A | 1M addition 1A answer 1R rounding off (3) | P L2 |
[30] |
QUESTION 4 [29 MARKS] | |||
Ques. | Solution | Explanation | T&L |
4.1.1 | 7 000 000 ✓✓A | 2A answer (2) | F L1 |
4.1.2 | $7 000 000 ÷ 30✓M = $233 333,33 ✓A ≈ $233 000 ✓R | 1M division by 30 1A answer 1R rounding off (3) | F L2 |
4.2.1 | 5 000 × 19,1305 ✓RT = R95 652,50 ✓M 2 000 × 24,3861 = R48 772,20 ✓A Total = R144 424,70 ✓A Commission 2,5 / 100× 144 424,7 = R3 610,62 ✓M Money deposited R144 424,70 − R3 610,62 = R140 814,08 ✓A | 1RT correct values 1M multiplication 1A answer 1A answer 1M multiplication 1A answer (6) | F L4 |
4.2.2 | To make profit. ✓✓O | 2O explanation (2) | F L4 |
4.3.1 | 5 provinces ✓✓A | 2A answer (2) | DH L1 |
4.3.2 | Pie chart ✓✓A | 2A answer (2) | DH L1 |
4.3.3 | 5 ✓RT / 8 ✓RT× 100 ✓M = 62,5% ✓A | 2 RT correct values 1M multiply by 100 1A answer (4) | P L2 |
4.4.1 | Income generated from selling packs is equal to the cost of packs. ✓✓O | 2O explanation (2) | F L1 |
4.4.2 | Fixed cost ✓✓A | 2A answer (2) | F L1 |
4.4.3 | Formula for income = 750n ✓M Formula for cost = 6 000 + 350n ✓M Break-even: 750n = 6 000 + 350n ✓M 400n = 6 000 n = 15 packs ✓A | 1M formula for income 1M formula for cost 1M equation 1A answer (4) | F L4 |
[29] | |||
TOTAL: 100 | |||