PHYSICAL SCIENCES CHEMISTRY PAPER 2 GRADE 12 MEMORANDUM - NSC PAST PAPERS AND MEMOS NOVEMBER 2016

More in this category: « LIFE SCIENCES PAPER 1 MEMORANDUM - NSC PAST PAPERS AND MEMOS LIFE SCIENCES PAPER 2 GRADE 12 QUESTIONS - NSC PAST PAERS AND MEMOS NOVEMBER 2016 »

Share via Whatsapp Join our WhatsApp Group Join our Telegram Group

PHYSICAL SCIENCES (CHEMISTRY)
PAPER TWO (P2)
GRADE 12
NSC EXAM PAPERS AND MEMOS
NOVEMBER 2016

MEMORANDUM

QUESTION 1
1.1 D ✓✓ (2)
1.2 C ✓✓ (2)
1.3 C ✓✓ (2)
1.4 D ✓✓ (2)
1.5 B ✓✓ (2)
1.6 D ✓✓ (2)
1.7 A ✓✓ (2)
1.8 A ✓✓ (2)
1.9 B ✓✓ (2)
1.10 B ✓✓ (2)

[20]

QUESTION 2
2.1

2.1.1 A OR/OF D ✓ (1)
2.1.2 B ✓ (1)
2.1.3 E ✓ (1)
2.1.4 D ✓ (1)

2.2

2.2.1 (3)

Marking criteria:

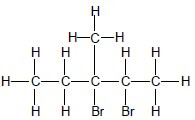
Five C atoms in longest chain. ✓
Two Br and one methyl substituents. ✓
Whole structure correct. ✓

2.2.2 (2)

Marking criteria:

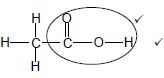
Whole structure correct: 2/2
Only functional group correct: Max 1/2
Accept -OH as condensed.
IF:
More than one functional group 0/2

2.2.3 (2)

Marking criteria:

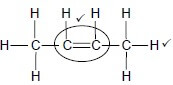
Whole structure correct: 2/2
Only functional group correct 1/2

IF: More than one functional group 0/2

2.3

2.3.1 Hydrogen (gas) ✓ (1)
2.3.2 Addition / Hydrogenation ✓ (1)

[13]

QUESTION 3
3.1 Compounds with the same molecular formula ✓ but different structural formulae.✓ (2)
3.2 Chain✓ (1)
3.3 From A to C:

Structure:
Less branched / less compact / less spherical/longer chain length / larger surface area (over which intermolecular forces act).✓
Intermolecular forces:
Stronger / more intermolecular forces / Van der Waals forces / London forces / dispersion forces. ✓
Energy:
More energy needed to overcome or break intermolecular forces / Van der Waals forces. ✓
OR
From C to A:
Structure:
More branched / more compact / more spherical / smaller surface area (over which intermolecular forces act).✓
Intermolecular forces:
Weaker / less intermolecular forces / Van der Waals forces / London forces / dispersion forces. ✓
Energy:
Less energy needed to overcome or break intermolecular forces / Van der Waals forces. ✓ (3)

3.4 A / 2,2-dimethylpropane ✓ → Lowest boiling point. ✓ (2)
3.5 C₅H₁₂ + 8O₂ ✓ ⭢ 5CO₂ + 6H₂O ✓ Bal ✓ (3)

Notes:

Reactants ✓ Products ✓ Balancing ✓
Ignore double arrows and phases.
Marking rule 6.3.10.
If condensed structural formulae used:Max.2/3

[11]

QUESTION 4
4.1

4.1.1 High temperature / heat / high energy / high pressure ✓ (1)
4.1.2 C6H12 ✓ (1)

Accept: Condensed structural formula and structural formula.
E.g : CH₃CH₂CH₂CH₂CHCH₂

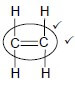
4.1.3 Alkenes ✓ (1)

4.2 X / C6H12 / Alkene / Hexene ✓
OPTION 1

X is an alkene / has a double bond / unsaturated. ✓
X can undergo addition. ✓
X will react without light / heat / is more reactive. ✓

OPTION 2

Butane is an alkane OR butane is saturated. ✓
Butane can only undergo substitution. ✓
Butane will only react in the presence of light / heat OR butane is less reactive. ✓ (4)

4.3

4.3.1 2-chloro✓butane ✓  (2)
4.3.2 Substitution / Hydrolysis ✓  (1)
4.3.3   (2)

Marking criteria:

Whole structure correct 2/2
Only functional group correct 1/2

IF: More than one functional group 0/2

4.3.4 Hydration ✓ (1)

[13]

QUESTION 5
5.1

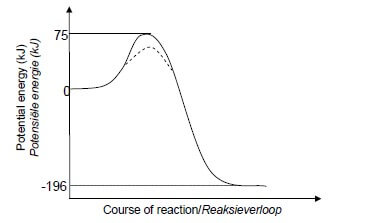
5.1.1 The minimum energy needed for a reaction to take place. ✓✓
OR
Minimum energy needed to form the activated complex(2)
5.1.2 (3)

Marking criteria:
Shape of curve for exothermic reaction as shown.	✓
Energy of activated complex shown as 75 kJ in line with the peak.	✓
Energy of products shown as − 196 kJ below the zero.	✓
IF: Wrong shape, e.g. straight line.	⁰/₃

5.1.3 Marking criteria

Dotted line (---) on graph in QUESTION 5.1.2 showing lower energy for activated complex. ✓
Dotted curve starts at/above energy of reactants and ends at/above energy of products on the inside of the original curve. ✓ (2)

Note:
Allocate marks only if curve for either exothermic or endothermic reaction drawn in QUESTION 5.1.2.

5.1.4

A catalyst provides an alternative pathway of lower activation energy. ✓
More molecules have sufficient / enough (kinetic) energy. ✓
OR
More molecules have kinetic energy equal to or greater than the activation energy.
More effective collisions per unit time / second. ✓
OR
Rate / frequency of effective collisions increases. (3)

5.2

5.2 .1 Ave rate. tempo = ΔV
Δt
= 52 - 16  ✓
40 - 10
=1,2(dm³.s^-1)✓  (3)

Accept:

Volume range: 16 to 17 cm³
Answer range: 1,167 to 1,2 dm³∙s^-1

5.2.2 (4)

Marking criteria:

V(O₂) = 60 dm³ AND divide volume by 24 ✓
Use ratio: n(H₂O₂) = 2n(O₂) = 1:2 ✓
Use 34 g∙mol^-1 in n = m or in ratio calculation. ✓
M
Final answer: 170 g ✓

OPTION 1
n(O₂) = V
V_M
= 60 ✓
24
= 2,5 mol
n(H₂O₂) = 2n(O₂)
= 2(2,5) ✓
= 5 mol
n(H₂O₂) = m
M
∴5 = m
34 ✓
∴m = 170 g ✓

OPTION 2
24 dm³ : 1 mol
60 dm³ : 2,5 mol ✓
n(H₂O₂) = 2n(O₂)
= 2(2,5) ✓
= 5 mol
34 g ✓: 1 mol
x : 5 mol
x = 170 g ✓

OPTION 3

n(O₂) = V
V_M
= 60 ✓
24
= 2,5 mol
n(O₂) = m
M
∴2,5 = m
32
∴m = 80 g
✓
2(34) g✓ H₂O₂ .......32 g O₂ x g H₂O₂ ................ 80 g O₂m(H₂O₂) = 170 g ✓

5.2.3 Equal to (1)

5.3

5.3.1 Q ✓ (1)
5.3.2 P ✓ (1)

[20]

QUESTION 6
6.1 The stage in a chemical reaction when the rate of forward reaction equals the rate of reverse reaction. ✓✓ (2 marks or no marks)
OR
The state where the concentrations / quantities of reactants and products remain constant. (2)
6.2

6.2.1 Remains the same✓ (1)
6.2.2 Decreases ✓

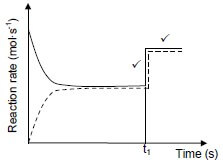
When the temperature is increased the reaction that will oppose this increase / decrease the temperature will be favoured. ✓
OR
The forward reaction is exothermic.
An increase in temperature favours the endothermic reaction. ✓
The reverse reaction is favoured. ✓ (4)

6.3

Marking criteria:

Vertical parallel lines show a sudden increase in rate of both forward and reverse reactions. ✓
Horisontal parallel lines showing a constant higher rate for both forward and reverse catalysed reactions after time t1. ✓

(2)

6.4 CALCULATIONS USING NUMBER OF MOLES

Marking criteria:

Use M(PbS) = 239 g∙mol^-1 in n = m or in ratio calculation ✓
M
Use ratio: n(H₂S)_equil = n(PbS) ✓
n(H₂S)_formed = n(H₂S)_equilibrium✓
USING ratio: H₂ : H₂S = 1 : 1 ✓
n(H₂)_equilibrium = n(H₂)_initia – n(H₂)_formed ✓
Divide equilibrium n(H₂S) & n(H₂) by 2 dm³. ✓
Correct K_c expression ✓
Substitution of concentrations into K_c expression. ✓
Final answer: 0,07 ✓
NB.: If not rounded: 0,067

OPTION 1
n(PbS) = m = 2,39 = 0,01 mol
M 239 ✓
n(H₂S)_equilibrium = n(PbS) ✓ = 0,01 mol

	H₂	H₂S
Initial quantity (mol)	0,16	0
Chnage (mol)	0,01	0,01✔	ratio✔
Quantity at equilibrium (mol)	0,15 ✔	0,01
Equlibrium concentration (mol.dm^-3)	0,075	0,005	divide by 2✔

Kc = [H₂S ] ✔
[H₂]
= 0,005 ✔
0,075
=0,067 = 0,07 ✔

No K_c expression, correct substitution: Max : 8/9

Wrong K_c expression: Max : 6/9

IF: [S] = 1 in Kc = [H₂S ]
[H₂][S]
No mark for K_c expression, but continue marking substitution and answer

OPTION 2
n(PbS) = m = 2,39 = 0,01 mol
M 239 ✓
n(H₂S)r_eacted = n(PbS) ✓ = 0,01 mol
= n(H₂S)_equilibriumn(H₂S)_formed= n(H₂S)_equilibrium – n(H₂S)_initial = 0,01 – 0 ✓
= 0,01 mol
n(H₂)_reacted = n(H₂S)_formed✓ = 0,01 mol
n(H₂)_equilibrium = n(H₂)_initial - n(H₂)_reacted= 0,16 - 0,01 ✓
= 0,15 mol
c(H₂) = n c(H₂S) = n
V V
= 0,15 = 0,01
2 2
= 0, 075 mol.dm^-3 = 0,005 mol.dm^-3

Kc = [H₂S ] ✔
[H₂]
= 0,005 ✔
0,075
=0,067 = 0,07 ✔

No K_c expression, correct substitution: Max : 8/9

Wrong K_c expression: Max : 6/9

IF: [S] = 1 in Kc = [H₂S ]
[H₂][S]
No mark for K_c expression, but continue marking substitution and answer

OPTION 3

	H₂	H₂S
Initial quantity (mol)	0,16	0
Change (mol)	x	x✔	ratio✔
Quantity at equilibrium (mol)	0,16 - x ✔	x
Equlibrium concentration (mol.dm^-3)	0,16 - x 2	x 2	divide by 2✔

n(PbS) = m = 2,39 = 0,01 mol
M 239 ✓
n(H₂S)_equilibrium = n(PbS)  ✔ ∴ x = 0,01 mol
[H₂]_equilibrium = 0,16 - 0,01 = 0,075  mol.dm^-3
2
[H₂S]_equilibrium =  0,01 = 0,005  mol.dm^-3
2

Kc = [H₂S ] ✔
[H₂]
= 0,005 ✔
0,075
=0,067 = 0,07 ✔

No K_c expression, correct substitution: Max : 8/9

Wrong K_c expression: Max : 6/9

IF: [S] = 1 in Kc = [H₂S ]
[H₂] [S]
No mark for K_c expression, but continue marking substitution and answer

CALCULATIONS USING CONCENTRATION

Marking criteria:

Use M(PbS) = 239 g∙mol^-1 in n = m or in ratio calculation ✓
Use ratio: n(H₂S)_equil = n(PbS) ✓
Divide equilibrium n(H₂S)equil & n(H₂)initial by 2 dm³. ✓
(H₂S) _formed = (H₂S)_equal ✓
USING ratio: H₂:H₂S =1 :1
[H₂]_equilibrium = [H₂]_initial – [H₂]_formed ✓
Correct K_c expression ✓
Substitution of concentrations into K_c expression. ✓
Final answer: 0,07 ✓

Note: If not rounded: 0,067

OPTION 4

n(PbS) = m = 2,39 = 0,01 mol
M 239 ✓
n(H₂S)_equilibrium = n(PbS) ✓ = 0,01 mol

	H₂	H₂S
Initia concentration ( mol.dm^-3 )	0,16 / 2 = 0,08	0
Change in concentration ( mol.dm^-3 )	0,05	0,05✔	ratio✔
Equlibrium concentration (mol.dm^-3)	0,075	0,005	divide by 2✔

Kc = [H₂S ] ✔
[H₂]
= 0,005 ✔
0,075
=0,067 = 0,07 ✔

No K_c expression, correct substitution: Max : 8/9

Wrong K_c expression: Max : 6/9

IF: [S] = 1 in Kc = [H₂S ]
[H₂][S]
No mark for K_c expression, but continue marking substitution and answer

OPTION 5

n(PbS) = m = 2,39 = 0,01 mol
M 239 ✓
n(H₂S)_equilibrium = n(PbS) ✓ = 0,01 mol
[H₂S]e_quilibrium = n
V
= 0.01
2
= 0,005  mol.dm^-3
[H₂]_initial =   n
V
= 0.16
2
= 0,008 mol.dm^-3
[H₂S]_formed ^{= [H₂S]}_equilibrium^{- [H2S]}_{initial
= 0,005 - 0  ✓
= 0,005} mol.dm^-3[H₂]_reacted = [H₂S]_formed = 0,005 mol
^[H₂]equilibrium= [H₂]initial- [H₂]_reacted= 0,008 - 0,005
^{= 0,075 mol}

Kc = [H₂S ] ✔
[H₂]
= 0,005 ✔
0,075
=0,067 = 0,07 ✔

No K_c expression, correct substitution: Max : 8/9

Wrong K_c expression: Max : 6/9

IF: [S] = 1 in Kc = [H₂S ]
[H₂][S]
No mark for K_c expression, but continue marking substitution and answer

(9)
[18]

QUESTION 7
7.1

7.1.1 Hydrolysis is the reaction (of a salt) with water. ✓✓ (2 or 0)
Accept:
A chemical reaction in which water is a reactant. (2)

7.1.2 Smaller than (7) ✓
NH₄⁺ + H₂O ✓ → NH₃ + H₃O⁺✓
Accept:
NH₄Cℓ + H₂O → NH₃ + H₃O⁺ + Cℓ^-
NH₄⁺ → NH₃ + H⁺((3)

Note:

Mark equation independently of first answer.
If incorrect balancing: Max 2/3

7.2

Marking criteria for equation:

Reactants ✓ Products ✓
Ignore double arrows and phases.
Marking rule 6.3.10

7.2.1 (2)

Marking guidelines:

Substitution of 98 g∙mol^-1. ✓
Final answer: 0,08 mol ✓
Note:
If not rounded: (0,075 mol)

OPTION 1

n = m = 7,35 = 0,08 mol
M 98 ✓
(0,075 mol)

OPTION 2
98 g ✓: 1 mol
7,35 :0,08 mol ✓

OPTION 3

n = m = 7,35
MV 98 × 0,5✓
= 0,15 mol∙dm^-3
n = cV
= 0,15 x 0,5
= 0,08 mol ✓

7.2.2 POSITIVE MARKING FROM QUESTION 7.2.1.

OPTION 1

pH = −log[H₃O⁺] ✓
1,3 ✓ = −log[H₃O⁺]
[H₃O⁺] = 0,05 mol∙dm^-3[H₂SO₄] = ½[H₃O⁺]
= ½ x 0,05 ✓
= 0,025 mol∙dm^-3 (0,03)

n(H₂SO₄)_ex = cV ✓
= 0,025 x 0,5 ✓
= 0,0125 mol (0,02)
n(H₂SO₄)_react = 0,075 – 0,0125 ✓
[the highlighted part is from Q7.2.1]
= 0,0625 mol (0,06)
n(NaOH) = 2n(H₂SO₄)
= 2 x 0,0625 ✓
= 0,125 mol (0,12)

OR EITHER⇒⇒⇒
⇓
⇓
⇓

n(NaOH) = m = 0,125 = m
M 40 ✓

m = 5 g ✓ (4,8 g)

Marking guidelines:

Formula: pH = −log[H₃O⁺] ✓
Substitution of 1,3 ✓
Use [H₂SO₄] : [H₃O⁺] = 1 : 2 ✓
Formula: c = n ✓
V
Multiply by 0,5 dm³ ✓
Subtract n_initial – n_excess ✓
Use n(NaOH) : n(H₂SO₄) = 2:1 ✓
Substitution of 40 g∙mol^-1 ✓
Final answer: m = 5 g ✓
Range: 4,8 – 5,6 g

1 mol : 40 g ✓
0,125 mol : 5 g ✓

OPTION 2

pH = −log[H₃O⁺] ✓
1,3 ✓ = −log[H₃O⁺]
[H₃O⁺] = 0,05 mol∙dm^-3n(H₃O⁺)_ex= cV ✓
= (0,05)(0,5) ✓
= 0,025 mol (0,03)
n(H₃O⁺)_in = 2n(H₂SO₄)
[the highlighted part is from Q7.2.1]
= 0,075 x 2 ✓
= 0,15 mol (0,16)
n(H₃O⁺)_react = 0,15 – 0,025 ✓
= 0,125 mol (0,13)
n(NaOH) = n(H3O⁺) ✓
= 0,125 mol (0,13)

OR EITHER⇒⇒⇒
⇓
⇓
⇓

n(NaOH) = m = 0,125 = m
M 40 ✓

m = 5 g ✓ (5,2 g)

Marking guidelines:

Formula: pH = −log[H₃O⁺] ✓
Substitution of 1,3 ✓
Formula/Formule: c = n ✓
V
Multiply by 0,5 dm³ ✓
Use n(H2SO4) : n(H₃O⁺) = 1 : 2 ✓
Subtract n_initial – n_excess ✓
Use n(H₃O⁺) : n(NaOH) = 1 : 1 ✓
Substitution of 40 g∙mol^-1 ✓
Final answer: m = 5 g ✓
Range: 4,8 – 5,6 g

1 mol : 40 g ✓
0,125 mol : 5 g ✓

OPTION 3
[H₂S]e_in = n = 0,075
V 0,5
[the highlighted part is from Q7.2.1]
= 0,15 mol∙dm-³ (0,16)
[H₃O+]_in = 2[H₂SO₄]
= 2 x 0,15 ✓

= 0,3 mol∙dm^-3 (0,32)
pH = −log[H₃O⁺] ✓
1,3 ✓ = -log[H₃O⁺]
[H₃O⁺] = 0,05 mol∙dm^-3
[H₃O⁺]_react = 0,3 – 0,05✓
= 0,25 mol∙dm^-3 (0,27)
[H₂SO₄]_react = ½[H₃O⁺]
= ½ x 0,25
= 0,125 mol∙dm-3 (0,14)

Marking guidelines:

Formula: c = n ✓
V
Divide by 0,5 dm³ ✓
Use [H₃O⁺] : [H₂SO₄] = 2:1 ✓
Formula: pH = −log[H₃O⁺] ✓
Substitution of 1,3 ✓
Subtract [H₃O⁺]_initial – [H₃O⁺]_excess ✓
Use n(NaOH) : n(H₂SO₄) = 2:1 ✓
OR
Use [H₂SO₄] : [NaOH] = 1 : 2 ✓
Substitution of 40 g∙mol^-1 ✓
Final answer: m = 5 g ✓
Range: 4,8 – 5,6 g

n(H₂SO₄)_react = cV
= (0,125)(0,5)
= 0,0625 mol (0,07)
n(NaOH) = 2n(H₂SO₄)
= 2 x 0,0625 ✓
= 0,125 mol (0,14)

n(NaOH) = m
M
0,125 = m
40✓
m = 5 g ✓ (5,6 g)

[H₂SO₄] : [NaOH]

1 : 2
0,125 : 0,25 ✓ (0,28)

m = cMV
= 0,25 x 40 ✓x 0,5
= 5 g ✓ (5,6 g)

(9)
[16]

QUESTION 8

8.1

8.1.1 AgNO₃ / Silver nitrate ✓ (1)
8.1.2 Ni → Ni²⁺ + 2e^- ✓✓ (2)

Marking guidelines:

Ni ⇌ Ni²⁺ + 2e^- ½ Ni²⁺ + 2e^- ⇌ Ni ⁰/₂
2 Ni²⁺ + 2e^- → Ni ½ Ni²⁺ + 2e^- ← Ni ⁰/₂
Ignore if charge omitted on electron.
If charge (+) omitted on Ni²⁺: Max: 21 Example: Ni → Ni² + 2e^- ✓

8.1.3 Ni + 2Ag⁺ ✓ → Ni²⁺ + 2Ag ✓ Bal ✓
OR
Ni + 2 AgNO₃ → Ni(NO₃)₂ + 2Ag (3)

Notes:

Reactants ✓ Products ✓ Balancing: ✓
Ignore double arrows.
Marking rule 6.3.10/

8.2

8.2.1 Ni ✓ - Ni is a stronger reducing agent. / Ni has a higher reducing ability. / Ni is the anode. / Ni loses electrons. / Ni is oxidised. ✓ (2) ✓ ✓ ✓

8.2.2 Ni (s) | Ni²⁺ (aq) || Ag⁺(aq) | Ag(s)
OR
Ni (s) | Ni²⁺ (1 mol∙dm^-3) || Ag⁺(1 mol∙dm^-3) | Ag(s)
Accept:
Ni | Ni²⁺ || Ag⁺ | Ag (3)

8.2.3 (4)

OPTION 1:
E^θ_cell = E^θ_reduction −E^θ_oxidation✓
= 0,80 ✓ – (-0,27) ✓ = 1,07 V ✓

Notes

Accept any other correct formula from the data sheet
Any other formula using unconventional abbreviations, e.g. E°cell = E°OA - E°RA followed by correct substitutions: ¾

OPTION 2
Ag⁺ + e^- ⭢ Ag✓ E^θ = 0,80 V ✓
Ni ⭢ Ni²⁺ + 2e^- E^θ = +0,27 V ✓
Ag⁺ + Ni ⭢ Ag + Ni²⁺ E^θ = +1,07 V ✓

8.2.4 Increases ✓ (1)

[16]

QUESTION 9
9.1 Endothermic ✓ (1)
9.2 Anode ✓ - Connected to the positive terminal of the battery. ✓ (2)

9.3

9.3.1 Chlorine (gas) / Cℓ₂✓ (1)
9.3.2 Hydrogen (gas) /H₂ ✓ (1)
9.3.3 2H₂O(ℓ) + 2e^- ⭢ H₂(g) + 2OH^-(aq) ✓✓ (2)
Ignore phases

Notes
H₂(g) + 2OH^-(aq) ← 2H₂O(ℓ) + 2e^- 2/2 2H₂O(ℓ) + 2e^- ⇌ H₂(g) + 2OH^-(aq) 1/2
H₂(g) + 2OH^-(aq) ⇌ 2H₂O(ℓ) + 2e^- 0/2 2H₂O(ℓ) + 2e^- ← H₂(g) + 2OH^-(aq) 0/2

9.4 Basic ✓ - OR Alkaline
OH⁻ (ions) / NaOH / Strong base forms.✓ (2)

[9]

QUESTION 10
10.1

10.1.1 Haber (process) ✓ (1)
10.1.2 Contact process / Catalytic oxidation of SO₂ ✓ (1)
10.1.3 Sulphur trioxide / SO₃ ✓ (1)
10.1.4 SO₃ + H₂SO₄ ✓ ⭢ H₂S₂O₇ ✓ Bal. ✓ (3)

Notes

Reactants ✓ Products ✓ Balancing ✓
Ignore ⇌ and phases
Marking rule 6.3.10

10.1.5 H₂SO₄ ✓ + 2NH₃ ✓ ⭢ (NH₄)₂SO₄ ✓ Bal. ✓ (4)

Notes

Reactants ✓✓ Products ✓ Balancing ✓
Ignorer ⇌ and phases
Marking rule 6.3.10

10.2 (4)

Marking guidelines:

Calculate the mass of fertiliser.
Add %N and %P OR mass N and mass P.
Subtraction: 100 – (%N + %P)
OR m(fertiliser) – [m(N) + m(P)]
OR %fertiliser – [%N + %P]
Final answer: 8:1:5

OPTION 1:

m(fertiliser) = ³⁶/₁₀₀ x 20
= 7,2 kg
%N = 4,11 x 100
7,2
= 57,08%
%P = 0,51 x 100
7,2
= 7,08%
%K = 100 – ✓ (57,08 + 7,08) ✓
= 35,84%
57,08 : 7,08 : 35,84
8 : 1 : 5 ✓

OPTION 2:

m(fertiliser) = ³⁶/₁₀₀ x 20 ✓
= 7,2 kg
m(K) = 7,2 – ✓ (4,11 + 0,51) ✓
= 2,58 kg
4,11 : 0,51 : 2,58
8 : 1 : 5 ✓

OPTION 3

%N =4,11 x 100 = 20,55%  ✓
20
%P =0,51 x 100  = 2,55%
20
%K = 36 –✓ (20,55 + 2,55) ✓ = 12,9%

20,55 : 2,55 : 12,9
8 : 1 : 5 ✓

[14]
TOTAL: 150

Last modified on Tuesday, 15 June 2021 12:40

Published in Grade 12 NSC Exam Papers and Memos 2016 November

PHYSICAL SCIENCES CHEMISTRY PAPER 2 GRADE 12 MEMORANDUM - NSC PAST PAPERS AND MEMOS NOVEMBER 2016

MEMORANDUM

Related items