PHYSICAL SCIENCES GRADE 12 PAPER 1 MEMORANDUM - NSC PAST PAPERS AND MEMOS SEPTEMBER 2017

Friday, 23 July 2021 12:26

PHYSICAL SCIENCES GRADE 12 PAPER 1 MEMORANDUM - NSC PAST PAPERS AND MEMOS SEPTEMBER 2017

More in this category: « MECHANICAL TECHNOLOGY GRADE 12 QUESTIONS - NSC PAST PAPERS AND MEMOS SEPTEMBER 2017 PHYSICAL SCIENCES GRADE 12 PAPER 2 MEMORANDUM - NSC PAST PAPERS AND MEMOS SEPTEMBER 2017 »

Share via Whatsapp Join our WhatsApp Group Join our Telegram Group

PHYSICAL SCIENCES
GRADE 12
PAPER 1
NSC PAST PAPERS AND MEMOS
SEPTEMBER 2017

GENERAL GUIDELINES
1. CALCULATIONS
1.1 Marks will be awarded for: correct formula, correct substitution, correct answer with unit.
1.2 No marks will be awarded if an incorrect or inappropriate formula is used, even though there are many relevant symbols and applicable substitutions.
1.3 When an error is made during substitution into a correct formula, a mark will be awarded for the correct formula and for the correct substitutions, but no further marks will be given.
1.4 If no formula is given, but all substitutions are correct, a candidate will forfeit one mark.
1.5 No penalisation if zero substitutions are omitted in calculations where correct formula/principle is correctly given.
1.6 Mathematical manipulations and change of subject of appropriate formulae carry no marks, but if a candidate starts off with the correct formula and then changes the subject of the formula incorrectly, marks will be awarded for the formula and correct substitutions. The mark for the incorrect numerical answer is forfeited.
1.7 Marks are only awarded for a formula if a calculation has been attempted, i.e. substitutions have been made or a numerical answer given.
1.8 Marks can only be allocated for substitutions when values are substituted into formulae and not when listed before a calculation starts.
1.9 All calculations, when not specified in the question, must be done to a minimum of two decimal places.
1.10 If a final answer to a calculation is correct, full marks will not automatically be awarded. Markers will always ensure that the correct/appropriate formula is used and that workings, including substitutions, are correct.
1.11 Questions where a series of calculations have to be made (e.g. a circuit diagram question) do not necessarily always have to follow the same order. FULL MARKS will be awarded provided it is a valid solution to the problem. However, any calculation that will not bring the candidate closer to the answer than the original data, will no count any marks.

2. UNITS
2.1 Candidates will only be penalised once for the repeated use of an incorrect unit within a question.
2.2 Units are only required in the final answer to a calculation.
2.3 Marks are only awarded for an answer, and not for a unit per se. Candidates will therefore forfeit the mark allocated for the answer in each of the following situations:

Correct answer + wrong unit
Wrong answer + correct unit
Correct answer + no unit

2.4 SI units must be used except in certain cases, e.g. V.m-1instead of N.C^-1, and cm•s^-1 or km•h-1instead of m•s-1 where the question warrants this.

3. GENERAL
3.1 If one answer or calculation is required, but two are given by the candidate, only the first one will be marked, irrespective of which one is correct. If two answers are required, only the first two will be marked, etc.
3.2 For marking purposes, alternative symbols (s, u, t, etc.) will also be accepted.
3.3 Separate compound units with a multiplication dot, no a full stop, for example, m•s^-1.
For marking purposes, m•s^-1 and m/s will also be accepted.

4. POSITIVE MARKING
Positive marking regarding calculations will be followed in the following cases:
4.1 Subquestion to subquestion: When a certain variable is calculated in one subquestion (e.g. 3.1) and needs to be substituted in another (3.2 of 3.3), e.g. if the answer for 3.1 is incorrect and is substituted correctly in 3.2 or 3.3, full marks are to be awarded for the subsequent subquestions.
4.2 A multistep question in a subquestion: If the candidate has to calculate, for example, current in die first step and gets it wrong due to a substitution error, the mark for the substitution and the final answer will be forfeited.

5. NEGATIVE MARKING
Normally an incorrect answer cannot be correctly motivated if based on a conceptual mistake. If the candidate is therefore required to motivate in QUESTION 3.2 the answer given in QUESTION 3.1, and 3.1 is incorrect, no marks can be awarded for QUESTION 3.2. However, if the answer for e.g. 3.1 is based on a calculation, the motivation for the incorrect answer could be considered.

MEMORANDUM

QUESTION 1
MULTIPLE-CHOICE QUESTIONS
1.1 D ✓✓ (2)
1.2 D ✓✓ (2)
1.3 C ✓✓ (2)
1.4 B ✓✓ (2)
1.5 B ✓✓ (2)
1.6 D✓✓ (2)
1.7 A ✓✓ (2)
1.8 C ✓✓ (2)
1.9 C ✓✓ (2)
1.10 A ✓✓ (2) [20]

QUESTION 2

2.1	If the resultant/net force acts on an object, the object will accelerate in the direction of the resultant/net force with an acceleration that is directly proportional to the resultant/net force ✓ and inversely proportional to the mass ✓ of the object.					(2)
2.2			✓ for both components			(5)
2.3	2.3.1	(For the vertical motion) F_net = 0 ✓ any one F_N + F_AY +(- w) = 0 F_N + F_AY = w F_N + F_A.sin30° = 5 × 9,8 ✓ F_N = 49 – (5 × 9,8 × sin 30°) FN = 24,5 N ✓			F_AY is the vertical component of FA. F_A = 5 N	(3)
	2.3.2	f_k = μk N ✓ any one f_k = μk mg f_k = 0,2 × 24,5 ✓ f_k = 4,9 N ✓				(2)
	2.3.3	Positive marking from 2.3.1: At 5 kg block B: F_net = ma T + (-f) + (-F_AX) = ma T = 5 × a ✓ T = 5a + 4,9 + 5 cos 30°✓.....(1)		At 12 kg block A W + (-T) = ma mg – T = 12 × a 12 × 9,8 – T = 12 × a T = 117,6 – 12a ✓........ (2) (1) = (2): 117,6 –12a = 5a + 4,9 + 5cos30° 117,6 – (4,9+5cos30°)=5a + 12a 108,369873 = 17a a = 6,37 m•s^-2 ✓		(4)
						[16]

QUESTION 3

Related Items

3.1	Free-fall is the motion of an object when the only force acting on it is gravitational force ✓✓				(2)
3.2	3.2.1	OPTION 1 (downwards positive) v_f = v_i + a∆t ✓ 0 = (-15) + (9,8)x ∆t ✓ ∆t = 1,53 s✓		OPTION 2 (upwards positive) v_f = v_i + a∆t ✓ 0 = (15) + (-9,8)∆t ✓ ∆t = 1,53 s✓	(3)
	3.2.2	OPTION 1 (downwards positive) vf = vi + a∆t ✓ = (-15) + (9,8)(2,4) ✓ = 8,52 vf = 8,52 m•s^-1 ✓ (downwards ) ✓		OPTION 2 (upwards positive) vf = vi + a∆t ✓ = (15) + (-9,8)(2,4) ✓ = -8,52 vf = 8,52 m•s^-1 ✓ (downwards ) ✓	(4)
		OPTION 3 (downwards positive) Descent : Y – X: ∆t = 2,4 – 1,53 = 0,87 s vf = vi + a∆t ✓ = 0 + (9,8)(0,87) ✓ = 8,53 m•s^-1 ✓ (downwards ) ✓		OPTION 2 (upwards positive) Descent : Y – X: ∆t = 2,4 – 1,53 = 0,87 s vf = vi + a∆t ✓ = 0 + (-9,8)(0,87) ✓ = -8,53 m•s^-1 = 8,53 m•s^-1 ✓ (downwards ) ✓
3.3	OPTION 1 (upwards positive) A – Y: ∆y = vi∆t + ½ a∆t² ✓ = 15 × 1,53 + ¹/₂(-9,8)(1,53)²✓ = 11,48 m ✓ Y – X: ∆t = 2,4 – 1,53 = 0,87 s ∆y = vi∆t + ¹/₂a∆t² = 0 × 0,87 + ¹/₂(-9,8)(0,87)²✓ = -3,71 ✓ = 3,71 m downwards/afwaarts Height of the building is given by: h = 11,48 + 1,8 – 3,71 ∴h = 9,57 m ✓		OPTION 2 (downwards positive) A – Y: ∆y = vi∆t + ½ a∆t² ✓ = -15 × 1,53 + ¹/₂(9,8)(1,53)² ✓ = -11,48 m ✓ = 11,48 m upwards Y – X: ∆t = 2,4 – 1,53 = 0,87 s ∆y = vi∆t + ¹/₂a∆t² = 0 × 0,87 + ¹/₂(9,8)(0,87)²✓ = 3,71 m ✓ Height of the building is given by: h = -11,48 – 1,8 +3,71 ∴h = -9,57 m ∴h = 9,57 m ✓

	OPTION 3 (upwards positive) (Y-X): v_f = v_i + a∆t -8,52 = (0) + (-9,8) ∆t ∆t = 0,87 s ✓ (A-Roof): 1,53 = ∆t + 0,87 ∆t = 0,66 s ✓ (A-X): v_f² = v_i² + 2a∆y ✓ (8,52)² = (15)² + 2(-9,8)∆y ✓ ∆y = 7,78 m ✓ Height: h = 7,78 + 1,8 = 9,58 m ✓	OPTION 4 (upwards positive) (Y-X): v_f = v_i + a∆t -8,52 = (0) + (-9,8) ∆t ∆t = 0,87 s ✓ (A-Roof): 1,53 = ∆t + 0,87 ∆t = 0,66 s ✓ (A-X): ∆y = v_i . ∆t + ¹/₂g∆t²✓ = 15 × 0,66 + ¹/₂x – 9,8 × 0,66² ✓ = 7,77 m ✓ Height: h = 7,78 + 1,8 = 9,58 m ✓		(6)
3.4	OPTION 1 (downwards positive) Δt (s)	OPTION 2 (upwards positive) Δt (s)		(3)
	Criteria for graph		Marks/
	Initial velocity		✓
	Final velocity		✓
	Time at Y, maximum height		✓
				[18]

QUESTION 4

4.1	In an isolated system ✓the total mechanical energy remains constant. ✓ ✓			(2)
4.2	OPTION 1 E_mech at/by A = E_mech at/by B ✓ Any one (mgh + ¹/₂mv²) at/by A = (mgh + ¹/₂mv²) at/by B 4 × 9,8 × (7 sin 60°) ✓+ ¹/₂ × 4 × 0 = 4 x 9,8 × (4 sin 60°)✓+ ¹/₂× 4 × v² v = 7,14 m•s^-1 ✓			(4)
	OPTION 2 E_mech at/by A = E_mech at/by B ✓ (mgh + ¹/₂mv²) at A = (mgh + ¹/₂mv²) at B 4 × 9,8 × (3 sin 60°) ✓+ ¹/₂× 4 × 0 = 4 × 9,8 × (0)✓+ ¹/₂× 4 × v² v = 7,14 m•s^-1 ✓
4.3	4.3.1	Work done by a net force is equal✓ to the change in kinetic energy✓ of an object. OR The net (total) work done ✓is equal to the change in kinetic energy of the object. ✓		(2)
	4.3.2		✓ for both components of weight ✓	(3)
	4.3.3	OPTION 1 W_net = ∆ EK ✓ any one W_f + W_g// = ¹/₂m(v²_f – v²_i) f ∙ ∆x ∙ cosθ + mgsin 60 ∙ ∆x ∙ cosθ = ¹/₂× 4(3² – 7,14²) ✓ f × 2 ✓ × -1 + 4 × 9,8 sin 60 ✓ × 2 × 1 = -83,9592 cos 60° cos 60° -4f + 135,7927833 = -83,9592 f = 54,94 N ✓
		OPTION 2 W_nc = ∆ E_p + ∆ E_K W_f = mgh_f -mgh_i + ¹/₂mv²_f –¹/₂m v²_i ✓ any one f ∙ cos θ ∙ ∆x = mg (h_f –h_i) + ¹/₂m (v²_f – v²_i) f ∙ cos 0 × 4 ✓ = 4 × 9,8 (0 – 4 sin 60°)✓ + ¹/₂× 4 (3² – 7,14²)✓ f = 54,94 N ✓		(5)
4.4	Remains the same ✓			(1)
				[17]

QUESTION 5
5.1	The total linear momentum of an isolated system remains constant (is conserved) ✓✓ OR Total linear momentum before a collision equals total linear momentum after a collision in an isolated system. ✓✓			(2)
5.2	5.2.1	OPTION 1 ∆y = v _i ∆t + ¹/₂a ∆t² ✓ 0,32 = v _i × 0,33 + 0 ✓ Vi = 0,97 m•s^-1 ✓	OPTION 2 ∆y = vi + vf ∆t² ✓ 2 0,32 = ^2v/₂ × 0,33 ✓ V = 0,97 m•s^-1 ✓	(3)
	5.2.2	Consider LEFT as positive ∑p_i = ∑p_f(m_c + m_m)v_i = m_cv_cf + m_mv_mf ✓ 0 ✓ = 2 × -0,97 + 0,005 × v_mf ✓ v_fm = 392 m•s^-1 east/right/forward ✓		(4)
5.3	Impulse = F_net .∆t = ∆p = m(v_f –v_i) ✓ any one = 2 (-0,4 – 0,97) ✓ = - 2,74 N•s = 2,97 N•s ✓			(3)
				[12]

QUESTION 6

6.1	Doppler effect ✓		(1)
6.2	The number of waves reaching the detector per unit ✓ time decreases ✓. OR As the ambulance is moving away from the scene /detector, the wavelengths become longer resulting in less waves reaching the detector ✓ per unit time ✓ hence the frequency decreases.		(2)
6.3	fL = v ± vL fs ✓ v ± vs any one fL = v fs v+vs 90% × 890 ✓ = 340✓ × 890 ✓ 340+vs 801 = 340 × 890 340+vs 340 +vs = 340 × 890 801 vs = 37,78 m•s^-1 ✓		(5)
6.4	Doppler flow meter is used to determine whether arteries are clogged / narrowed ✓ OR to determine the rate of flow of blood ✓		(1)
6.5	6.5.1	The shift is to a longer wavelength, lower frequency, as the star is moving away from the Earth. ✓	(1)
	6.5.2	A greater shift, therefore it shows that the distant star is moving away at a greater speed than a nearby star. ✓✓	(2)
			[12]

QUESTION 7

7.1	The electrostatics force between the two charges is directly proportional the product of the two charges and inversely proportional to the square of the distance between them. ✓✓				(2)
7.2	F_j= kQ_JQ_L r² F_J =(9 × 10⁹)(3 × 10⁻⁶)(2 × 10⁻⁶) (0,2)² FJ₌ 1,35 N right ✓				(4)
7.3	7.3.1	Q_L = -3 × 10^-6 ✓			(1)
	7.3.2	OPTION 1 n_ē = Qf - Qi qē n_ē = −a ×10⁻⁶−2×10⁻⁶ 1,6×10⁻¹⁹ ✓ n_ē = 3,125 × 1013✓	OPTION 2 n_ē = Qf - Qi qē n_ē =−3 × 10⁻⁶ + 8 × 10⁻⁶ 1,6 × 10⁻¹⁹ ✓ n_ē = 3,125 × 10¹³✓		(3)
	7.3.3		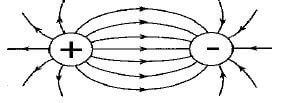		(3)
		Criteria for sketch /		Marks
		Correct shape		✓
		Correct direction		✓
		Field lines not crossing each other		✓
7.4	OPTION 1 E_J = KQ_J✓ = 9 × 10⁹ × 3 × 10⁻⁶= 1 875 000 N•C^-1 West/Left r² (0,12)² ✓ EM = KQ_M = 9 × 10⁹ × 3 × 10⁻⁶= 4 218 750 N•C^-1 West/Left r² (0,08)² ✓ Left positive : E_NET = E_J + E_M✓ E_NET = (+1 875 000) + (+4 218 750) ✓(both substitutions) E_NET= 6 093 750 N•C^-1 West/Left ✓				(6)
	OPTION 2 E_net = E_J + E_M ✓ = KQ_J + KQ_M ✓ r² r² ✓ one of the two/ below = (9×10⁹)× (3×10⁻⁶) + (9×10⁹)× (3×10⁻⁶) (0,12)² ✓ (0,08)² = 6 093 750 N•C^-1 West/Left ✓
					[19]

QUESTION 8

8.1	8.1.1	V max ✓ OR/OF maximum voltage ✓	(1)
	8.1.2	P_average = V_rms. I_rms ✓ (Pgem = V_wgk . I_wgk ✓) = (94,3) . ( 3 × 94,3 ) = 133,39 W ✓ (√2) ✓ (100 √2) ✓	(4)
	8.1.3	Replacing the slip rings with a split-ring commutator ✓✓	(2)
8.2	8.2.1	P =(V_rms)² R✓ (P = V_wgk²/R ✓) 56✓ =90² R R = 144,64 Ω ✓	(4)
	8.2.2	Too bright ✓ The power of the generator is greater than the power of the light bulb. ✓ OR The power of the light bulb is smaller than the power of the generator. ✓	(2)
			[13]

QUESTION 9

9.1	Emf / Emk ✓			(1)
9.2	9.2.1	OPTION 1 V_lost =Ir ✓ 0,9 ✓= 4,5 × r ✓ r = 0,2 Ω ✓	OPTION 2 Emf (Emk) = I (R + r) ✓ 22,14 =V_ext + Ir 22,14✓ = 21,24 + 4,5 × r ✓ r = 0,2 Ω ✓	(4)
9.2	9.2.2	OPTION 1 V4Ω = IR4Ω = 4,5 × 4 ✓ = 18 V Ve_xt = V_p + V4_Ω ✓ 21,24 = Vp + 18 ✓ V_p = 3,24 V ✓ R_eff = 3,24 4,5✓ R_eff = 0,72 Ω ✓	OPTION 2 V_4Ω = IR_4Ω = 4,5 × 4 ✓ = 18 V Vex_t = V_p + V_4Ω 21,24 = V_p + 18 ✓ V_p = 3,24 V ✓ I_4Ω = V = 3,24 = 0,81 A R 4 I_3Ω = V = 3,24 = 1,08 A R 3 I_R1 = I_P − (I_4Ω + I_3Ω ) I_R1 = 4,5 – (0,81 + 1,08) = 2,61 A ✓ R₁ = 3,24 = 1,24 Ω ✓ 2,61 1 = 1 + 1 + 1 = 1,39 R_eff 4 3 1,24 :. R_eff = 0,72 Ω ✓	(6)
9.3	Temperature ✓			(1)
9.5	Increase ✓			(1)
				[13]

QUESTION 10

10.1	Work function of a metal is a minimum energy needed by a metal in order to release/ejects electrons from its surface. ✓✓		(2)
10.2	W₀ = hf₀ ✓ = (6,63 × 10^-34) × (1,4 × 10¹⁵) ✓ = 9,28 × 10^-19J ✓		(3)
10.3	10.3.1	Remains the same ✓	(1)
10.3	10.3.2	Increase ✓	(1)
10.4	E_k =¹/₂mv² ✓ 0,7 × 10^-18 = ¹/₂× 9,11 × 10⁻³¹ × v²✓ v = √0,7 × 10⁻¹⁸ × 2 9,11 ×10−31 v = 1,24 × 10⁶ m•s^-1 ✓		(3)
			[10]
			TOTAL: 150

Last modified on Monday, 26 July 2021 07:33

Published in 2017 September Grade 12 Trial Examinations